1. Introduction

The first non-relativistic attempts to find the self-effects of radiation of the charged particle on its motion were made in the 19th century by Lorentz ^1,2 and few years later by Planck ^3-5 and by Abraham ^6,7. A relativistic proposal was made by Dirac ⁸ in 1938 such that its nonrelativistic limit coincides with the Abraham-Lorentz equation. Nevertheless, the Lorentz-Dirac equation ⁸ predicts some unphysical results such as the runaway solutions and the preaccelerations. As a consequence, many different proposals appeared in literature trying to avoid such inconsistencies ^9,10. The most famous approaches were made by Eliezer ¹¹, Wheeler and Feynman ^12,13, Caldirola ¹⁴, Mo and Papas ¹⁵, Landau and Lifshitz ¹⁶ and Yaghjian ¹⁷. However, in the past few years, the Landau-Lifshitz equation ¹⁶ and the Eliezer equation ¹¹ have been considered as the best equations for describing the motion of a charged point particle within Classical Electrodynamics. This is based on many distinct reasons. First, the mathematical analysis made by Spohn ^18,19 shows that in a perturbation theory the Landau-Lifshitz equation is obtained if the Lorentz-Dirac equation is restricted to its critical surface. Therefore, the approximations made obtaining the Lorentz-Dirac equation are of the same order of magnitude as those in the deduction of the the Landau-Lifshitz equation. Moreover, Rohrlich ^20-22 supports the Landau-Lifshitz equation by showing that the physical inconveniences of the Lorentz-Dirac equation disappear when the Landau-Lifshitz equation is considered due to the second order differential character of the equation. Second, a Landau-Lifshitz-like equation ²³ in General Relativity has been proposed supporting the validity of the Landau-Lifshitz equation in Special Relativity. Third, considering the point nature of a charge as a limit, which permits avoiding the black hole behavior of a point particle ²⁴, a structure must be assigned to the charge. In this order of ideas, considering a charged particle with structure, Ford and O’Connell ^25-27 by using quantum arguments and a Langevin equation, deduced an equation of motion for the non-relativistic case, known as the Ford equation which can be physically generalized to Special Relativity giving the Eliezer equation ²⁷. Fourth, within the Shen zone ²⁸ where a classical trajectory can be defined for a charged particle and quantum effects may be neglected, although the Landau-Lifshitz equation and the Eliezer equation are mathematical different, the solutions for both equations are similar and the differences cannot be detected ^29-31. Of course, the non-relativistic Landau-Lifshitz equation and the non-relativistic Eliezer equation (Ford equation) ²⁹ are equivalent. Fifth, and perhaps the most important argument to support the Landau-Lifshitz equation has been done by Krivitskiĭ et al³² by showing that the radiation reaction term represents an average radiation reaction force in Quantum Electrodynamics.

The Landau-Lifshitz and the Eliezer equations predict the vanishing of the damping force when a constant electric field is applied to a charged particle ³³; that is, the motion of the particle corresponds to the Lorentz equation of motion in such a case. This apparent paradox is explained by other authors by noticing that the radiation exits at the infinite; that is, the energy radiated to infinite is taken from the attached fields (The Scott term or the acceleration energy) and consequently even if the total radiation reaction term in the equation of motion vanishes, the radiation to infinite (the irreversible emission of radiation) exists ^34-37. Moreover, by using similar arguments, DeWitt and Brehme explain this phenomenon in his generalization to General Relativity of the damping term ^38,39. This explanation is not accepted by many authors ^40,37 and they have reached the conclusion that the rest mass of the charge is not conserved. Moreover, Sorkin ⁴¹ says that this phenomenon raises a paradox which we will call the constant electric field paradox. Recently, Hammond ^42-47 has proposed a new method which avoids this paradox and practically coincides with the solutions of the Landau-Lifshitz and Eliezer equations in many cases. Indeed, if we make a comparison of the solutions of both, Landau-Lifshitz equation and Hammond equation, for the constant magnetic field and low electromagnetic pulses we will notice that within the approximations made for the levels of energy where the damping force is important, the results are similar. However, Hammond claims that for high intense electromagnetic pulses the differences between the motions predicted by the Eliezer equation (denoted sometimes as the relativistic Ford equation), the Landau-Lifshitz equation and the Hammond equation are important. Such differences appear within the Shen’s zone ²⁸ where quantum effects are not important and an equation of motion is meaningful for a physical description. Moreover, Hammond argues that the results obtained by using Ford or Landau-Lifshitz equations do not accomplish a balance of energy ⁴⁶. In counterpart, these last equations are founded in different expressions for the radiated energy at the infinite; that is: the Larmor formula does not represent the radiation power at the infinite in these theories ^47,35. All these points of view make the study of Hammond proposal more interesting.

On the other hand, if we study the work done by Dirac ⁸, we will notice the necessity of considering a plus sign in the term of the damping force corresponding to the irreversible emission of radiation in order the total damping term is orthogonal to the 4−velocity and also that the motion of the electron in the hydrogen atom should make a spiralling inwards and ultimately falling into the nucleus (page 155 after Eq. (24) and page 157 after Eq. (30) of Dirac’s paper ⁸). The interesting fact consists in noticing that in Hammond theory the damping force contains a contrary sign. Therefore, an analysis of the central field, in particular for the attractive Coulomb case, is necessary in order to certify that in such situation the Hammond equation predicts a falling of the electron into the nucleus of an hydrogen atom with a rate of decay of energy consistent with the Larmor formula. Although as mentioned by Hammond ^45-47, the damping force is important for high energy and relativistic situations in particular for high intensity pulses, we will analyze the Coulomb case from a non-relativistic point of view since the purpose of the article just consists of noticing that Hammond equation predicts the falling of the charge into the center of the central field. If such a result is proved, the Hammond theory will be strongly supported as long as the degree of energy loss is acceptable and comparable to the Larmor formula. It has to be noted that Hammond already gave a simple example showing the falling of a charge in a special central field; for example in Hammond’s paper in 2010 ⁴³, it is stated that “To show this makes sense, we consider yet another simple problem: a charged mass rotating in a circle of radius R, confined by a circular collar that provides the inward normal force. We assume it has an initial velocity υ₀ and initial angular velocity w. Once again, to order τ_o, the analysis is elementary and we find that f = mτ_o w ³ R and υ = υ₀ − mτ_o w³ Rt. This yields for example, the change in kinetic energy ∆E_K per period T is ∆E_K /T = mτ_o w² R⁴ (in Hammond equation f is accompanied by minus sign). The right hand side is exactly what the Larmor formula predicts”. However, the inward force proportional to 1/r² has not been analyzed and it is necessary to prove the falling of the charge into the center of the central field for such physical important case.

In the analysis of the non-relativistic motion of a charged particle including the radiation reaction damping force it is necessary to consider a term due to a relativistic correction which is larger than the radiation reaction force in a central field ⁴⁸. Therefore, in Sec. 2, the solution of the attractive Coulomb central field will be briefly shown for the modified Ford equation ⁴⁸. The falling of an electron to the nucleus will be shown. In Sec. 3, the Hammond theory of radiation reaction force will be explained and a short description of its success will be exposed. In Sec. 4, by applying the Hammond model, the attractive Coulomb central field case for a non relativistic motion will be solved to see if the falling of the particle is predicted. The term due to a relativistic correction will be considered. In Sec. 5, in concluding remarks, the applicability of Hammond proposal is described for experiments particularly in Plasma Physics and its generalization to General Relativity is analyzed.

2. Corrected Ford Equation for a Central Field

In the last decade of the 20th century, by using quantum arguments and a Langevin equation, Ford and O’Connell ^25-27 deduced a non-relativistic equation representing the nonrelativistic limit of the Eliezer equation ²⁹. Without considering the paradox of the vanishing of the radiation reaction force in the case of a constant electric force, this equation does not present incovenient physical solutions. In this model, it has to be remembered that the Larmor formula is substituted by another expression for the radiation power at the infinite ²⁷. Nevertheless, this equation neglects some terms that come from Special Relativity which are bigger than the radiation reaction terms. Before analyzing the corrections that have to be made to the Ford equation ⁴⁸, let us describe the method that can be used to solve such an equation in the attractive Coulomb case.

2.1. Ford equation for an attractive Coulomb electric field

The Ford equation is written as

ma→=F→+τoF→, (1)

where the dot “·” represents the derivative with respect to the time, τ_o = 2q² /3mc³ is the characteristic time of the charge q (in cgs units) and F→ is the applied force. Let us consider a negative point charge (−q) which is subject to the electric Coulomb force F→ = q∇ϕ with the potential ϕ = q/r due to a positive electric charge q (the attractive case). The Ford equation can be written as

ddtmr-τqq∂ϕ∂r=l2r3+q∂ϕ∂r , (2)

being l→=mr→×υ→ the angular momentum. Defining U = −(qϕ/m) = K/mr (K < 0 for the attractive case with K = −kq²; this notation is used to follow Rajeev’s article⁴⁹), U_r = ∂U/∂r and l→=r→×υ→=l→/m. Wearrive to

l˙=-τorUrl    and  Ur=-Kmr2 , (3)

and consequently,

ddtr˙+τqUr=l2r3-Ur . (4)

Defining y as

y=l2 , (5)

and after some algebra ⁴⁹, one obtains

d3ydθ3+dydθ+2K2τqy=0 , (6)

where θ represents the polar angle and the orbit is given by

1r(θ)=1Kτqdydθ . (7)

Taking the natural units for this equations (|K| = τ_q = 1), we arrive to

d3ydθ3+dydθ+2y=0   and   1r(θ)=dydθ . (8)

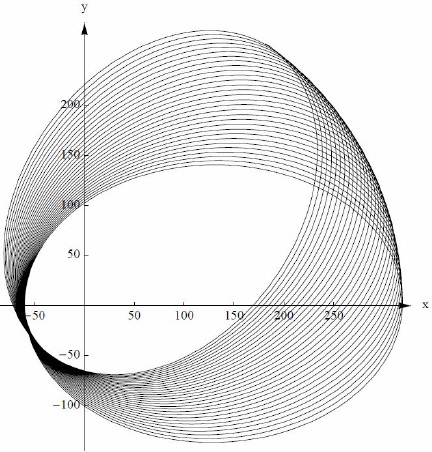
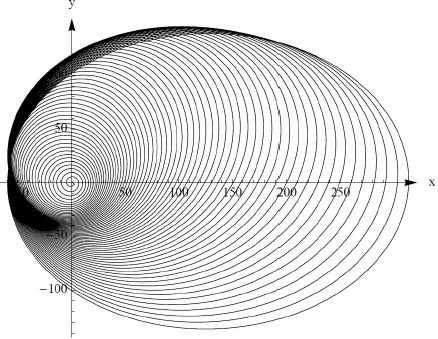
This equation can be numerically solved and gives a very congruent result about the falling of the charge into the center of the Coulomb electric field (see Rajeev ⁴⁹ and Ares de Parga et al⁴⁸, see Figs. (1) and (2)). It must be noted that there is no precession in the charge trajectory because the major axis of the decaying elliptical orbit does not change within its orbital plane.

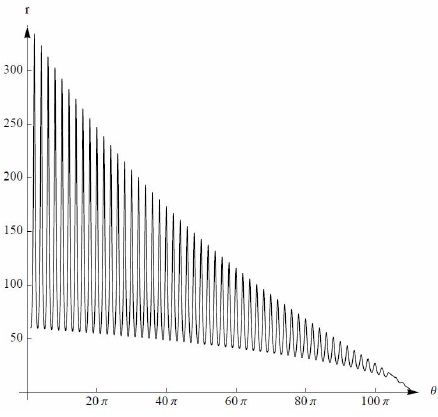
Figure 1 In this figure, we show r vs θ by using Ford equation for a charge in an attractive Coulomb field without considering the relativistic correction. 

Figure 2 The charge trajectory by using Ford equation for an attractive Coulomb field without considering the relativistic correction. 

2.2. Modified Ford equation for the attractive Coulomb electric field

Without considering the constant electric field paradox ^37,40-42,46 explained in the introduction, as we mentioned before for many authors ^{25,26,18-22,35} the Ford equation represents the best choice to describe the motion of a charged particle in the non-relativistic case. However, as we will see below, in the Ford equation, a term (−k² q⁴ /c² mr³) due to a relativistic correction must be added since its magnitude is larger than the radiation reaction damping force τoF→. Let us make a summary of why this term must be kept. First, consider just the Lorentz force for the Coulomb field, the relativistic equation for the r component in polar coordinates is

lr2ddθlmr2drdθ-l2mr3+k2q4mc21r3=-kq2Emc21r2 , (9)

where l = mr² dθ/dτ with τ the proper time and E represents the total energy of the particle. The term k² q⁴ /mc² r³ can be neglected in the non-relativistic case leading to closed orbits. However, if a radiation reaction term is added τoF→ to the Lorentz equation in the non relativistic case, the term (k² q ⁴ /mc² r³) must be kept because it is bigger than the radiation reaction term. Indeed, the modified Ford equation turns out to be

mr→¨=-kq2r2r^-k2q4mc2r3r^+τqkq2c2mr3r˙r^-τqkq2c2mr2θ˙θ^ . (10)

This is because if we compare the radiation reaction force with the k² q⁴ /mc² r³ term, we notice that the last one is larger; that is: (taking k = 1 in cgs units)

τqq2r˙r3q4c2mr3=τqmc2r˙q2=2r˙3c≪1 ,orτqq2θ˙r2q4c2mr3=τqmc2rθ˙q2=2rθ˙3c≪1 . (11)

Therefore, by looking to the central field case in Special Relativity, Eq. (9), it is obvious that an effective angular momentum due to a relativistic correction has to be incorporated in the equation of motion in the non-relativistic case ⁴⁸. This means that even if in the Ford equation the terms proportional to υ² /c² are neglected, not all the others terms which are proportional to 1/c² have to be neglected. Indeed, in order to heuristically recover the precession coming from Special Relativity, an effective force,

F→eff=-kq2r2r^-k2q4c2mr3r^ , (12)

must be considered in the equation of motion as is described in Eq. (10) and it corresponds to interchange l² by L²; that is:

ddtr˙+τqUr=L2r3-Ur , (13)

where

L2=l2-K2c2 , (14)

being,

l˙=-τqrUrl,   and   Ur=-Kmr2 . (15)

we arrive to

r¨+2τqKr˙r3=l2-K2/c2r3+Kr2 . (16)

Therefore, by putting

y=l2 , (17)

we obtain

d3ydθ3+dydθ-1c2ydydθ+2k2τqy=0 , (18)

with

1rθ=1Kτqdydθ . (19)

Applying the same numerical method and equal natural units as in the case of the usual Ford equation, we can see the difference between the Rajeev result ⁴⁹ and the result obtained from the modified Ford equation in Figs. (1), (2), (3) and (4). An interesting aspect should be highlighted. If we solve the equation of motion without considering the radiation reaction term but keeping the term (k² q⁴ /mc ² r³), a precession appears in the charge trajectory since the major axis of the elliptical orbit precesses within its orbital plane. Such precession causes non-closed orbits similar to rosettes as it is shown in Figs. (5) and (6). However, when the radiation reaction term is taken into account, the precession is reduced as can be seen in Figs. (3) and (4). Nevertheless, a slight change in the major axis can be observed in the trajectory (see Fig. (4)).

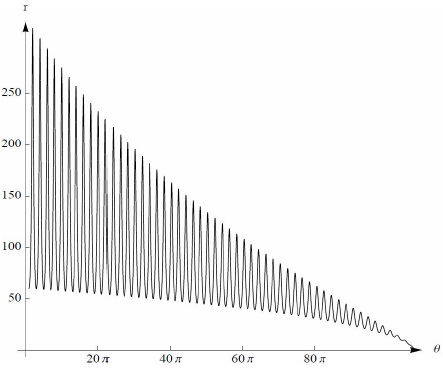
Figure 3 In this figure, we show r vs θ by using Ford equation for a charge in an attractive Coulomb field by considering the relativistic correction (the modified Ford equation). 

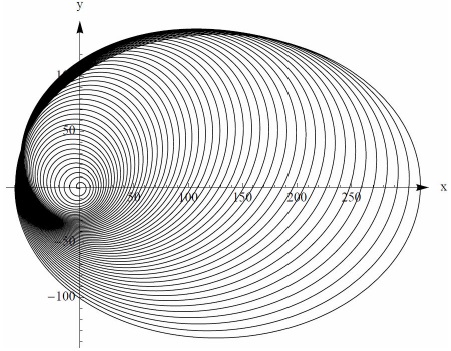
Figure 4 The charge trajectory by using Ford equation for an attractive Coulomb field by considering the relativistic correction (the modified Ford equation). 

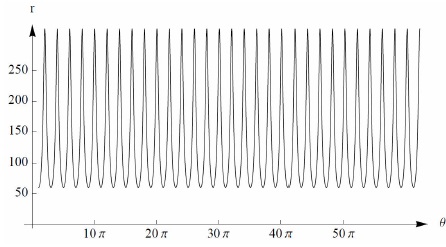
Figure 5 In this figure, we show r vs θ by using Lorentz equation for a charge in an attractive Coulomb field by considering the relativistic correction. 

Figure 6 The charge trajectory by using Lorentz equation for an attractive Coulomb field by considering the relativistic correction. The non-closed orbit effect is shown. 

3. Hammond Relativistic Proposal

In order to avoid the constant electric field paradox ^37,4042,33 and as Hammond mentioned ⁴⁶ “to obviate all of the problems outlined above by assuming energy is conserved without assuming some of it in wondrously stored in an immeasurable field”, based on the fact that radiation represents an irreversible process, Hammond ^42-47 proposed that an equation of motion for a charged particle which includes a self-force due to its radiation itself cannot be expressed in an usual form; that is: the method to obtain the self-force is described as follows: consider an equation of motion with a radiation reaction force f^μ such that

with

where ϕ represents a potential which has to be deduced in each case. It has to be noted that due to the form of f^μ,

Consequently, the radiation reaction force is orthogonal to υ_μ and Eq. (20) satisfies the balance of energy. Moreover, in order to make this system compatible with Physics, a constraint is required; that is

with P = −τ_o a_μ a^μ. Equations (20), (21) and (23) represent a complete system. Of course, this is not a covariant statement but it holds for a particular frame where we measure the field. This method has been used to solve the magnetic and electric constant field cases, pulses etc ^42-47. The results are quite physical and they support the Hammond proposal as an interesting theory to substitute all the other equations which try to include the self-effects due to the radiation reaction force of the charge. It has to be noted that the equation does not possess any physical inconveniences. In the nonrelativistic case, the equation may be written as

where F→eff represents the external force plus the relativistic correction that has to be deduced and added for each external force as in the case of the central force in Eq. (12) and f⃗ the radiation reaction force. The constraint is

Therefore, by using Eqs. (24) and (25), a complete description is obtained which permits to propose the value of the radiation reaction force f→ in each case.

4. Non Relativistic Modified Hammond Proposal for the Attractive Coulomb Case

In the central field case, the radiation reaction force can be proposed as

f→=τoma2υ→υ2 . (26)

With this expression of the radiation reaction force, we are assuring the concordance with the Larmor formula, we arrive to,

mr→¨=-kq2r2r^-K2c2mr3r^-τoma2r→˙υ2 . (27)

It has to be noticed that the force is antiparallel to the motion of the charge as Hammond requires. Since we are interested in describing the motion of the charge and due to the fact that τ_o is very small, for obtaining a numerical solution we can substitute the acceleration in Eq. (26) by

a=kq2mr2 . (28)

Then, we have

mr→¨=-kq2r2r^-K2c2mr3r^-τomk2q4r4r→˙υ2 . (29)

Now, knowing that

r→˙=r˙r^+rθ˙θ^ , (30)

we obtain

mr→¨=-kq2r2r^-k2q4c2mr3r^-τomk2q4r4r˙r^+rθ˙θ^r˙2+r2θ˙2 . (31)

Therefore,

mr¨-rθ˙2=-kq2r2-k2q4c2mr3-τomk2q4r4r˙r˙2+r2θ˙2, m2r˙θ˙+rθ¨=-τomk2q4r4rθ˙r˙2+r2θ˙2 . (32)

These previous equations are very difficult to analytically solve and a numerical method must be used to make a comparison with the modified Ford equation. When the velocity vanishes, the radiation reaction force diverges and the equation of motion for this case may present some difficulties. Accordingly, we will just analyze cases where the velocity does not vanish. Figures (7) and (8) describe the solution of the Hammond proposal for the attractive Coulomb case without considering the precession correction due to the term (k²q⁴/mc ²r³). Figures (9) and (10) are the solution of the modified Hammond proposal for the attractive Coulomb case, Eq. (32).

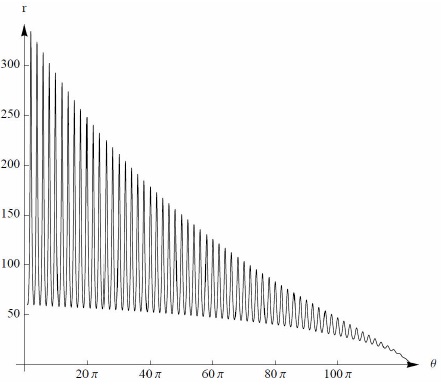
Figure 7 In this figure, we show r vs θ by using the Hammond proposal for a charge in an attractive Coulomb field without considering the relativistic correction. 

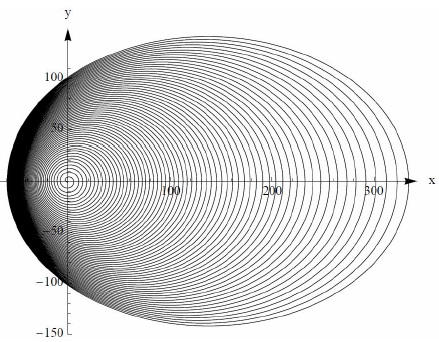
Figure 8 The charge trajectory by using the Hammond proposal for an attractive Coulomb field without considering the relativistic correction. 

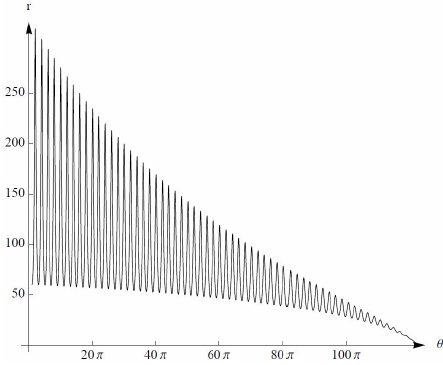
Figure 9 In this figure, we show r vs θ by using the Hammond proposal for a charge in an attractive Coulomb field considering the relativistic correction (the modified Hammond equation). 

Figure 10 The charge trajectory by using the Hammond proposal for an attractive Coulomb field and by considering the relativistic correction (the modified Hammond equation). 

5. Comparison Between Equations

The objective of this section consists of showing the differences between the Ford equation and the Hammond equation in the case of an attractive Coulomb field by considering the relativistic correction or not. Let us first consider the Ford equation and the Hammond equation without the relativistic case. At first glance, in Figs. (1), (2), (7) and (8), it is not trivial to notice the differences. However, if we make a superposition of Figs. (1) and (7), we can notice that a difference exists in Fig. (11). Indeed, a rapid analysis shows that the Hammond orbit falls into the center of the attractive Coulomb center slower than the Ford orbit.

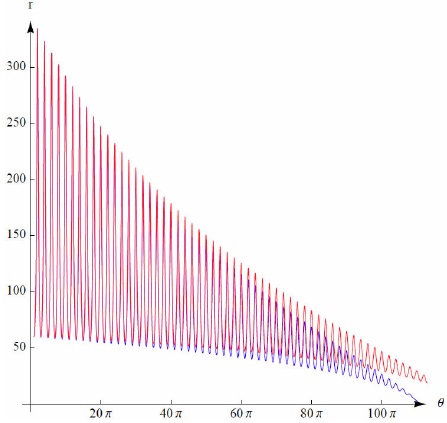
Figure 11 Superposition of Figs. (1) and (7); the blue and red lines correspond to Ford and Hammond lines, respectively. 

On the other hand, looking at Fig. (12), which describes the orbits predicted by Ford equation and Hammond equation by using a superposition and by making a zoom, we can observe that as in the previous figure, the Hammond orbit falls into the center slower but it can be noticed that the eccentricity of the Hammond orbit decreases faster than the Ford orbit. This means that Hammond predicts a falling to the center but with a bigger tendency to convert into a circle with respect to the Ford equation.

Figure 12 Superposition and zoom of Figs. (2) and (8); the blue and red orbits correspond to the Ford and Hammond orbits, respectively. 

When we analyze the modified Ford orbit and the modified Hammond orbit we can look at Figs. (13) and (14). Although in both orbits a precession appears, the differences are similar to those obtained in the usual equations (not modified).

Figure 13 Superposition of Figs. (3) and (9); the blue and red lines correspond to the modified Ford and modified Hammond lines, respectively. 

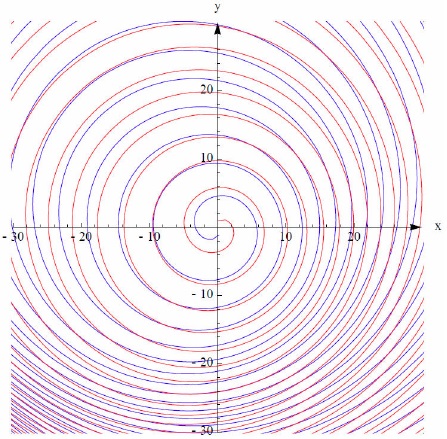
Figure 14 Superposition and zoom Figs. (4) and (10); the blue and red orbits correspond to the modified Ford and modified Hammond orbits, respectively. 

Notwithstanding some differences can be detected between the solutions of the equations the differences are negligible. It has to be remembered that one of the purposes of this paper was to demonstrate that the Hammond equation satisfies the Dirac constraint of obtaining an equation of motion that should cause the electron in the hydrogen atom to spiral inward and finally to fall into the nucleus.

Finally, if a comparison between the solutions of the modified Hammond and the modified Ford equations is made, the similarities are obvious. Therefore, it can be concluded that the Hammond proposal is a consistent model which can be considered as an acceptable theory to describe the radiation reaction force. The differences between the Hammond equation with the Landau-Lifshitz and the EliezerFord-O’Connell equations are fundamentally two: the electric constant paradox in the Hammond proposal does not appear since the radiation reaction force does not vanish ^42-47; for high intensity pulses, the gain of energy by a charge is larger in the Hammond theory ⁴⁶.

6. Concluding Remarks

Some concluding remarks will be presented:

i)It was demonstrated that the obtained orbits from the Hammond equations with or without relativistic correction fall into the center of the attractive Coulomb field (see Figs. (8) and (10)). Then, the objective of the present work was achieved.

ii)In the solution of the modified Hammond equation a precession is observed in the decaying orbit as in the solution of the modified Ford equation. Although there is a slight difference between the results obtained from Ford and Hammond equations since in the case of Hammond the decay is slightly smaller and the eccentricity of the orbits tends more rapidly to zero, the differences are negligible. Moreover, Quantum mechanics is predominant for energies that generate orbits and the above study may loss sense ²⁸. In fact, we are only analyzing the feasibility of Hammond theory.

iii)In fact, the differences between the equations can be detected in very special physical conditions. Actually, Di Piazza et al⁵⁰ described the experiments that have been performed to explore the classical regime concerning the interaction of free electrons with intense laser beams. Hammond has also proposed an experiment ⁴⁶ which includes the use of recently reached ultrahigh laser intensities (10²² Wcm^{−2 (51}), in order to distinguish the differences between the predicted results by the Landau-Lifshitz equation, by the Eliezer-Ford-O’Connell equation and by his own proposal. In fact in the case of an applied electromagnetic pulse, unlike the others equations, the solution of the Hammond equation gives a gain of kinetic energy. In the seventies of the past century, Shen ²⁸ described a zone in a diagram fields vs energy, where quantum effects are negligible for a constant magnetic field. For an electric field, following Shen, the classical radiation theory is an adequate approximation of the quantum radiation theory when

If laser intensity I is around of 10²² Wcm⁻², the corresponding average electric energy is

Consequently, the kinetic energy of the electrons must be such that

It can be accomplished for relativistic velocities but not for the ultrarelativistic case. The Hammond experiment must be constrained to Eq. (35).

iv) Other experiments have been proposed, for instance, the influence of radiation reaction can be analyzed on multiphoton Thomson scattering by an electron colliding head-on with a strong laser beam ⁵². Di Pizza et al ⁵² affirmed “Radiation Reaction can be experimentally investigated with currently available laser systems and the underlying widely discussed theoretical equations become testable for the first time”. Although this experiment was designed to test the validity of Landau-Lifshitz equation, it can be improved to compare the results with the Hammond equation. The Hammond equation could be analyzed in other experiments as the ones proposed by Harvey et al⁵³ (phase factor), by Zidhov ⁵⁴et al (the radiation reaction damping may restrain the maximal energy of relativistic electrons in ultraintenselaser-produced plasmas), by Tamburini et al⁵⁵ (on radiation pressure acceleration of plasma slabs) or by Schlege et al⁵⁶ (Laser ponderomotive force at superhigh intensities, overdense plasmas).

v) In Plasma Physics by linearizing the relativistic Vlasov equation and including the Landau-Lifshitz radiation reaction force, Hakim and Mangeney ⁵⁷ succeeded in obtaining the dispersion relations which include terms proportional to τ_o. A strong damping for high frequencies (τ_ow²/2) appears. Such damping effect can be compared with the one obtained by taking into account the Hammond equation in the relativistic Vlasov equation.

vi) In General Relativity, a consistent theory where the work done by the self-force matches the energy radiated away by the particle implies the existence of a tail term which includes all the history of the particle ^38,23. A General Relativity theory of the motion of the charges within the philosophy of Hammond has not been achieved and it represents an important goal to be attained in order to close the Hammond proposal. Moreover, a low energy model has been developed by DeWitt and DeWitt ⁵⁸ which can be used as a model for generating a Hammond equation in General Relativity at low energies.