1. Introduction

Recently the physics of particles and fields in curved surfaces in Euclidean space has become subject of interest, because many phenomena are reduced to one of them. For example, it is known that a liquid crystal on curved surfaces, look for smectic phases as parallel curved surface¹. We also know, that some electronic properties of certain two-dimensional materials, are explained modeling particles, living in the surface, satisfying the Schrödinger or the Dirac equation². Topological defects on surfaces³, relativistic particle dynamics⁴, and diffusion on surfaces⁵ are also some examples of how the interaction of particles and fields with surfaces through geometry, can be used to model several natural phenomena. Although from a mathematical point of view, variational problems related to curves and surfaces, has been addressed⁶, they have recently attracted attention, because has been possible to interpret the Euler-Lagrange equations in physical terms, as a balance of internal forces and moments, see e.g.⁷ and⁸.

Inspired by a recent formalism developed to investigate properties of elastic curves constrained on surfaces⁹, in this work we obtain the basic, but generic, geometric elements of particles constrained on surfaces. Although it is well known the classical physics of particles, usually specific cases of particles moving on surfaces are solved. Here we present its description in terms of the geometric information of the surface, either intrinsic through the gaussian curvature or extrinsic information, encapsulated in the second fundamental form.

We obtain, aside to the equations of motion, the constraining force on the particle. The corresponding equations of small perturbations are also obtained, showing that they includes, even with no external forces, extrinsic information. Some general results if the surface has axial symmetry and the gravitational field taken into account are showed.

2. Lagrangian Classical Mechanics

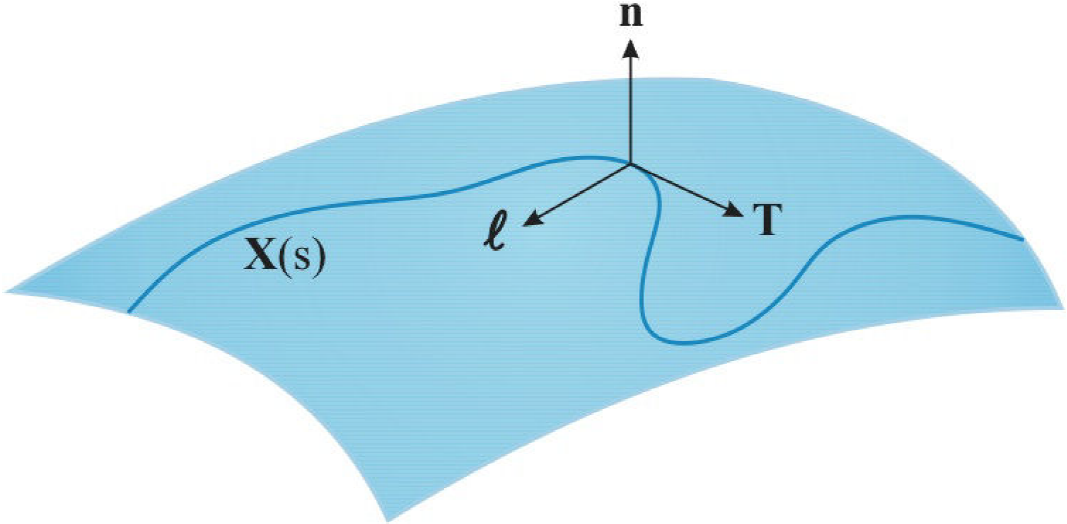
Let us consider the action of a free particle, constrained on a surface through the vector Lagrange multiplier λ,

Here x(t)=(x1(t),x2(t),x3(t))∈R3, the dot stands for the derivative with respect to the time t, and m is the particle’s mass. The equation of the surface, parametrized by local coordinates ξa, a={1, 2}, can be written as x=X(ξa). By taking infinitesimal deformations x→x+δx we have

After integration by parts in the first term, the corresponding Euler-Lagrange equations are given by

Moreover, variation respect to the local variables, ξa→ξa+δξa, gives

where ea=∂aX, are tangent vectors to the surface. Therefore, the Lagrange multiplier λ=-λn, being n=e1×e2/||⋅||, the unit normal to the surface. Finally, under deformation of the Lagrange multiplier, δλ, we have that

and the equation for the constraint is obtained.

However, the Eq. (3), in not useful in this form. Instead, we write it in the Darboux frame {T,n,l=T×n}, where T is the unit tangent to the curve and l is orthogonal to the curve on the surface, see Fig. (1). This basis satisfies that T'=κnn+κgl, n'=-κnT+τgl, l'=-κgT-τgn, where prime stands for the derivative respect to arc length s¹⁰. These equations defines de normal curvature κn=T'⋅n, the geodesic curvature κg=T'⋅l in addition to the geodesic torsion τg=n'⋅l.

Figure 1. The Darboux frame. 

We also consider that the constraint does not depend on time, then the velocity of the particle is given by

where v(t)=ds/dt, ta=dξa/ds and s the arc length along the trajectory of the particle. The relation of time t, with the arc length of the curve is given by ds2=gab(dξa/dt)(dξb/dt)dt2, that involves the induced metric on the surface, gab=ea⋅eb. Then we can write v2(t)=gab(dξa/dt)(dξb/dt). In addition, using Eqs. (5) and (3), we see that x˙⋅x¨=0, and therefore the conservation of the energy, mv2(t)/2=E, follows.

The second derivative can be obtained from

In the first term we can get

in the second line, the second fundamental form of the surface Kab=-∂aeb⋅n, has been introduced, and the Christoffel symbols Γbca compatible with gab also appear; there is no projection along T, since it is a unit vector. Notice that we can identify κn=-Kabtatb and κgl=κgcec. Thus, we can write Eq. (3) in the form

where a(t)=dv/dt. Therefore, we can read that the particle moves along a geodesic path, κg=0, with a(t)=0, i.e. v(t)=v0, and feels a force, along the normal to the surface given by

We can write this force in terms of the principal curvatures κi, of the surface

where, as usual, θ is the angle between T and the principal vector v ₁.

If there is an external force F, acting on the particle, we can decompose the equation of motion in the local basis and we can write

such that, the corresponding equations to solve are then given by

and the energy is conserved, mv2(t)/2+U=E, with U the corresponding potential relative to the external force field. Notice that if we use the first and the third equations, then

i.e. the force λ is written in terms of geometric information of the surface and of course, in general, it depends on time t. Therefore, a condition such that no constraining force exists is given by Fn=mv2κn, or

This is a remarkable expression, which tell us that, in order that the constraining force vanishes, the ratio of non-tangential components of the external field must be equal to the ratio of the curvatures of the surface.

2. 2. Geodesic trajectories

On perturbed geodesic curves, the deformation on the constraining force, is given by

δλ=2mvτgΨ̇+mvτ̇gΨ. (28)

Without external forces it simplifies such that δλ=mv(2τgΨ̇+τ̇gΨ). The transversal perturbation satisfies the Raychadhury-like equation¹²

mΨ¨-(mv2(τg2+R/2)+(mv2κn-Fn)Kablalb)Ψ=0. (29)

Besides intrinsic information, projections of the second fundamental form appear in this equation. Even without external forces, extrinsic information are required,

Ψ¨-(v2(τg2+R/2)+v2κnKablalb)Ψ=0. (30)

A simple example is given by a particle falling on the unit sphere, starting from rest at the north pole, under the gravitational field. Since Fl=0 then, according to the first equation in Eq. (12), we have κg=0, and therefore the trajectory is a great circle. For a sphere of radius r it is known that Kab=gab/r, such that for a unit sphere we have Kab=gab and hence κn=-1, τg=0. Moreover, since R=K2-KabKab in this case R=2, and from the energy conservation we have v2=2g(1-cosθ), where θ is the angle between the normal vector n and the axis z. Due to the geodesic torsion vanishes we get δλ=0. On the normal direction, Fn=-mgcosθ, and then the transversal deformation follows the equation Ψ̈-gcosθΨ=0. In terms of the angle θ,

v2θ'2∇θΨθ+gθ'sinθ Ψθ-gcosθ Ψ=0. (31)

Nevertheless, we note that a first integral is, Ψ̇2/2-gcosθ Ψ2=C, and therefore

2g(1-cosθ)θ'2Ψθ2-2gcosθ Ψ2=C. (32)

2.2.1 Axisymmetric surfaces

If the surface has axial symmetry, we can parametrize it in the form

X(φ,l)=(ρ(l)cos⁡φ,ρ(l)sin⁡φ,z(l)). (33)

The infinitesimal element of distance is given by ds2=ρ2dφ2+(ρ'2+z'2)dl2, here prime indicates derivative respect to l. The tangent vectors e _a are

eφ=(-ρsin⁡φ,ρcos⁡φ,0),el=(ρ'cos⁡φ,ρ'sin⁡φ,z'). (34)

The unit normal is then

n=1ρ'2+z'2(z'cos⁡φ,z'sin⁡φ,-ρ'). (35)

The second fundamental form K _ab has components,

Kφφ=ρz'ρ'2+z'2

and

Kll=ρ'z″-ρ″z'ρ'2+z'2.

The principal curvatures are found to be

Kφφ=z'ρρ'2+z'2

and

Kll=Kllρ'2+z'2.

With these basic elements we can describe a particle restricted to lie along this surface. Because the symmetry under rotations about the z axis, Mz=M⋅k is conserved where M=mx × x˙, is the angular momenta and x˙=eφφ˙+ell˙. We find

Mz=mρ2φ̇. (36)

The normal curvature along the trajectory is given by

-κn=Kφφdφds2+Klldlds2. (37)

This equation can be written in terms of M _z . In the first term we can use Eq. (36), in the second one we can use the induced metric on the surface, to obtain a first integral of κg=0, in the form

12dlds2+Ueff=0, (38)

where

Ueff(Mz,l)=-12(ρ'2+z'2)1-Mz2m2v2ρ2. (39)

Then we can write the normal curvature of geodesics

-κn=Mz2m2v2z'ρ'2+z'21ρ3+ρ'z″-ρ″z'(ρ'2+z'2)3/2(1-Mz2m2v2ρ2), (40)

and therefore, the force λ in Eq. (9), is completely determined. If the gravitational field F=-mgk is taken into account, we can see that

Fn=mgρ'ρ'2+z'2,Fl=mgz'ρρ'2+z'2dφds. (41)

The velocity v(t), is determined by conservation of the energy

v2(t)=2mE-mgz(l(t)). (42)

An example is the catenoid, a minimal surface that we can parametrize through

X(φ,l)=(cosh⁡lcos⁡φ,cosh⁡lsin⁡φ,l), (43)

where l∈(-∞,∞), φ∈ 0, 2π. The normal curvature of geodesic curves is parametrized by M _z

-κn=sech2l(2Mz2sech2lm2v2-1). (44)

The curvature R=-2sech4l, and the second fundamental form has components Kφφ=1, and Kll=-1. If a particle moves on this surface under the gravitational field, then the projections of the force are Fn=mgtanhl, and Fl=gMz/v cosh2l. Because the symmetry, geodesics can be classified according the angular momentum M_z, see Fig. (2): If 0<Mz<1, geodesic crosses parallels along the catenoid; for Mz>1, the path is on one side of the catenoid; the equator corresponds to Mz=1 and Mz=0 is a meridian¹¹.

Figure 2. The several geodesic curves on the catenoid, classified according the angular momenta. 

An easy example is a particle falling along a meridian starting at l ₀, and v2=2g(l0-l). The constraining force λ is found to be

λ(l)=mg(tanhl-2(l0-l)sech2l). (45)

If the particle leaves the catenoid at l _s , then λ(ls)=0, implies that tanhls=2(l0-ls)sech2ls. If for instance l0=1, then ls∼0.61.

Being φ=const, for this path tφ=0 and tl=sechl. From the definition of l, we note that la=gϵabtb. We have ll=0 and lφ=sechl. Therefore the equation of the transversal deformation is given by

Ψ¨+gsech2ltanhlΨ=0. (46)

2.2.2 Particle on a surface in a curved space

If the particle moves onto a surface embedded into a curved space, with coordinates xμ and metric gμν(xα), we have the constrained functional as

L(xμ,ξa,λ)=m2∫v2dt+∫λμ(xμ(t)-Xμ(ξa))dt. (47)

The first term involves the background metric, v2=gμν(x)ẋμẋν, the second one the Lagrange multipliers λμ. It generalizes equation (1) to curved background. Under deformations δx we obtain as before, equation (3), but now in the form

md2xμdt2+Γναμdxνdtdxαdt=-λnμ, (48)

where the Christoffel symbols compatible with gμν appear. The projection on the Darboux frame follows the same lines as before. We then have

mv2(t)(κnnμ+κglμ+ΓναμTνTα)+ma(t)Tμ=-λnμ, (49)

where T=Tμeμ, is the tangent vector field, and eμ=∂μX, the tangent vectors to the surface. If the particle moves freely we have, as before, that it follows a geodesic curve along the surface, with a(t) = 0. The force λ is given by

λ=-mv2κn. (50)

In this case the second fundamental form Kab is defined through the covariant derivative Dμ, compatible with gμν: Da=eaμDμ, i.e. Kab=-g(Daeb,n). In addition we can see that the projection of the Christoffel symbols along the tangent vector is null

ΓαβνTαTβ=0. (51)

It is certainly interesting to know the effect of nontrivial background, that we will present in future work.

3. Summary and conclusions

In this paper we have presented the geometric elements in the description of particles on surfaces. Projection of the Newton second law along the normal to surface, gives the constraining force, that involves the normal curvature of the surface. Geodesic curvature (times v ²) gives the acceleration in the transversal direction to the movement. Classical perturbations of the path is equivalent to a field theory on curved surfaces. We show that from conservation of energy, a first order equation of the tangential deformation, is obtained. We also show that the equation of the transversal perturbation follows a Raychaudhuri-like equation that includes extrinsic information even if there is not external forces. If the particle moves onto a surface with axial symmetry, the results are parametrized in terms of the conserved projection of the angular momenta. The particular results in the case of geodesic curves are obtained. Using the formalism here presented, a problem to be addressed, is related to the motion of extended objects on surfaces, for elastic curves, the model must include the bending energy, quadratic in the curvature which has been extensively examined¹³.