Services on Demand
Journal
Article
Indicators
Related links
Share
EconoQuantum
On-line version ISSN 2007-9869Print version ISSN 1870-6622
EconoQuantum vol.10 n.1 Zapopan Jan./Jun. 2013
Artículos
Reaching the optimal growth: The role of labor market institutions
Coralia Azucena Quintero Rojas1
1 Universidad de Guanajuato, Departamento de Economía y Finanzas, DCEA. Agradezco los comentarios hechos por los dictaminadores anónimos que hicieron que el artículo se enriqueciera.
Recepción: 30/06/2011
Aceptación: 01/06/2012
Abstract
We develop a general equilibrium model of endogenous growth with trade unions and other labor market institutions. We show that it is possible to reach the optimal growth rate by compensating the distortions on the goods-sector due to the growth process with the distortions induced by the labor market rigidities.
Keywords: endogenous growth, optimal growth, labor market institutions.
JEL Clasification: J5, O3, O4.
Resumen
En este trabajo se desarrolla un modelo de crecimiento endógeno con sindicatos y otras instituciones propias del mercado laboral. En este contexto se demuestra que es posible alcanzar la tasa de crecimiento social compensando las distorsiones en el mercado de bienes con aquéllas inducidas por las instituciones laborales.
Introduction
In this paper, we extend the basic model of technological change of Aghion and Howitt (1992) to introduce trade unions and other labor market institutions such as labor taxes on labor income and unemployment benefits. This provides us with a straightforward context to analyze the optimality problems that derive from the basic market imperfections associated to technological innovation. As in the basic model, growth is primarily driven by a sequence of quality-improving innovations, each of which destroys the rents generated by previous innovations. This creative destruction in the economic growth process could lead either to insufficient or excessive economic growth. This is mainly explained by the distortions on the goods-sector induced by the monopolistic rents generated by R&D. However, we show that when the institutions and rigidities present in the labor market of many developed economies are acknowledged by the model, the optimal growth rate could be reached by compensating the goods-market distortions with those produced by the labor market rigidities. In our simple framework, the market power of incumbent firms is also related to the bargaining problem with labor, which makes the assumption of the existence of unions more natural.
The introduction of unions and other labor institutions in endogenous growth models has already been treated in the vast literature concerned with the impact of growth on employment and the other way around. For instance, also building on the Schumpeterian framework of Aghion and Howitt (1992), we can cite a few contributions: Lingens (2003) treats the impact of trade unions in a model with two kind of skills and shows that the bargain over the wage rate of low-skilled workers causes unemployment but the growth effect is ambiguous. Mortensen (2005) finds a negative effect of labor market policy variables on unemployment, but an ambiguous effect on growth.
Adjemian, Langot, and Quintero-Rojas (2010) analyze how the frictions in the labor market simultaneously affect the economic growth and the long run unemployment and show that increases in the labor costs or in the power of trade unions lead to higher unemployment and lower growth. On the other side, Palokangas (2004) supports the hypothesis of a positive growth impact of trade unions in a two-sector economy with R&D-driven growth in a high-tech sector and a stagnant low-tech sector.
All these contributions share an aspect: they feature Schumpeterian endogenous growth and analyze the impact of unionization, or a higher union bargaining power, and other labor market variables on growth and employment. Our paper takes distance from this and contributes to the current literature by analyzing optimality issues in the presence of a variety of labor-market related distortions.
The model
As in the basic model of Aghion and Howitt (1992, 1998): (i) Growth is generated by innovations that improve the quality of products and destroy the result of previous innovations by making them obsolete. (ii) Innovations are the result of random discoveries produced by research activity driven by profits. Additionally, we have the following hypothesis characterizing the labor market: (iii) There is a union representing the workers' interests that bargains over the wages. (iv) Employed workers pay a tax on their labor income whereas unemployed workers receive an unemployment compensation.
Basic Framework
The economy is populated by L identical individuals, with L constant over time. Preferences are represented by the welfare function

ρ < 0 is the subjective rate of time preference and Ct is the individual's consumption of the final good at time t. Since there is risk neutrality, the interest rate equals the discount parameter. Every individual offers one unit of labor per unit of time, at zero cost.
The final good, which is consumed, is produced by perfectly competitive firms that use the latest vintage of intermediate input xvt with the technology2
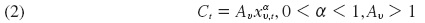
Av is the productivity of the intermediate product and is determined by the number of technical improvements realized up to date t. Innovations improve the quality of intermediate products: an innovation creates a new variety of intermediate product whose productivity is larger by a multiplicative factor q > 1 than the previous intermediate product

Final output sector is perfectly competitive, so that the profits of the final output producer are

from the profit maximization we deduce that the marginal productivity of intermediate product in this sector is equal to the price

At each time, individuals may be employed on the intermediate product sector, trying their hand at R&D or unemployed. The intermediate product sector uses a one-to-one technology, so that one unit of labor produces one unit of intermediate output. Let us denote xv the amount of labor devoted to the intermediate product sector, nv the quantity of labor in the research sector, and uv the quantity of unemployed labor. Then, we have the following labor constraint:

where the subindex υ stands for the current intermediate product variety.
The employed workers pay a tax τ on their labor income whereas the unemployed receive some unemployment benefits B, so that the government faces the following balanced-budget constraint:

Then, any change in the revenue caused by changes in taxes and subsidies is rebated to household through the lump-sum transfer T. The value of one innovation.
Technology improvements lead to good-specific public knowledge allowing to start improvement efforts upon the current vintage. Innovations arrive randomly at a Poisson rate hnv > 0, where h > 0 a parameter indicating the productivity of the research technology.3
Firms which innovate can monopolize the production of the new intermediate product. Consequently, innovations create three externalities:
1. The monopoly rent obtained by the innovator is generally smaller than the surplus induced by the innovation.
2. Every innovation increases A and then the productivity of future research, since knowledge is a non-rival good.
3. Previous goods are destroyed.
If the current vintage is υ, the value or expected payoff of next innovation, denoted by Vυ + 1 is defined as the net discounted value of an asset yielding πυ + 1 per period, until the arrival of next innovation, at the arrival rate hnυ + 1 That is:

where π are the instantaneous monopolistic profits earned by the successful innovator, given by:

Finally, the size of the R&D sector is deduced from the fact that individuals can choose between production or research activities, so that the following arbitrage condition holds:

That is, since labor can be freely allocated, the net value of one hour of work in the production sector (left hand side) must be equal to the expected value of one hour devoted to research (right hand side).4
Wage bargaining and labor demand
The wage rate is the solution to the bargaining problem between the monopolistic producer and the trade union representing the workers' interests. After the wage bargaining process, under the right to manage assumption, the firms determine their optimal labor demand taking as given the bargained labor cost per employee.
Substituting the expression for pυ + 1 from equation (5) into equation (9), and solving the maximization problem we get:
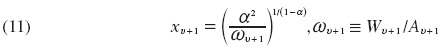
We model the bargaining process as a generalized Nash bargaining game, with union's relative bargaining power β. If agents don't agree, workers get the unemployment benefits and the monopolist makes zero profits. Given the bargained wages, the firm chooses the level of employment that maximizes her profit flow. That is,

with and  .
.
Equilibrium
Given r > 0, the equilibrium is defined as follows:
Wage rule: The normalized wage rate is given by the solution of the Nash game,5
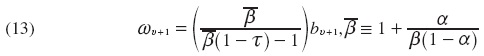
Labor demand: Given (13), the labor demand (11) becomes
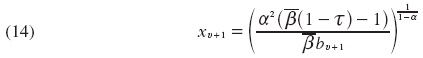
Labor allocated to research: The size of the R&D sector is determined by the free entry condition (10):

Unemployment: Substituting (14) and (19) into (6) gives the unemployment level:

Stationary Growth
The stationary solution, denoted by (w,x,n,u) satisfies equations (13) to (16):

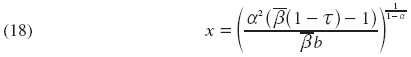

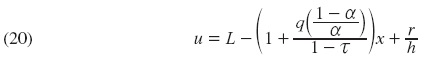
Finally, let us compute the average rate of growth. There are hndt innovations over every small interval of time. Every innovation increases output by q. Then,
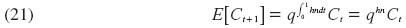
Taking natural logarithms and rearranging terms:
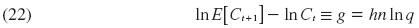
The optimal economic growth
The optimal growth rate g* is determined by the optimal level of research n* that would be chosen by a social planner whose objective was to maximize the expected welfare E(U). Since consumption is a random variable that takes the values  with probability Φ (T,t) that there is exactly t innovations between time 0 and time t, given by:
with probability Φ (T,t) that there is exactly t innovations between time 0 and time t, given by:
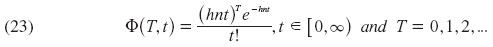
From this, the expected consumption is computed as follows6
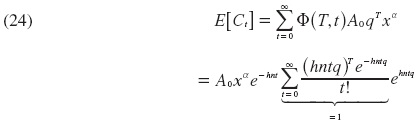
Then, the expected welfare becomes:

Hence the social planner will choose (x,n) to maximize the expected present value of lifetime consumption, subject to the labor constraint L = x + n.7 Then,

Given this level of research, the optimal growth rate is g* = hn* ln(q).
Equilibrium growth v.s. optimal growth
Given that the average growth rate is proportional to the number of researchers, it is sufficient to compare the optimal level of research with the equilibrium level of our economy. In order to simplify the comparison between n* and n we rewrite (26) and (19) respectively as:


As in the Aghion and Howitt (1992)'s model, we find the following basic differences between n* and n:
D1 The social discount rate r—hn* (q—1) is less than the private discount rate r + hn ("intertemporal-spillover effect").
D2 The private monopolist is unable to appropriate the whole output flow, but just a fraction (1 —α).
D3 The factor (q—1) corresponds to the so-called "business-stealing" effect, whereby the successful monopolist destroys the surplus attributable to the previous generation of intermediate good by making it obsolete.
Whereas distortions D1 and D2 tend to make the average growth rate less than optimal, D3 tends to make it greater. Due to the offsetting nature of these effects, the market average growth rate may be more or less than optimal. These three distortions summarize the main welfare implications of introducing creative destruction in the process of economic growth: laissez-faire growth may be either insufficient or excessive.
Additionally, we have two other differences due to the rigidities on the labor market, say:
D4 The optimal employment L — n* is bigger that the equilibrium employment L — n — u This is directly due to the bargaining power of unions.
D5 Global labor occupation (employment and R&D) is affected by a factor 1/(1 — τ)a cause of the distortive effect of taxes.
Clearly, D4 tends to make the average growth rate less than optimal. In contrast, D5 is growth enhancing only when 1 - τ < 1 i.e., when τ > 0. Nevertheless, the stark difference between distortions due to D1– D3 and those due to D4– D5, is that the two lasts depend on labor-market policy variables that, at least theoretically, can be controlled by the policy makers. This naturally suggest the question of whether variations in the policy variables, already present in the labor market, can reduce the gap between the optimal and the equilibrium growth rates caused by distortions D1 to D3. In other words, we are interested on issues as the following:
n > n*: If the negative externality that new innovators exert upon incumbent firms (D3) dominates, which kind of policy adjustments could be done to converge to the optimum?
n < n*: Conversely, if the intertemporal-spillover and the appropriability effects dominate (D1 and D2) and which policy could foster growth?
To answer these questions, we look to the impact of the policy variable τ on the research level:
Proposition. An increase in taxes leads to: (a) lower employment, (b) lower labor allocated to R&D and (c) higher unemployment.
Proof:
(a.):

(b.):
Expression (19) can be rewritten as:

From this:

(c.): From expressions (29), (30) and (6) it is easily deduced that  .
.
This suggests that when growth is excessive, some labor market rigidities are desirable because they can help to reduce the gap between the equilibrium rate of growth and the optimal one. In particular:
Corollary.
C1. When the economic growth is suboptimal, the optimal rate can be reached by subsidizing labor.
C2. Conversely, when the economic growth is excessive, the optimal rate can be reached by increasing labor taxes.
Conclusion
In this paper, we have shown the potential possibility of reaching the optimal growth rate using a simple growth model based on Aghion and Howitt (1992). We extended the basic framework by introducing a labor union, which bargains wages with monopolistic firms, and other labor market institutions such as labor taxes on labor income and unemployment benefits. We analyzed the several imperfections to shed light on how to reach the optimal growth rate by compensating the distortions on the goods-sector due to the growth process with the distortions induced by the labor market rigidities.
Even if our framework is so stylized that is not directly applicable to the actual problems related to growth, this theoretical exercise highlights interesting mechanisms and adds to the rich literature on Schumpeterian growth theory.
References
Adjemian, S., F. Langot, and C. Quintero-Rojas (2010). "How do Labor Market Institutions affect the Link between Growth and Unemployment: the case of European countries", The European Journal of Comparative Economics, 7. [ Links ]
Aghion, P., and P. Howitt (1992). "A Model of Growth through Creative Destruction", Econometrica, (60). [ Links ]
---------- (1998). Endogenous Growth Theory. MIT. [ Links ]
Lingens, J. (2003). "The impact of a unionised labor market in a Schumpeterian growth model", Labour Economics, (10), 91-104. [ Links ]
Mortensen, D. T. (2005). "Growth, Unemployment and Labor Market Policy", Journal of the European Economic Association. [ Links ]
Palokangas, T. (2004). "Union-Firm Bargaining, Productivity Improvement and Endogenous Growth", Labour, 18. [ Links ]
2 Results are qualitatively the same if we assume instead a continuum of perfectly substitute intermediate inputs.
3 There are hnυdt innovations over the small interval of time [t,t + dt]
4 Equivalently, the opportunity cost of R & D is the hourly net wage prevailing in the intermediate product sector, (1 — τ)wυ , times the expected duration of the innovation process,  .
.
5 It is easy to show by contradiction that 
6 The underbraced term is equal to 1 because it is the accumulative sum of Poisson probabilities.
7 Obviously, in an optimal setting there is no unemployment.














