Services on Demand
Journal
Article
Indicators
-
 Cited by SciELO
Cited by SciELO -
 Access statistics
Access statistics
Related links
-
 Similars in
SciELO
Similars in
SciELO
Share
Journal of the Mexican Chemical Society
Print version ISSN 1870-249X
J. Mex. Chem. Soc vol.58 n.2 Ciudad de México Apr./Jun. 2014
Article
Exploration of Diverse Interactions of Some Vitamins in Aqueous Mixtures of Cysteine
Mahendra Nath Roy,* and Palash Chakraborti
Department of Chemistry, University of North Bengal, Darjeeling-734013, India. mahendraroy2002@yahoo.co.in
Received September 17th, 2013
Accepted January 14th, 2014
Abstract
The apparent molar volume (ϕV), viscosity B-coefficient, molal refraction (R) and adiabatic compressibility (ϕK) of Nicotinic Acid, Ascorbic Acid, and Folic Acid have been determined in 0.01, 0.03, 0.05 mol··dm-3 aqueous Cysteine solutions at 298.15 K from density (ρ), viscosity (η), refractive index (nD) and speed of sound (u) respectively. The limiting apparent molar volumes (ϕV0) and experimental slopes (S*V), derived from the Masson equation, have been interpreted in terms of solute-solvent and solute-solute interactions respectively. The viscosity data were analyzed using the Jones-Dole equation and the derived parameters A and B have also been interpreted in terms of solute-solute and solute-solvent interactions respectively in the solutions. Using the Lorentz-Lorenz equation, molal refractions (R) have been calculated. At infinite dilution, limiting apparent molar adiabatic compressibilities (ϕK0) of these vitamins were evaluated and discussed.
Key words: Apparent molar volume, solute-solvent interaction, solute-solute Interaction, cysteine, nicotinic acid, ascorbic acid, folic acid.
Resumen
Fueron determinados el volumen molar aparente (ϕV), el coeficiente B de viscosidad, la refracción molar (R) y la compresibilidad adiabática (ϕK) de los ácidos nicotínico, ascórbico y fólico en soluciones acuosas de 0.01, 0.03, 0.05 mol·dm-3 cisteína (298.15 K) a partir de los valores experimentales de densidad (ρ), viscosidad (η), índice de refracción (nD) y (u), respectivamente. Los volúmenes molares aparentes límites (ϕV0) y las pendientes experimentales (S*V), obtenidas con la ecuación de Masson, han sido asociados a las interacciones soluto-solvente y solvente-solvente, respectivamente. Los datos de viscosidad fueron analizados utilizando la ecuación de Jones-Dole y los parámetros A y B obtenidos de este análisis fueron también asociados con las interacciones soluto-solvente y solvente-solvente, respectivamente. Por otra parte, las refracciones molales (R) fueron calculadas con la ecuación de Lorentz-Lorenz. Los valores de compresibilidad molar límite aparente (ϕK0) de estas vitaminas fueron obtenidos y discutidos.
Palabras clave: Apparent molar volume, solute-solvent interaction, solute-solute Interaction, cysteine, nicotinic acid, ascorbic acid, folic acid.
Introduction
A vitamin is an organic compound required by an organism as a vital nutrient in limited amounts. Vitamins are essential precursors for various coenzymes. These coenzymes are therefore required in almost all metabolic pathways [1]. Nicotinic Acid, commonly known as vitamin B3 [2], is a water-soluble vitamin, an essential micronutrient and a reactive moiety of the coenzyme nicotinamide adenine dinucleotide (NAD). Ascorbic acid, known as vitamin C is water soluble vitamin, required for the synthesis of collagen, the intercellular "cement" which gives the structure of muscles, vascular tissues, bones, and tendon. Vitamin C plays an important role for the synthesis of several important peptide hormones neurotransmitters and creatinine. It also enhances the eye's ability and delay the progression of advanced age related muscular degeneration [3]. Folic acid is water-soluble vitamin, known as vitamin B9 (folate). It is an essential vitamin that is yellow-orange in color, is reported to be present in photosensitive organs, various mammalian metabolic pathways, and possibly involved in photosynthesis [4]. Humans cannot synthesize folate inside body; therefore, folate has to be supplied through the diet to meet their daily requirements. Children and adults both require folic acid to produce healthy red blood cells and prevent anemia [5].
Cysteine is a semi-essential amino acid, which means that it can be bio-synthesized in human body [6] under normal physiological conditions if a sufficient quantity of methionine is available. Although classified as a non-essential amino acid, in rare cases, cysteine may be essential for infants, the elderly, and individuals with certain metabolic disease.
To interpret various interactions occurring in solutions, the volumetric, viscometric and interferometric behavior of solutes has been proved to be very useful. To obtain information on solute-solute, solute-solvent, and solvent-solvent interactions, studies on the effect of concentration (molality), the apparent molar volumes of solutes have been extensively used.
In view of the above and in continuation of our studies, we have undertaken a systematic study on the density, viscosity, refractive index and ultrasonic speed of some vitamins in aqueous cysteine solutions at 298.15 K and we have attempted to report the limiting apparent molar volume (ϕV0), experimental slopes (S*V), viscosity B-coefficients, molar refraction (R) and limiting apparent molar adiabatic compressibility (ϕK0) for the cited vitamins in aqueous cysteine solution. The nature and mode of the cysteine, interacting with the additionally input vitamins has also been discussed.
Experimental section
Source and purity of samples
The studied salts (Nicotinic acid, Ascorbic acid and Folic acid) and cysteine, puriss grade was purchased from Sigma-Aldrich, Germany and was used as purchased. The mass purity of salts were ≥0.99. The salts were dried from moisture at 353 K for 24 h, and then they were cooled and store in a desiccator prior to use. Triply distilled water with a specific conductance <10-6 S cm-1 was used for the preparation of different aqueous cysteine solutions. The physical properties of different mass fraction of aqueous cysteine mixture are listed in Table 1.

Apparatus and Procedure
Aqueous binary solution of cysteine was prepared by mass (Mettler Toledo AG-285 with uncertainty ± 0.0003 g), which are used as solvent. Stock solutions of the salts (vitamins) were also prepared by mass and the working solutions were obtained by mass dilution. The conversion of molarity into molality was accomplished using experimental density values. All solutions were prepared afresh before use. The experimental values of densities (ρ), viscosities (η), refractive indices (nD) and ultrasonic speeds (u) of solutions are reported in Table 2 and the derived parameters are reported in Table 3 and Table 4.
The densities of the solutions (ρ) were measured by means of vibrating-u-tube Anton Paar digital density meter (DMA 4500M) with a precision of ± 0.00005 g cm-3 maintained at ± 0.01 K of the desired temperature. It was calibrated by triply-distilled water and passing dry air.
The viscosities were measured using a Brookfield DV-III Ultra Programmable Rheometer with fitted spindle size-42. The viscosities were obtained using the following equation
η = (100/RPM) × TK × torque × SMC (1)
where RPM, TK (0.09373) and SMC (0.327) are the speed, viscometer torque constant and spindle multiplier constant, respectively. The instrument was calibrated against the standard viscosity samples supplied with the instrument, water and aqueous CaCl2 solutions [7]. Temperature of the solution was maintained within ± 0.01 °C using Brookfield Digital TC-500 temperature thermostat bath. The viscosities were measured with an accuracy of ± 1.0% [viscosity of 0.01 molar aqueous CaCl2 solution is 0.896 mPa s (at 25 °C), water is 0.890 mPa s (at 25 °C)]. Each measurement reported herein is an average of triplicate reading with a precision of 0.3%.
Refractive index was measured with the help of a Digital Refractometer Mettler Toledo (Refracto 30 GS). The light source was LED, λ = 589.3 nm. The refractometer was calibrated twice using tripply distilled water, benzene and dry air and calibration was checked after every few measurements. The uncertainty of refractive index measurement was ± 0.0002 units.
The ultrasonic speed (u) was measured by multi frequency ultrasonic interferometer (Model M-81) from Mittal Enterprises, India. The interferometer working at 5 MHz is based on the same principle as was used by Freyer et al. [8] and Kiyoharo et al. [9]. The obtained speeds were corrected for diffraction errors as given by Subrahmayan et al. [10]. The uncertainty in the speed is ±0.2 m s-1. The temperature was controlled within ±0.01 K using a Lauda thermostat during the measurement.
Results and Discussions
Density measurement
Apparent molar volumes (ϕV) were determined from the solution densities using the equation 2 [11].
ϕV = M/ρ 1000(ρ - ρ0)/mρρ0 (2)
where M(g mol-1) is the molar mass of the solute, m(mol kg-1) is the molality of the solution, ρ0 (kg m-3) and ρ (kg m-3) are the densities of the mixture and the solution respectively. The plots of ϕV against square root of molal concentration (√m) were found to be linear. Using a least-square treatment to the plots of ϕV versus √m using the Masson equation, equation 3 [12], the limiting apparent molar volume ϕV0 was calculated.
ϕV = ϕV0 + S*V √m (3)
where ϕV0 is the limiting apparent molar volume at infinite dilution and S*V is the experimental slope. Values of ϕV0 and S*V are reported in Table 4.
A glance of Table 4 shows that ϕV0 values for vitamins are positive and increase with increasing concentrations in aqueous cysteine mixture, indicating the presence of strong solute-solvent interactions and these interactions are further strengthened as increases the mass fraction of cysteine in the mixture. A probable interaction pattern is shown in scheme 1.

Interaction of vitamins with cysteine increases with increasing interacting centre of vitamins. The trend in the solute-solvent interaction is
Nicotinic Acid (NA) < Ascorbic Acid (AA) < Folic Acid (FA)
The S*V values of the vitamin solution given in Table 4 decreases with increase in the interactive centres of the studied vitamins and with increase in the mass fraction of cysteine in the solvent mixture rendering minimum solute-solute interaction.
The magnitude of ϕV0 (Fig. 1) values is much greater than those of S*V for all studies vitamins as well as mass fraction of cysteine in the mixture suggests that solute-solvent interactions dominate over solute-solute interactions.

Viscosity measurement
The viscosity data has been analyzed using Jones-Dole equation, equation 4 [13].
(η/η0 - 1)/m1/2 = A + Bm1/2 (4)
where η0 (mPa s) and η (mPa s) are the viscosities of the solvent and solution respectively, m(mol kg-1) is the molality of the solution. A( kg mol-1) and B( kg1/2 mol-1/2) are the viscosity co-efficient estimated by a least-squares method and are reported in Table 4. The values of the A co-efficient are found to decrease with the increase in the mass fraction of cysteine in solvent mixture. The results indicate the presence of very weak solute-solute interactions. These results are in excellent agreement with those obtained from S*V values discussed earlier.
The effects of solute-solvent interactions on the solution viscosity can be inferred from the B-coefficient [14, 15]. The viscosity B-coefficient is a valuable tool to provide information concerning the solvation of the solutes and their effects on the structure of the solvent. From Table 4 and Fig. 2 it is evident that the values of the B-coefficient are positive, thereby suggesting the presence of strong solute-solvent interactions, and strengthened with increase of mass fraction of cysteine in the solvent mixture, are in agreement with the results obtained from ϕV0 values discussed.

Refractive index measurement
The molar refraction, R can be evaluated from the Lorentz-Lorenz relation, equation 5 [16].
R = {(n2D - 1)/(n2D + 2)}(M/ρ) (5)
where R (cm3 mol-1), nD, M (gm·mol-1) and ρ (kg m-3) are the molar refraction, the refractive index, the molar mass and the density of solution respectively. The refractive index of a substance is defined as the ratio c0/c, where c is the speed of light in the medium and c0 the speed of light in vacuum. Stated more simply, the refractive index of a compound describes its ability to refract light as it moves from one medium to another and thus, the higher the refractive index of a compound, the more the light is refracted [17], as stated by Deetlefs et al. [18].
The refractive index of a substance is higher when its molecules are more tightly packed or in general when the compound is denser and with the increase of mass fraction of cysteine in solvent mixture refractive index value also increases. Hence a perusal of Table 2 & Table 3 we found that the refractive index and the molar refraction values respectively are higher for Folic Acid than Ascorbic Acid and Nicotinic Acid, indicating the fact that the molecules are more tightly packed in the mixture. The interaction in the solution is basically solute-solvent interaction and a small amount of solute-solute interaction. This is also good agreement with the results obtained from density and viscosity parameters discussed above. The trend in the package of the studied vitamins in aqueous mixture of cysteine is
Nicotinic Acid < Ascorbic Acid < Folic Acid.
Ultrasonic speed measurement
The adiabatic compressibility (β) was evaluated from the following equation:
β = 1/u2ρ (6)
where ρ (kg m-3) is the density of solution and u(ms-1) is the speed of sound in the solution. The apparent molal adiabatic compressibility (ϕK) of the solutions was determined from the relation [19].
ϕK = Mβ/ρ + 1000(βρ0 - β0ρ)/mρρ0 (7)
where β0, β are the adiabatic compressibility of the solvent and solution respectively and m (mol kg-1) is the molality of the solution. Limiting partial molal adiabatic compressibilities (ϕK0) and experimental slopes (S*K) were obtained by fitting ϕK against the square root of molality of the electrolyte (√m) using the method of least squares.
ϕK = ϕK0 + S*K · √m (8)
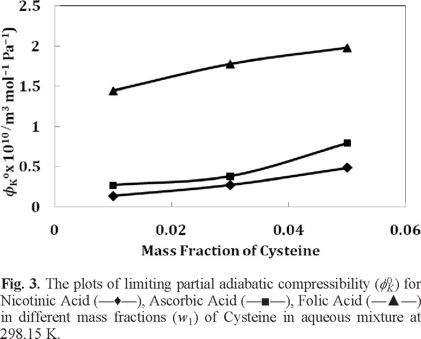
The values of β and ϕK are reported in Table 3. The values of ϕK0 (m3 mol-1 Pa-1) and S*K (m3 mol-3/2 Pa-1 kg1/2) are presented in Table 4. Since the values of ϕK0 and S*K are measures of solute-solvent and solute-solute interactions respectively, a perusal of Table 4 and Figure 3 shows that the ϕK0 values are in good agreement with those drawn from the values of ϕV0 discussed earlier.
Conclusion
The positive effects of the derived parameters, as limiting apparent molar volume (ϕV0), viscosity B-coefficients and limiting partial isentropic compressibility (ϕK0) suggested the presence of strong solute(vitamins)-solvent(aq. mix. of Cystine) interactions; which increases with the increase in the interacting centres (groups) of vitamins and with increase of mass fraction of cysteine in the aqueous mixture. The refractive index and the molar refraction values imply that Folic Acid molecules are more tightly packed in the solution leading to higher solute-solvent interaction than the other vitamins. The conclusions from experimental and derived parameters also provides important working function of the cysteine with vitamins in biological systems; which demands the uniqueness of the work.
Acknowledgement
The authors are grateful to the Departmental Special Assistance Scheme, Department of Chemistry, NBU under the University Grants Commission, New Delhi (No. 540/27/DRS/2007, SAP-1) for financial support and instrumental facilities in order to continue this research work.
One of the authors, Prof. M. N. Roy is thankful to University Grant Commission, New Delhi, Government of India for being awarded one time grant under Basic Scientific Research via the grant-in-Aid No. F.4-10/2010 (BSR) regarding his active service for augmenting of research facilities to facilitate further research work.
References
1. Robinson, F. A. The Vitamin B-Complexes, Chapter 4. Chapman & Hall, London, 1951. [ Links ]
2. Cakir, S.; Bulut, I.; Bicer, E.; Cakir, O. Coord. J. Chem. 2003, 56, 511-521. [ Links ]
3. Roy, M. N.; Das, R. K.; Bhattacharjee, A. Russian J. Phys. Chem. A 2010, 84, 2201-2210 [ Links ]
4. Chahidi, C. ; Aubailly, M.; Momzikoff, A.; Bazin, M. Photochem. Photobiol. 1981, 33, 641. [ Links ]
5. "Dietary Supplement Fact Sheet: Folate", Office of Dietary Supplements, National Institutes of Health, http://ods.od.nih.gov/factsheets/folate.asp [ Links ]
6. "The primary structure of proteins is the amino acid sequence". The Microbial World. University of Wisconsin-Madison Bacteriology Department. Retrieved 16 September 2012. [ Links ]]
7. Abdulagatov, I. M.; Azizov, N. D. Fluid Phase Equilibria 2006, 240, 204. [ Links ]
8. Freyer, E.B.; Hubbard, J.D.; Andrews, D.H. J. Am. Chem. Soc. 1929, 51, 759. [ Links ]
9. Kiyohara, O.; Grolier, J. P. E.; Benson, G. C. Can. J. Chem. 1974, 52, 2287 [ Links ]
10. Murthy, N. M.; Subrahmanyam, S. V. Bull. Chem. Soc. Jpn. 1977, 50, 2589. [ Links ]
11. Ayranci, E. J. Chem. Eng. Data 1997, 42, 934-937. [ Links ]
12. Masson, D. O. Phil. Mag. 1929, 8, 218-226. [ Links ]
13. Jones, G.; Dole, M. J. Am. Chem. Soc. 1929, 51, 2950-2964. [ Links ]
14. Millero, F. J.; Chem. Rev. 1971, 71, 147-176. [ Links ]
15. Millero, F. J.; Losurdo, A.; Shin, C. J. Phys. Chem. 1978, 82, 784-792. [ Links ]
16. Minkin, V.; Osipov, O.; Zhdanov, Y. Dipole Moments in Organic Chemistry, Plenum Press, New York, London, 1970. [ Links ]
17. Born, M.; Wolf, E.; Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light, 7th ed., Cambridge University Press, London, 1999. [ Links ]
18. Deetlefs, M.; Seddon, K.; Shara, M. Phys. Chem. Chem. Phys. 2006, 8, 642-649. [ Links ]
19. Roy, M. N.; Ekka , D.; Dewan, R. Acta Chim. Slov. 2011, 58, 792-796. [ Links ]














