1 Introduction
Cylindric algebras were introduced by A. Tarski in the 1940s with the intention of providing an algebraic counterpart to the first–order predicate calculus. As a general reference we mention the fundamental work by Henkin, Monk and Tarski [7].
In particular, the class of diagonal–free two– dimensional cylindric algebras constitute an algebraic counterpart to the first–order predicate calculus without identity and considering just two variable symbols in the language.
Formally a diagonal–free two–dimensional cylindric algebra is a Boolean algebra enriched with two existential quantifiers which commute.
This class of algebras will be denoted Df2, in agreement with the notation introduced in [7]. Besides, the class Df2 constitute a variety (that is, it can be described by means of a finite number of equations) and has been widely studied.
However, little research has pursued to investigate those problems inherent to finite algebras. On the other hand, a monadic Boolean algebra is any pair (A,∃) formed by a Boolean algebra A enriched with an existential quantifier ∃ defined on A (see [6]) and, within the context of cylindric algebras, these algebras are diagonal-free one-dimensional cylindric algebras or Df1−algebras.
As we said, the variety Df2 has been widely investigated by different authors. Among other known results, it can be mentioned that D. Monk studied the lattice Λ(Df2) of all subvarieties of Df2 and proved that it has ℵ0 elements (subvarieties).
This author also showed that every element of Λ(Df2) has a finite base and a decidable equational theory. Later, N. Bezhanishvili, in [1], proved that every proper subvariety of Df2 is locally finite although Df2 is not.
On the other hand, some problems inherent to finite algebras have also been studied.
For instance, in [3], the author exhibited a connection between Df2−algebras and pairs formed by a monadic Boolean algebra and a certain subalgebra of it; and as a consequence, it was obtained a formula to calculate the number of monadic subalgebras of a given finite monadic Boolean algebra.
Also, in [4], formulas for computing the number of Df2−algebra structures that can be defined over a finite Boolean algebra as well as the fine spectrum of Df2 were obtained.
Finally, the lattice Λ(Df2) was studied and a full description of the poset of all its joint–irreducible elements was given.
Besides, in [5] A. V. Figallo and C. M. Gomes studied the variety of Tkm−algebras, this is, monadic Boolean algebras endowed with a monadic automorphism of period k and established, in the finite case, the relationship between this variety and the variety Df2.
It is worth mentioning that the study of the lattice of all subalgebras of an abstract algebra has interested many authors.
For instance, G. Birkhoff and O. Frink, [2], characterized the subalgebra lattices of universal algebras as algebraic lattices.
On the other hand, in [8], the author proved that every algebraic lattice is isomorphic to the subalgebra lattice of a square of some universal algebra.
The purpose of this paper is to study some properties related to the subalgebras of a finite diagonal–free two–dimensional cylindric algebra.
In section 2, we recall some well-known facts about Df2−algebras, we emphasize, in particular, those which refer to finite algebras and which were stated in [3]; [4] and [7].
The main results of this work are in section 3. There, we define an order over the family of certain partitions of the set of atoms of a finite Df2−algebra.
As a consequence of this and other results stated in section 2, we obtain a full description of the lattice of all subalgebras of a finite Df2−algebra.
2 Preliminaries
In this section, we shall review some notions and results concerning finite Df2−algebras will be used to obtain the main result of this work. We refer the interested reader to the references [3, 4].
Recall that a Boolean algebra is a structure A=(A,∨,∧,¬,0,1) such that (A,∨,∧,0,1) is a bounded distributive lattice with first element 0, last element 1 and where ¬a is the Boolean complement of a, for every a∈A.
A Df2−algebra is a triple (A,∃1,∃2), where A is a Boolean algebra and ∃1, ∃2 are quantifiers defined on A that commute, that is ∃1 and ∃2 are unary operators on A,∃1:A→A(i=1,2), that verify the following conditions:
∃i0=0,
(1)
x≤∃ix,
(2)
∃i(x∧∃iy)=∃ix∧∃iy,
(3)
∃i∃jx=∃j∃ix.
(4)
For 1≤i,j≤2 and i≠j. The first three are the defining conditions of existential quantifier. In what follows we will denote the Boolean algebra with n atoms by Bn and by Π(Bn) the set of its atoms.
It is well known that there is an onto and one-to-one correspondence between the family of all quantifiers that can be defined over Bn, and the family of all Boolean subalgebras of Bn. Indeed, if S is a subalgebra of Bn, then the map ∃:Bn→Bn∃ : Bn → Bn defined by:
∃(x)=∧{s∈S:x≤s}.
(5)
Is a quantifier which will be called the quantifier associated to S. Moreover, all quantifiers on Bncan be obtained in this way.
On the other hand, every subalgebra S of Bn induces a partition PS of the set Π(Bn) of its atoms which will be called partition induced by S and it is obtained, by considering the set Π(S) of the atoms of S, in the following way:
C∈PS,iff
(6)
there is s∈Π(S) such that s=∨a∈Ca.
(7)
Conversely, every partition P of Π(Bn) induces a subalgebra SO of Bn as follows: for every C∈P, we consider the element aC=∨a∈Ca.
Then, SP is the Boolean subalgebra generated by the set {aC:C∈P}. From the above, we can conclude that there is an onto and one-to-one correspondence between the family of all quantifiers that can be defined over Bn, and the family of all partitions of Π(Bn).
Let ∃ be an arbitrary quantifier defined on Bn and let P be the partition of Π(Bn) associated to ∃. Then, we denote by P(x) the set:
{C∈P:∨a∈Ca≤x}.(
8)
For each x∈∃Bn. The following definition plays an important role when dealing with finite Df2−algebras and was introduced in [3].
Definition 1. Let P1 and P2 be two partitions of Π(Bn). For each C∈Pi, we will call mj–saturated of C, and we will denote it by mj(C), the least (in the sense of inclusion) subset of Pj which verifies C⊆∪F∈mj(C)F, for 1≤i,j≤2 and i≠j.
Then, we can determine the mj–saturated of any C∈Pi, with i≠j, 1≤i,j≤2, as it is indicated in the next lemma.
Lemma 1. If C∈Pi and b=∨a∈Ca, then mj(C)=Pj(∃jb), with 1≤i,j≤2 and i≠j.
Another characterization of mj(C), for any C∈Pi, is given next.
Lemma 2. If C∈Pi, then mj(C)={D∈Pj:C∩D≠0}, 1≤i,j≤2 and i≠j.
Remark 1. If C∈Pi and b=∨a∈Ca, then ∃jb can be calculated in the following way:
∃jb=∨a∈DD∈mj(C)a.
(9)
For 1≤i,j≤2 and i≠j.
Next, we define a binary relation between two partitions of Π(Bn).
Definition 2. Let P1 and P2 be two partitions of Π(Bn). We will say that P2 is a refinement of P1 and we will write P2≻P1, if for each C∈P1 there exists U⊆P1 such that:
∪G∈m2(C)G=∪F∈UF.
(10)
Remark 2. It is not difficult to check that the subset U, mentioned in Definition 2, is unique. Therefore, from now on, for each C∈P1, we will denote with UC the only subset of P1 such that:
∪G∈m2(C)G=∪F∈UcF.
(11)
A characterization of UC, for every C∈Pi, is stated in the following lemma.
Lemma 3. If C∈Pi and b=∨a∈Ca, then UC=P(∃jb), with 1≤i,j≤2 and i≠j.
In what follows, we will write P2≈P1 to indicate that each of the partitions is a refinement of the other. The following three results are the most important in this section and, as we shall see later, they will be very useful. A detailed proof of them can be found in [3].
Theorem 1. Let P1 and P2 be two partitions of Π(Bn) and ∃1, ∃2 their associated quantifiers. Then the following conditions are equivalent:
∃1 and ∃2 commute,
P1≈P2.
Lemma 4. Let (Bn,∃) be a finite monadic Boolean algebra, S a Boolean subalgebra of Bn, and let P2 and P1 be the partitions of Π(Bn) associated to the quantifier ∃ and the subalgebra S, respectively. Then the following conditions are equivalent:
S is a monadic subalgebra of (Bn,∃),
P2≻P1.
Lemma 5. Let P1, P2 be two partitions of Π(Bn). If P2≻P1, then P1≻P2.
3 Df2−Subalgebras of a Finite Df2−Algebra
In this section, we shall present a correspondence between the family of all subalgebras of a given Df2−algebra A=(Bn,∃1,∃2) and a certain family of partitions of the set of its atoms Π(Bn).
This will allow us to establish a characterization of the lattice of all subalgebras of A. A characterization of the subalgebras of a finite Df2−algebra is the following:
Lemma 6. Let A=(Bn,∃1,∃2) be a finite Df2−algebra, Pi the partition of Π(Bn) associated to ∃i, i=1,2, and S a Boolean subalgebra of A. Then the following conditions are equivalent:
1. S is a Df2−subalgebra of (Bn,∃1,∃2),
2. PS≈Pi for i=1,2, with PS the partition of Π(Bn) associated to S.
Proof. It is consequence of Lemma 4.
If A=(Bn,∃1,∃2) is a given finite Df2−algebra, we denote the set of all Df2−subalgebras of A by S(A) and the set of all partitions P of Π(Bn) such that O≈Pi for i=1,2, by ℘(A), where Pi is the partition of Π(Bn) associated to ∃i. Then, from the previous lemma, the following corollary is inferred:
Corollary 3.1. S(A) and ℘(A) have the same cardinality.
Now we will endow ℘(A) with an order relation ≼ defined as follows:
P≼P′iff(
12)
For all C∈P′, there is Q⊆P such that C=∪D∈QD.(
13)
Then we have:
Lemma 7. Let A=(Bn,∃1,∃2) be a finite Df2−algebra. Then, the ordered sets (S(A),⊆) and (℘(A),≼) are antiisomorphic.
Proof. Let α:S(A)→℘(A) be the application defined by:
α(S)=PS for each S∈S(A),
(14)
where PS is the partition of Π(Bn) associated to S. It is not difficult to check that α is one-to-one and onto. Now, let S1,S2∈S(A) such that (1) S1⊆S2. For each C∈α(S1), let:
d=∨a∈Ca.
(15)
Then, d∈Π(S1). From (1) d∈S2 and so, we may assert that d=∨b∈Π(S2)b≤db. Therefore,
C=∪D∈PS2(d)D.
(16)
And so, α(S2)≼α(S1). On the other hand, suppose that (2) α(S2)≼α(S1), and let d∈Π(S1). Then:
d=∨a∈Ca.
(17)
For some C∈PS1. Then, from (2), we have that C=∪D∈QD, with Q⊆PS2. Let us assume that Q={D1,D2,…,Dr} and let bi=∨a∈Dira where 1≤i≤r. Then, bi∈Π(S2) and d=∨i=1bi, that is to say, d∈S2. In this way, S1⊆S2.
Our next objective is to determine necessary and sufficient conditions for two elements of S(A) to be isomorphic. For this purpose, let S1 and S2 be two elements of S(A).
For each C∈PS1(D∈PS2) we will denote the saturated of C(D) in the partition Pi by miS1(C)(miS2(D)). Besides, we will denote by UCi S1(UDi S2) the least subset of PS1(PS2), such that:
∪H∈miS1(C)H=∪G∈UCi S1G(∪I∈miS2(D)I=∪F∈UDi S2F).
(18)
Lemma 8. Let A=(Bn,∃1,∃2) be a finite Df2−algebra, S1 and S2 Df2−subalgebras of A. Then, the following conditions are equivalent.
1. S1 and S2 are isomorphic,
2. there is a bijection f:PS1→PS2 such that:
∪G∈f(UCi S1)G=∪H∈miS2(f(C))H
(19)
For each C∈PS1 and i=1,2.
Proof. (i) ⇒ (ii). Let S1 and S2 be isomorphic Df2−subalgebras of A, and let ϕ:S1→S2 be the corresponding Df2−isomorphism. Let us define f:PS1→PS2 by:
f(C)=D∈PS2,iff
(20)
ϕ(∨a∈Ca)=∨b∈Db for every C∈PS1.
(21)
Then, it is clear that f is well defined. Besides, since ϕ|Π(S1) is a one-to-one and onto correspondence between Π(S1) and Π(S2), we can assert that f is one-to-one and onto. Let us prove that, for each C∈PS1, it holds:
∪G∈f(UCi S1)G=∪H∈miS2(f(C))H.
(22)
For i=1,2. Suppose that s1=∨a∈Ca, then:
ϕ(s1)=ϕ(∨a∈Ca)=s2=∨b∈f(C)b.
(23)
With s1∈Π(S1) and s2∈Π(S2). It can be verified without any difficulty that:
ecl(∃is1)=ϕ(∨a∈HH∈miS1(C)a)=ϕ(∨a∈GG∈UCi S1a)=ϕ(∨G∈UCi S1∨a∈Ga).
(24)
And, since ∨a∈Ga∈S1 for every G∈UCi S1, we get:
ϕ(∃is1)=∨G∈UCi S1ϕ(∨a∈Ga)=∨G∈UCi S1∨b∈f(G)b.
(25)
On the other hand:
∃iϕ(s1)=∃is2=∨b∈II∈miS2(f(C))b.
(26)
From ϕ(∃is1)=∃iϕ(s1), (1) and (2), we get:
∨G∈UCi S1∨b∈f(G)b=∨b∈II∈miS2(f(C))b.
(27)
From (3), and properties of UCi S1 and miS2(f(C)), it results that:
∪G∈f(UCi S1)G=∪H∈miS2(f(C))H.
(28)
(ii) ⇒ (i). Let f:PS1→PS2 be a one-to-one and onto function such that:
∪G∈f(UCi S1)G=∪H∈miS2(f(C))H
(29)
for every C∈PS1 and i=1,2. Let ψf:S1→S2 be the Boolean homomorphism defined by:
ψf(s)=∨H∈PS1(s)∨r∈f(H)r.
(30)
Since f is one-to-one and onto, it is easy to check that ψf is a Boolean isomorphism. Let us now check that ψf(∃is)=∃iψf(s) for every s∈Π(S1). Let s∈Π(S1), then there is C∈PS1 such that s=∨a∈Ca. By Lemma 3, we have:
ccrψf(∃is)=∨H∈PS1(∃is)∨r∈f(H)r=∨H∈UCi S1∨r∈f(C)r=∨G∈f(UCi S1)∨r∈Gr.
(31)
On other hand, it is clear that:
∃i(ψf(s))=∃i(∨a∈f(C)a)=∨D∈miS1(f(C))∨a∈Da.
(32)
From (4), (5) and (6), we get that ψf(∃is)=∃i(ψf(s)).
Now, consider the binary relation Δ on ℘(A) defined as:
P1ΔP2,iff
(33)
P1 and P2 satisfy condition Lemma 8 (ii).
(34)
Then, from all the results above stated, we have:
Theorem 2. The subalgebra lattice of the finite Df2−algebra A, S(A), is isomorphic to (℘(A)/Δ,≽).
Finally, we analyze some examples where we apply the result stated above.
Example 1. Let us consider the Df2−algebra, (B2,∃1,∃2) whose Hasse diagram is shown below and the quantifiers ∃1, ∃2 are defined by the next table.
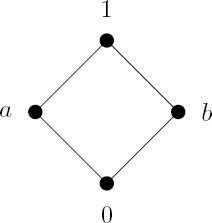 |
|
x |
∃1x |
∃2x |
| 0 |
0 |
0 |
| a |
a |
1 |
| b |
b |
1 |
| 1 |
1 |
1 |
In this case P1={{a},{b}} and P2={{a,b}} are the only partitions of Π(B2) associated to quantifiers ∃1 and ∃2, respectively.
Then ℘(B2)={P1,P2}, hence it is clear that (S(B2),⊆) is the chain with two elements and (B2,∃1,∃2) has two non-isomorphic subalgebras.
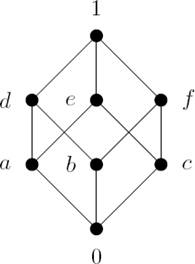
Example 2. Let (B3,∃1,∃2) be the Df2−algebra whose Hasse diagram is below and the quantifiers ∃1, ∃2 are given by the table:
Hence, P1={{a},{b,c}} and P2={{a,b,c}} are the partitions of Π(B3) associated to quantifiers ∃1 and ∃2, respectively.
 |
|
x |
∃1x |
∃2x |
| 0 |
0 |
0 |
| a |
a |
1 |
| b |
f |
1 |
| c |
f |
1 |
| d |
1 |
1 |
| e |
1 |
1 |
| f |
f |
1 |
| 1 |
1 |
1 |
Then ℘(A)π(B3)={P1,P2,P3} where P1={{a},{b},{c}}, P2={{a},{b,c}} and P3={{a,b,c}}.
It is easy to verify that P1≼P2≼P3, hence (S(B3),⊆) is the chain with three elements and (B3,∃1,∃2) has three non-isomorphic subalgebras.
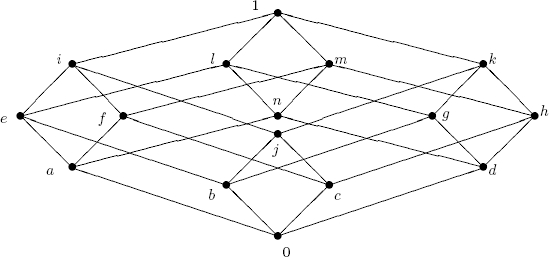
Example 3. Finally, let us consider the Df2−algebra, (B4,∃1,∃2) whose Hasse diagram is below and the quantifiers ∃1 and ∃2 are defined by the partitions P1={{a,b},{c,d}} and P2={{a,c},{b,d}} of Π(B4), respectively.
Then ℘(B4)={P1,P2,P3,P4,P5} where:
P1={{a},{b},{c},{d}},
(35)
P2={{a,b},{c,d}},
(36)
P3{{a,c},{b,d}},
(37)
P4{{a,d},{b,c}},
(38)
P5{{a,b,c,d}}.
(39)
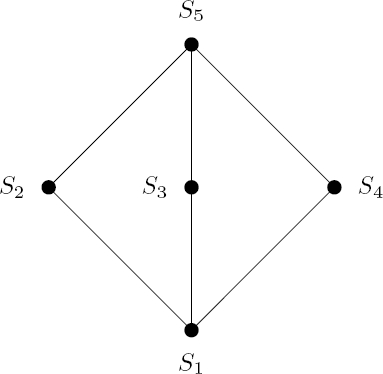
It can be seen that P1≼P2≼P5; P1≼P3≼P5; P1≼P4≼P5 and P2≼P3≼P4 are incomparable.
Then (S(B4),⊆) is the ordered set whose Hasse diagram is indicated next. Hence, the Df2−algebra (B4,∃1,∃2) has five non-isomorphic subalgebras.











 nueva página del texto (beta)
nueva página del texto (beta)