1. Introduction

Eighty years ago, Michels et al. ¹ used the confined hydrogen atom (CHA) as a model to study the change in the polarizability of a hydrogen atom subjected to high external pressure. In this model, the nucleus of the hydrogen atom was clamped at the center of an impenetrable sphere of radius R _c , while the electron could move within the included volume. In this system, ionization is not possible. The way to account for ionization is to allow the walls to be penetrable. This penetrable model was successfully used by Ley-Koo and Rubinstein² to explain the ionization of a hydrogen atom trapped in alpha-quartz^3-4.

Many applications have been developed from these models and they have been generalized to cavities with different geometries. This model has subsequently been applied to a wide range of physical problems ^1-13. Observable properties of the systems such the energy spectrum, transition frequencies and probabilities, polarizability and the behavior of atoms trapped in fullerenes, etc., are changed by spatial confinement. Reviews and books on those topics are available ⁵. Recent experimental studies show that the electron capture nuclear decay rate is increased under compression ^14-16. A partial explanation of this effect was given using the model of many-electron atoms confined in an impenetrable spherical cavity ¹⁷. With the advent of technology to construct atomic scale confinements, the study of confined systems has become increasingly relevant.

In most of the works on the properties of atoms and molecules, either free of any confinement or confined in cavities, it is assumed that the nuclei are points with charge and mass but without extent. The inclusion of a nucleus of finite size in the free hydrogen atom is accompanied by a shift in the electron energy ^20-26. The magnitude of this shift is very small compared to the energy of the hydrogen atom with a point nucleus. Until now, no one has studied how this energy shift changes for the hydrogen atom, confined in spherical penetrable cavities, when a nucleus of finite size is considered.

Pyarelal and Bhatnagar ²⁰ proposed a model of the hydrogen atom with an impenetrable nucleus of finite size. In that model, the wave functions must vanish at the surface of the nucleus. The reduction in the volume available for movement of the electron produces an increase in the energy of the electronic states. This problem has an exact solution, but the model is unrealistic.

A more realistic model consists of a spherical nucleus of radius r ₀ , with an uniform distribution of charge. For hydrogen-like atoms with a small nuclear charge, Z, it is well-known that the Schrödinger equation adequately describes those systems ¹⁹. An exact solution to this problem was found by Ley-Koo et al. ¹⁹, in which they studied the muonic atoms, free of any confinement, with Z up to 90. In this work, we will adopt the model of a spherical nucleus with an uniform charge distribution, and we will use first-order perturbation theory to calculate the correction to the ground-state energy of the hydrogen atom confined in spherical penetrable and impenetrable cavities, as a function of the radius of confinement R _c .

The objectives of this work are twofold: the former is to establish benchmark values for the energy of the ground state of the hydrogen atom confined in a spherical penetrable cavity, the second is to calculate the energy shift of this system when considering a spherical nucleus of finite volume with an uniform distribution of charge.

The organization of this work is as follows: in Sec. 2, we solve CHA with a point nucleus in a spherical, padded cavity. In Sec. 3 we use first-order perturbation theory to compute the energy correction due to inclusion of a nucleus of finite size. In Sec. 4 we show the results of the calculation of the energy correction due to a finite size nucleus, obtained for both penetrable and impenetrable confinements. Finally, in Sec. 5 we give our conclusions.

2. The CHA in a spherical padded cavity

In this section we give a brief description of the solution of the hydrogen atom confined in a spherical padded cavity. A detailed explanation can be found in Refs. 2, 7 and 9.

In atomic units (m=e=ℏ=1), the Schrödinger equation for a hydrogen atom at the center of a sphere of radius R _c and confined by a constant potential V ₀ is

where the potential is

Equation (1) can be solved using separation of variables

where Yl,m(θ,ϕ) is a normalized spherical harmonic and R(r) is a radial function composed of Ri(r), the wavefunction inside the sphere and Re(r), the wavefunction external to the sphere.

The Schrödinger equation for the inner region 0≤r≤Rc

whose solution is given by ^8-9:

where M(a,b,r) is the confluent hypergoemetric function ¹⁸ and

The external region, r>Rc, is described by the Schrödinger equation

which can be written as

where

and whose solution is given by ²

Hereafter, we restrict our attention to states with l=0 because these are the only states which have non-zero value of Ri(r) when r=0.

The eigenvalues are determined by the requirement that the total wavefunction must be continuous with continuous first derivatives at r=Rc. This is most easily accomplished by matching logarithmic derivatives at R _c , resulting in the equation

The normalization constants A and B are found from the requirements that

and the normalization condition

As mentioned above, the zeroes of the logarithmic derivative equation are the eigenvalues of the problem. The first zero corresponds to the ground state energy. With this value, we construct the wave function in each region. To find the zeroes of the equation we used Mathematica 9 with the command FindRoot with 50-digit precision variables. The eigenvalues obtained through this procedure are shown in Tables I, II, III, V as a function of the box radius R _c and the potential height V 0 . These results are in complete agreement with previous calculations ^2,7,9. The results are shown with 15 digits after the decimal point.

3. Finite nucleus size correction

The Hamiltonian of the hydrogen atom with a finite nucleus confined by a spherical penetrable wall is given by

where

The first two terms in Eq. (15) are the usual terms of a free hydrogen atom with a finite size nucleus ^6,19,21-23, and 𝑟 0 is the radius of the hydrogen atom, a proton. The third term is introduced to confine the hydrogen atom in a spherical cavity of radiusR _c .

We define the potential

Adding and subtracting Ṽ(r) to Eq. (14), and grouping terms we obtain the

This Hamiltonian can be written in the more familiar form

From Eq. (17) we immediately identify the first two terms as the Hamiltonian of a hydrogen atom confined in a penetrable spherical cavity, as analyzed in previous section. We note that forH ⁰ , the unperturbed Hamiltonian, the eigenfunctions ψnlm0 and eigenvalues Enl0 are well-known.

The perturbation is given by ^{(6, 21-23)}

As mentioned above, we are only interested in states with l=0, because the electron density is non-zero at the origin. The correction of the energy to first-order is given by

A straightforward calculation gives the following expression. The eigenfunctions ψn00 are an orthonormal set of wave functions with the form

Substituting (21) in (20) we obtain

In the region r≤r0, taking in account that for a proton, r0≪1,

Equation (22) then becomes

In this work we will use the most recent measured proton radius r0=0.8335 femtometers (1.575086726×10-5 bohrs) ²⁴.

4. Shannon entropy

Claude E. Shannon in 1948 introduced the so called Shannon entropy in his paper “A Mathematical Theory of Communication” ²⁷. The quantum version of Shannon entropy 𝑆 𝑟 , in configuration space is defined as

where ρ(r⃗) is probability density of the electron, in atomic units.

The Shannon entropy in quantum computation means the absolute limit of the best possible lossless compression of any communication, under some particular constraints ³³. On the other hand, the Shannon entropy has a wide variety of applications in Physics and Chemistry ³⁴. Usually, it is interpreted as the uncertainty associated with the particle position, which is related with the degree of localization (delocalization) of the particle. In chemistry, the Shannon entropy is associated with the delocalization of an electron in aromatic compounds ³⁵.

Panos et al. ³⁶, computed and plotted S _r as a function of the atomic number, Z, for many-electron atoms. They found that the curve Sr(Z) has relative minimum values for the closed shell atoms as He, Ne, Ar and Kr. They interpreted this fact as the electronic density of those atoms is more compact (localized) than their near neighbors. Recently, this interpretation for the Shannon entropy was used successfully in the study of many-electron atoms confined in soft spherical cavities ^37,38. In this work we will adopt this interpretation for the Shannon entropy.

5. Results

In Tables I, II, III, we show the corrections to the ground-state energy of CHA, taking into account a nucleus with finite size. The correction to the energy is small compared with the energy of the unperturbed confined atom. As the confinement radius R _c grows, E10(1) approaches the value of the first-order correction of the free hydrogen atom, E10free(1)=0.9923592777×10-10 hartrees, according to Eq. (24).We can see that there is a change in the value of the energy correction that depends on R _c andV ₀ . This behavior can be seen more clearly in Fig. 1, where we have plotted the ratio between the correction of the ground state energy of CHA due to the finite nucleus, relative to the correction for the free hydrogen atom. It should be noted that this ratio is independent of the value of r ₀ and depends only on the ratio of the wave functions evaluated at the origin

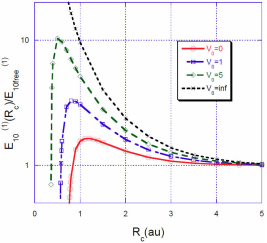
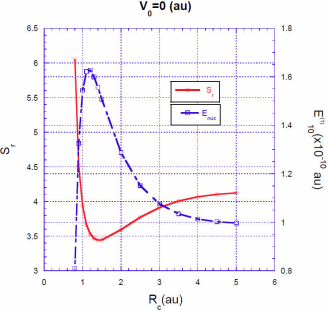
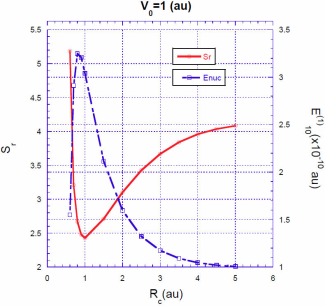
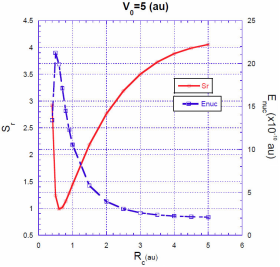
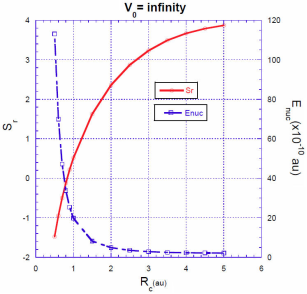
FIGURE 1 Ratio E10(1) (Rc)=E10free(1) 10free of the energy correction of CHA to the free hydrogen atom as a function of Rc. 

where Rfree(r) and R10(r) are the radial wave functions of the free hydrogen atom and the CHA, respectively.

For a spherical cavity with impenetrable walls, the correction to the energy grows rapidly as R _c tends to zero because by reducing R _c the electron is closer to the nucleus without the possibility to escape. In Fig. 1 we can see that for a value of Rc=1, the energy correction E10(1) to the ground state of the CHA is 10 times greater than in the free hydrogen atom.

This value is in complete agreement with that calculated by Goldman and Joslin ⁶. They found that for very small values of 𝑅 𝑐 and very excited states, the correction of the energy, E10(1), can be several orders of magnitude greater than the value for the free atom.

For a fixed value of the barrier heightV ₀ , the situation is quite different. The energy correction E10(1) (R _c ) grows as R _c decreases, and it reaches its maximum value at a confinement radius that we call R _cmax . As R _c continues to decrease, the energy correction, E10(1)(Rc), decreases also, approaching zero as R _c approaches a critical radius, at which the total energy of the electron is equal to the height of the barrierV ₀ .

Intuitively, one expects that the energy correction E10(1) be higher in a small size cavity, in which the electron is closer the nucleus, i.e. a cavity in which the electronic density is more compact. One way to quantify the compactness of the electronic density is by means of the Shannon entropy ^27-38, as was mentioned above. This idea is supported in the interpretation of localization-delocalization associated with the Shannon entropy, S _r . A small value of S _r , means that the electron density is more localized around the nucleus ^30-32,36-38. At R _c , where the entropy curve, Sr(Rc), has a minimum value, the electron density is more compact around the nucleus, and thus the contribution to the energy correction E10(1)(Rc) is greater. In Figs. 2 to 5 we plot the energy correction E10(1)(Rc) and the Shannon entropy Sr(Rc) for a few values of V ₀ as a function of R _c . In Fig. 2 we plot together E10(1)(Rc) and Sr(Rc), as a function of the confinement radius, R _c , for V0=∞. We see that when R _c decreases, S _r also decreases. This means that the electron density around the nucleus increases and there is an increase in E10(1). This behavior continues as R _c diminishes.

FIGURE 2 Energy correction E10(1) and Shannon entropy Sr as a function of Rc for V₀ = 0 hartrees. 

In Figs. 3, 4, 5 we show the energy correction E10(1)(Rc) and the Shannon entropy S _r for fixed values of V ₀ . In all figures, we see that the curve of the energy correction E10(1)(Rc) reaches a maximum value at a confinement radius Rcmax which depends on the value of V ₀ . We can also see that the curve of the Shannon entropy Sr(Rc) reaches its minimum value at a confinement radius Rc*, 𝑖.𝑒., in a spherical box of radius Rc* the electron density is more compact around the nucleus. From Figs. 3-5 we can see that Rcmax<Rc*. This result can be interpreted in the following way. As R _c decreases, the value of S _r also decreases, and the density becomes more compact up to a maximum value at Rc*. As Rc continues to decrease, Sr begins to increase and the electron starts to be delocalized, the wave function increases its value at the origin and inside the barrier. If Rc continues decreasing, the value of the wave function at the origin decreases quickly, but it grows fast inside the barrier. This process continues until ionization take place.

FIGURE 3 Energy correction E10(1) and Shannon entropy Sr as a function of Rc for V₀ = 1 hartrees. 

FIGURE 4 Energy correction E10(1) and Shannon entropy Sr as a function of Rc for V₀ = 5 hartrees. 

FIGURE 5 Energy correction E10(1) and Shannon entropy Sr as a function of Rc for a spherical impenetrable of radius Rc. 

6. Conclusions

In this work we calculated the energies and wave functions, with high numerical precision, for the ground state of the hydrogen atom confined in a penetrable spherical cavity. We also calculated the energy correction due to a nucleus of finite size for the CHA as a function of R _c and V ₀ . For finite barriers, the curves of the energy correction reach a maximum value, while the curves of the Shannon entropy as a function of Rcreach a minimum value. The maximum of the energy correction is always close to the position at which the Shannon entropy has its minimum value, i.e. at the position in which the electron density is most compact.