PACS: 75.30.Kz; 75.75.-c; 75.70.-i
1. Introducction
Since the discovery of the exchange anisotropy by Meiklejhon and Bean in 1956 on Co-CoO particles 1, systems consisting of a ferromagnetic (FM) material in contact with an antiferromagnetic (AF) material have attracted much attention during the last decade. The term exchange anisotropy field, H
eb
, was coined to describe the magnetic interaction between the magnetic moments of the FM, and the magnetic moments of the AF just at the interface. The main features of these structures are a shift of the hysteresis curve along the applied field, an unusual increase in the coercivity, H
C
, of the FM compared with the bulk value, rotational hysteresis, and torque curves following purely sinusoidal symmetry. More recently, ferromagnetic resonance (FMR) profiles displaying an unidirectional anisotropy superposed to the usual uniaxial symmetry, has been reported 2. By the mid 1970’s, almost all significant research on AF-FM exchange coupling was reported on materials involving monoxide magnetic particles,untilthe seminal paper by Hempstead et al in 1978 3. They reported that depositing
Experimentally, a diversity of materials and methods has been employed to investigate the exchange bias phenomenon in magnetic bilayers and nanostructures. This is because, in general, the magnetic properties of these systems are highly affected by growth conditions and sample treatment, purity of the alloys, substrate temperature, film thickness, roughness, chemical stability of the alloys, interdiffusion of atoms at the interface, etc. The most extensively studied AF/FM bilayers are those based on the antiferromagnetic compound FeMn 6, however, is in general difficult to obtain, corrodes easily, and crystallizes in different phases. Besides FeMn, other compounds such as NiO, NiMn, PtMn, IrMn, are also employed as AF layer 7,8,9,10. This is because these materials may exhibit chemical stability, relatively simple crystalline structure, corrosion resistance, and are magnetically harder than FeMn. As FM layer, NiFe, NiFeCo, CoFe, are commonly used due to their soft magnetic properties, and because are easy to obtain 9,10. More recently, we have proposed the amorphous FM compound Fe4.6Co70.4Si15B10 to be employed as an alternative material in exchange-biased heterostructures and artificial interfaces 11. On the other hand, although several techniques are available to measure the magnetic properties of exchange-biased structures, most of the experiments are contradictory regarding the value of the exchange coupling field between the AF and the FM layer 12. An example of this, are MOKE loops and FMR curves, which yield to inconsistent conclusions when both measures are compared, being very sensitive to the films’ thickness. These discrepancies are indicative that the physical mechanisms responsible for the inter-film coupling at an AF/FM interface are yet not well understood.
The exchange coupling through an AF/FM interface is determined by the magnetic ordering, and is observed when the sample is field-cooled from a temperature above T
N
, but below
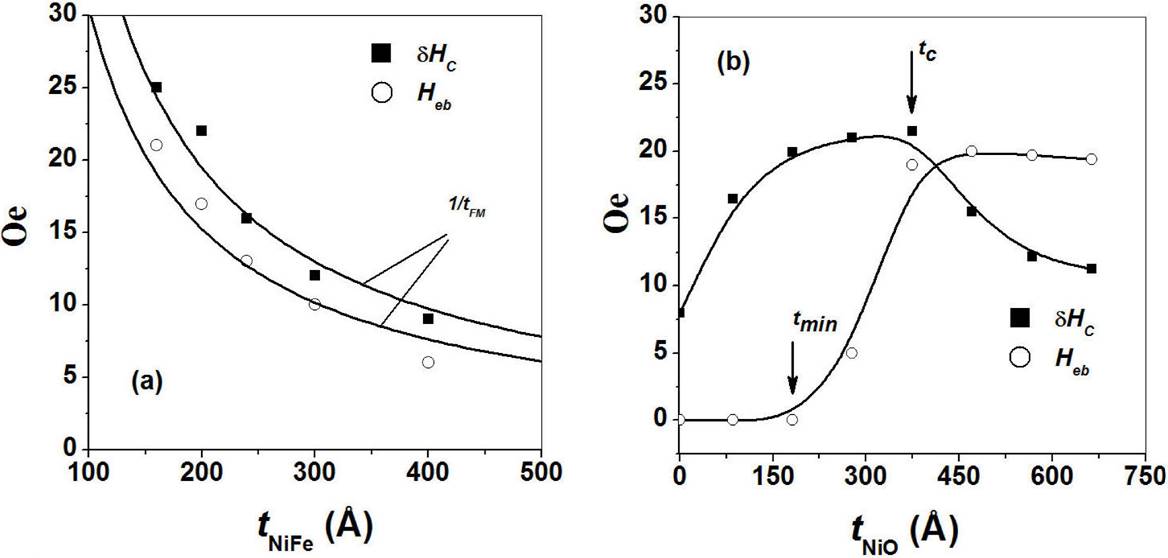
Another important aspect related to the physics of the exchange bias is the dependence on the thickness of the AF and FM layers, and the relation with the coercive field. Although this has been the subject of many theoretical 9,13 and experimental 14 studies in several AF/FM systems, there still exists controversy on the real origin of the phenomenology of exchange-coupled AF/FM bilayers. Two central features appear to be commonly reported in almost all investigations: (a) the dependence of the exchange bias and coercivity fields on the FM layer thickness follows the interface 1/t FM law; (b) there exist a critical AF layer thickness, below which the exchange bias field completely disappears. There is no doubt that these are mainly due to each sub-layer microstructure as long as the interface structure, which in turn, depends on the growth conditions.
The main goal of this study is to report on the dependence of the exchange bias field and coercivity in NiFe/NiO bilayers, as functions of both FM and AF films’ thickness. The in-plane angle symmetry is also studied. Unlike previous reports, in which different growth conditions are used from work to work, all samples treated here were grown under same experimental conditions. For this two series of samples, NiFe(200 Å)/NiO(t AF ) and NiFe(t FM )/NiO(375 Å), were grown onto Si(001) substrates by DC magnetron sputtering. The exchange bias and coercivity were characterized by measuring the hysteresis loop shift obtained by surface magneto-optical Kerr effect (SMOKE).
2. Experiment
A first series of samples with the AF film thickness fixed at 375 Å, and FM layer thickness, t
FM
, within the range 150-400 Å; and a second series of bilayers in which the FM film thickness was fixed at 200 Å, with AF layer thickness, t
AF
, within the range 86-660 Å, were grown by DC magnetron sputtering onto single crystalline Si(001) substrates commercially obtained. The substrates were cleaned in ultrasound baths of acetone and methanol for 10 min each, and then dried in flowing nitrogen. The base pressure of the system prior deposition was 2.0 x 10-7 Torr. The films were deposited in a 3.0 x 10-3 Torr argon atmosphere in the sputter-up configuration with the substrate held at a distance of 9 cm from the target. The electrical power was 20 W and substrate temperature of 130°C. The NiO layer was first deposited onto the substrate using reactive sputtering of Ni, with an O2 pressure of about
The magnetization curves were measured by surface magneto-optical Kerr effect (SMOKE) in the longitudinal geometry. In this configuration, the detected signal is proportional to the magnetization parallel to the aplied magnetic field. The ligth of a 2.0 mW He-Ne laser (632.8 nm), was linearly polarized at 45° with respect to the plane of incidence, and modulated at 50 kHz by a photoelastic modulator, striking the surface of the film at an angle of incidence of about 60°. Before detection, the reflected radiation passes through an analyzer in order to select the corresponding magnetization component. In order to measure hysteris loops with respect to in-plane angle,
3. Results an Discussion
The magnetization loops for the selected bilayer NiFe(200 Å)/NiO(375 Å)/Si (001), are shown in Fig. 1 for magnetic field orientations (a)
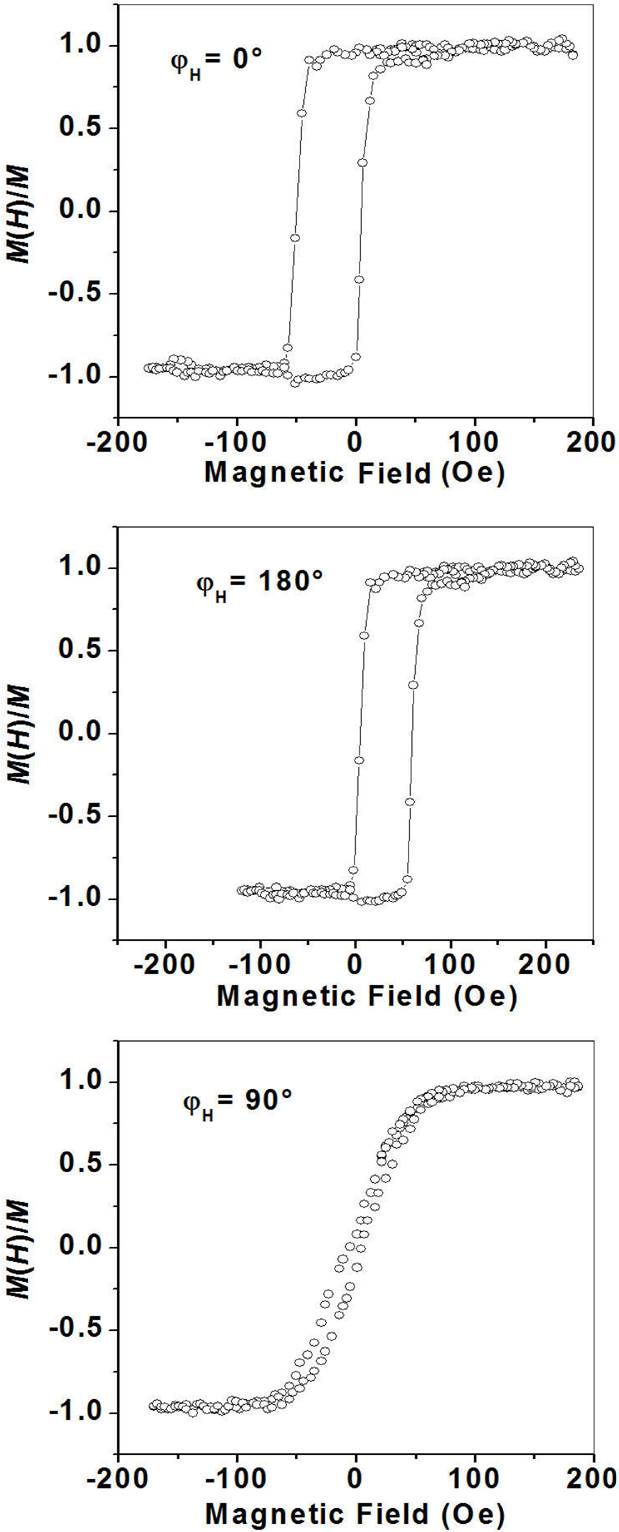
Figure 1. MOKE magnetization curves of NiFe(200 Å)/NiO (375 Å)/Si (001), obtained in three different orientations of the magnetic field.
Where K
u
is the uniaxial anisotropy constant in the FM, and K
eb
the unidirectional anisotropy constant. The equilibrium positions of the magnetization,
Or equivalently,
The exchange-bias field is obtained from Eq. (3) when H
0 equals the coercive field. In this case M = 0, and hence
From the magnetization curves the values of the exchange bias field, H
eb
, and the coercivity shift, H
C
, can be obtained as functions of the azimuthal angle,
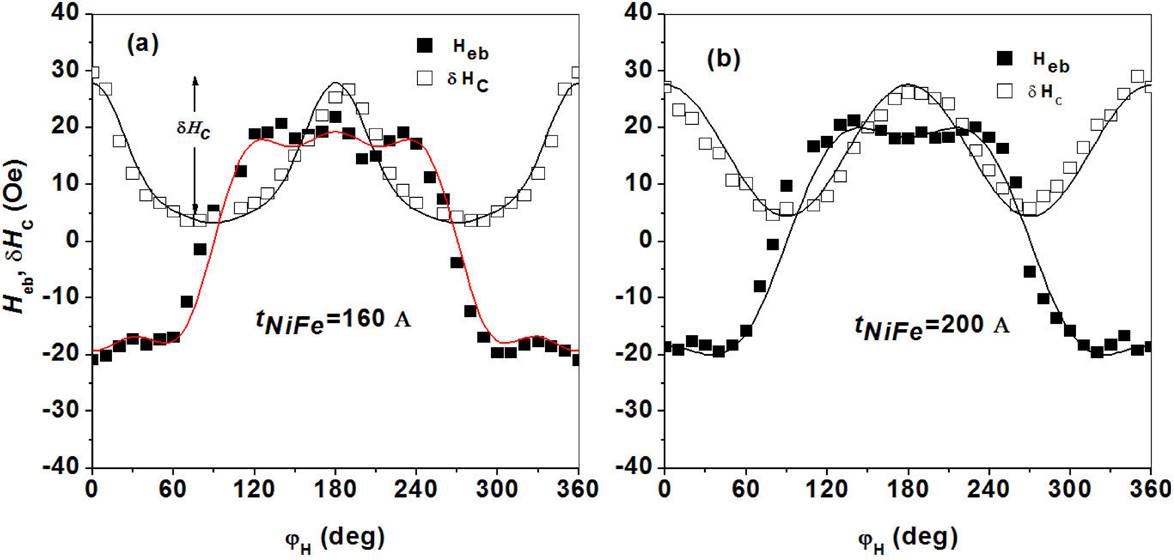
Figure 2. Angular dependece of coercivity, H C , and exchange bias, H eb , for (a) NiFe (160 Å)/NiO (375 Å), and (b) NiFe (200 Å)/NiO (375 Å). The solid curves are calculated from Eq. (6) using the coefficients listed in Table I.
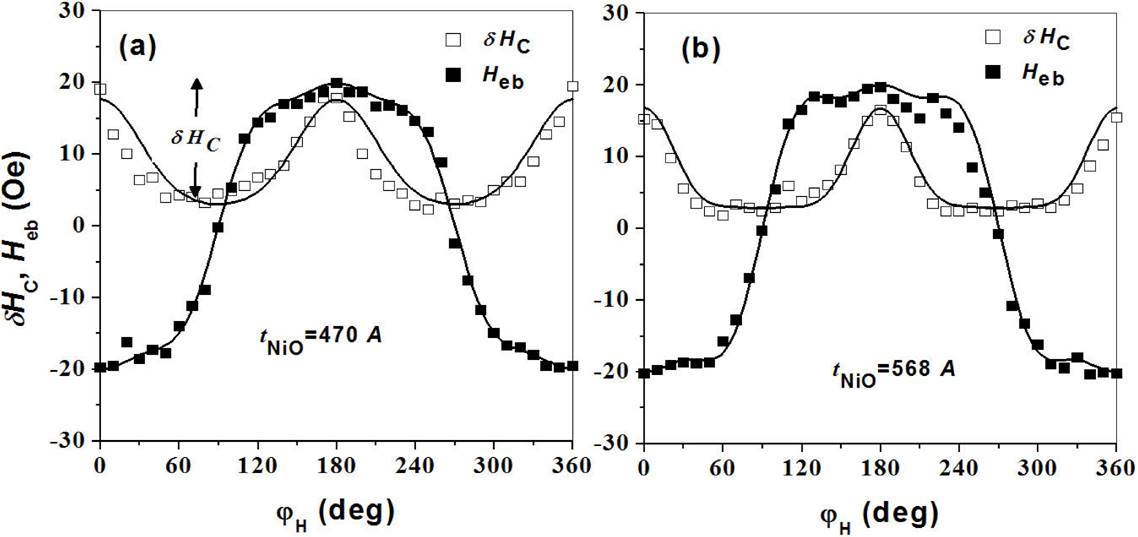
Figure 3. Angular dependence of coercivity, HC, and exchange bias, H eb , for (a) NiFe (200 Å)/NiO (470 Å), and (b) NiFe (200 Å)/NiO (568 Å). The solid curves are calculated from Eq. (6) using the coefficients listed in Table II.
with anisotropy field, H A ,
which after partitioned into even-n and odd-n terms, seems like
One immediately recognize that the first term is unidirectional and describes the additional symmetry in the exchange-bias field, while the second is uniaxial and takes account of the additional in-plane symmetry of the coercitivity. The solid curves in Figs. 2 and 3 are calculated by means of the unidirectional and uniaxial terms of Eq. (6), using the coefficients listed in Tables I and II, respectively. It is seen from Table I, that the symmetry of both H C and H eb turns simpler as the NiFe layer thickness increases, i.e., less anisotropy coefficients are needed to recover the additional in-plane symmetry. On the other hand, for varying NiO layer thickness the additional symmetry still present in all samples, with anisotropy coefficients of almost the same order. The values obtained from our analysis in NiFe/NiO are very close to those reported for other FM/AF bilayers, such as amorphous FeCoSiB/NiO bilayers 11. Numerical calculations show that the origin of these anisotropy coefficients can be related to the spin configuration at the FM/AF interface, and interface roughness 16.
An interesting property of an exchange-biased bilayer is that both the exchange-bias field shift and coercivity vary from sample to sample. This behavior gives suitable information about the interfacial nature of the magnetic anisotropies in the system. The thickness dependences of the maximum ¡field shift (measured at = 180°), and the amplitude of the coercivity curve (
4. Summary
NiFe/NiO exchange-biased FM/AF bilayers were deposited by DC-magnetron sputtering, onto commercial Si(100) wafers. The uniaxial and unidirectional anisotropies were then studied using Surface Magneto-optic Kerr Effect (SMOKE), as functions of the in-plane angle, and with respect to the NiFe and NiO layer thicknesses. The exchange bias and coercivity fields exhibit in-plane unidirectional and uniaxial symmetries, with angular dependences different from the simple











 text new page (beta)
text new page (beta)