1. Introduction
The Einstein’s special relativity plays a corner stone in modern physics. As stated in 1905 by Einstein, it is based on two postulates. The first postulate is about the constancy of the speed of light: the speed of light c is the same in all inertial frames of references. The second postulate is about the invariance form of the laws of physics under Lorentz transformations. As a consequence of this, any theory of space and time should be compatible with the theory of special relativity. There are some other aspects that were studied after the emergence of theory of relativity [1,2]. In Ref. [3], the Lorentz transformations were re-stated for an observer in a refracting but non-dispersive medium was proposed, and some physical consequences were discussed. In Ref. [4], Laue and Rosen theories of dielectric special relativity were derived, and argued that both are true but with different range of applicability. In Ref. [5], the non-local special relativity is introduced to overcome the difficulties accompanied the non-local electrodynamics problems.
In the last two decades, the fractional calculus approach to model or resolve various physical problems has attracted many researchers. There are a number of definitions or senses for fractional calculus such as Riemann-Liouville, Caputo, Riesz and Weyl [6-9]. The most important definitions are the Riemann-Liouville and Caputo definitions. These definitions have many applications in various fields [10-17]. The fractional derivative has lately been given a new definition. This is the first definition to use the limits definition, and it is called conformable fractional derivative (CFD) [18]. For a given function f(t) ∈ [0, ∞) → R, the conformable derivative of f(t) of order α, denoted as
This definition is simple in the sense that it meets the general properties and rules of the traditional derivative, whereas the other fractional derivatives do not satisfy them. From these properties the Leibniz, chain rules, and derivative of the quotient of two functions. Because of its ease of use, general features, and preservation of general properties including the locality property, the conformable derivative has a wide range of applications in a variety of fields of science.
In Refs. [19,20], this CFD is re-investigated and new properties similar to these in traditional calculus were derived and discussed. The CFD has been used to study various physical problems with possible nonlinear or diffusive nature. In Ref. [21], the mass spectroscopy of heavy mesons were investigated within the frame of conformable derivative searching for any ordering effect in their spectra that varies with the fractional order. In Ref. [22], the fractional dynamics of relativistic particles was studied, and it was found that fractional dynamics of such particles are described as non-Hamiltonian and dissipative. Possibility of being Hamiltonian system under some conditions was also presented. In Ref. [23], a new conformable fractional mechanics using the fractional addition was proposed and new definitions for the fractional velocity fractional acceleration are given. In Ref. [24], deformation of quantum mechanics due to the inclusion of conformable fractional derivative is presented and investigated with some physical illustrative examples. Recently, Pawar et.al. [25] introduced Riemannian geometry through using the conformable fractional derivative in Christoffel index symbols of the first and second kind. The conformable calculus has been used in making an extension of approximation methods to become applicable to conformable quantum mechanics [26-28], and to find solutions of related differential equations such as the conformable Laguerre and associated Laguerre equations [29]. In Ref. [30], the Hamiltonian for the conformable harmonic oscillator is constructed using fractional operators termed α-creation and α-annihilation operators.
Later, in Ref. [31], pointed out the conformable derivative is not fractional but it is an operator. Thus in the present paper we call it conformable derivative.
The purpose of this paper is to investigate the deformation of the theory of special relativity within the frame of conformable fractional derivative. This means that, we will adopt a new set of α-Lorentz transformations and use them to re-state the postulates of special relativity, and to verify the validity of the invariance principle to various laws or equations of physics.
2. Theory
Deformation of Lorentz transformations using conformable derivative is reported in Ref. [24].
Definition The α- Lorentz transformations between two inertial frames S and S’ are defined as [24]:
where
By adjusting the
We now state the two postulates of conformable special relativity as follows.
Postulate 1(Constancy of the speed of light): The speed of light is the same for all α - inertial frames of references.
Postulate 2(Invariance Principle): The laws of physics are invariant under α -Lorentz transformations.
The following subsections purpose is to clarify theses two postulates.
2.1 The α -velocity addition law
Following [23], we define the α -velocity of an event with respect to the S and S’ frames as
respectively. To calculate the velocity using Eqs. (2) and (3), we have
By interpreting
In case u x = c, we have
where we have made use of Eqs. (6) and (7). With the realization x/t = c and x’/t’ = c, we have
from which we obtain
or
This verifies that the α - Lorentz transformations proposed in Eqs. (2-5) leads to the constancy of the speed of light.
2.2 Conformable wave equation
Here, we verify the covariance of the wave equation under the
Using the
where
and then using the α - Lorentz transformations Eqs. (2) and (3),
Operating again on
From eqs. (4) and (5), it is clear that
and thus
Therefore,
Same procedure yields,
and
For the
Thus,
Substituting Eqs. (16),(18),(20) and (22) in Eq. (14), we obtain
Rearranging,
Using
or
which shows that the α - wave equation is invariant under the α - Lorentz transformations. In the following three subsections, we provide three examples that are in support of the second postulate.
2.3 Conformable Schrödinger equation
The conformable Schrödinger equation [24] is
In 3 + 1 -dimensions, we have
where
Thus, the conformable Schrödinger equation is not invariant under the α - Lorentz transformations.
2.4 Conformable Gordon-Klein equation
We firstly propose the following definition of conformable relativistic energy.
Definition The conformable relativistic energy is defined as
Quantization can be achieved by substituting for the conformable operators as
Substituting Eqs. (16), (18),(20) and (22) in Eq. (26), we have
Then,
Thus, we have
or,
Thus, the conformable Klein-Gordon equation is invariant under the α - Lorentz transformations.
2.5 Four vector in conformable form
We firstly present the definition of conformable position.
Definition.
1-The α - covariant notation for position
2- The α - contravariant notation for position
So, the relation between
where
Thus, the displacement in conformable four vector is given by
1- The α - covariant displacement
2- The α - contravariant displacement
The conformable differential line element is then given as
Secondly, we present the definition of operators in conformable four vector.
Definition. The dell operator in conformable four vector is defined as
1- The α - covariant dell operator is given by
2- The α - contravariant dell operator is given by
Thus, the α - D’Alembert operator is given by
So, using α - D’Alembert operator, the conformable wave equation and the conformable Klein-Gorden equation are
respectively. Thus, the energy-momentum four vector in conformable form can be obtained as follows:
1- In α - covariant form
2- In α - contravariant form
In case independent time of the conformable Schrodinger equation [24], we get
and
respectively, where
2.6 The α - Lorentz transformation in Minkowski Space
Minkowski space is the most popular mathematical framework on which special relativity is formulated, and it is strongly related with Einstein’s theories of special relativity and general relativity. It is also called Minkowski spacetime and it is a combination of three dimensional Euclidean space and time into a four-dimensional manifold [33].
The α -Lorentz transformation in Minkowski Space is given by
1- In the α - contravariant form
where
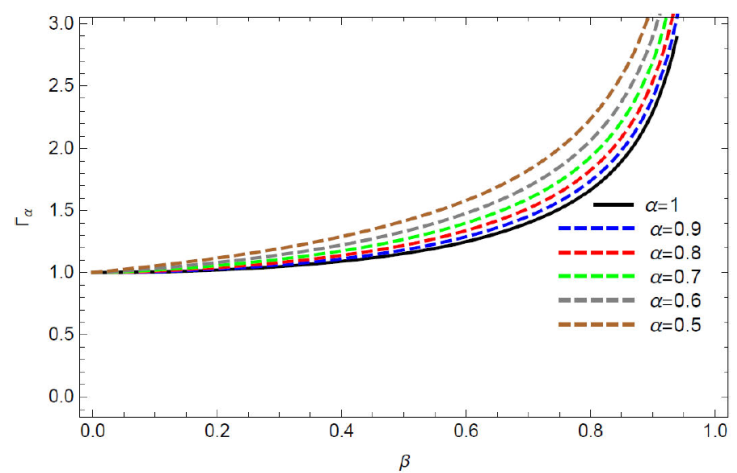
where β
α
= v
α
/c
α
and its inverse is
2-The α - covariant form
where
Proof. Using
we can write
Substituting in Eq. (47), yields
Then, we can write x θ as
Substituting in Eq. (49), we obtain
Thus,
Taking the inverse of
2.7 Conformable Dirac Equation
In Mozaffari et al., [34], the Dirac equation using the conformable derivative is investigated and it is introduced as
Where γ
μ
are the famous
However, when we do a Lorentz transformation, the wave function changes. Because the Dirac equation and Lorentz transformation are linear, we require that the transformation between Ψ and Ψ’ be linear too:
Where S denotes an x - independent matrix whose properties must be found. The Dirac equation in Lorentz covariance indicates that the γ matrices are identical in both frames. Using
From Eq. (55) we found
Substituting from Eq. (56) and then multiply with S from the left, yields
Comparing Eq. (58) with Eq. (54), we obtain
So,
Therefore, we demonstrated that the conformable Dirac equation is covariant in α -Lorentz transformation. For more information on the S matrix, one can refer to [35,36].
3. Summary and conclusions
In this paper, we have investigated the deformation of Einstein’s special relativity using the concept of conformable derivative. Within this frame, the α-Lorentz transformations were defined, and the two postulates of the theory were extended and re-stated. Then, the conformable addition of velocity laws were derived and used to verify the constancy of the speed of light for any fractional order α. The invariance principle of the laws of physics postulate was demonstrated for some typical illustrative partial differential equations of interest, namely, the conformable wave equation, the conformable Schrödinger equation, the conformable Klein-Gordon equation, and conformable Dirac equation. For a wave equation where time and space appeared with the same α-order, it is found that it is invariant under α-Lorentz transformations. Otherwise, it is not.











 nueva página del texto (beta)
nueva página del texto (beta)