Introduction
Mathematical figures have been employed to depict natural events and design objects that fit such behaviors. Leonardo of Pisa, better known as Fibonacci, described in his book Liber Abaci (The Book of Calculation) in 1202 AD the pillars of nature through a sequence meant to report the reproduction rate of rabbits. Referred to as the Fibonacci Series, the sequence involves an infinite chain of numbers determined by the sum of two consecutive integers, equal to the following: 0, 1, 1, 2, 3, 5, 8, 13, 21…1-3. Furthermore, the ratio of any two successive numbers approximates 1.618, a numerical value addressing the Greek letter Phi (φ), maintaining its accuracy as the numbers go higher. Phi correlates directly with the golden ratio (GR), a proportion that has been historically associated with the esthetic canon2.
As described by Euclid more than 2000 years ago, the GR exhibits the proportions of a line divided into two segments of unequal length4. The ratio between the shorter and longer segment is the same as the ratio between the length of the longer one and the total length of the line, noted as A/B = B/(A + B)3. It has been described in architecture, music patterns, art engineering, biological models, physics, and mathematical proportions1-7. The GR is rooted in nature as aesthetic perfection related to an adequate structure and function of living things that apply to the medical field. GR in the human body dictates the ear’s golden spiral8-10, anatomical proportions, and regulatory parameters of the heart rhythm, blood pressure, and pumping functions7 and can affect the visual processing according to aesthetic preference with Phi values10,11. The age of peak fertility and uterus measurements were equal to the GR10. The human genome itself consists of Fibonacci series sequences, showing it is established in DNA as in outer structures of the human body to maintain health and aesthetics10.
Notwithstanding, the vascular system is not an exception. The GR has been mainly studied in artery and heart dimensions, accounting for blood pressure values, valve figure designs, and vessel branching. In 2014, Yetkin et al. observed that systolic and diastolic blood pressure, and pulse pressure, follow the GR12. Chemla et al. demonstrated the relation of Phi with fluctuations between sPAP/mPAP and mPAP/dPAP (sPAP: systolic pulmonary artery pressure; dPAP: diastolic PAP; mPAP: mean PAP). In a further study, Yetkin et al. found that the increasing divergence of these ratios indicated more severe pulmonary hypertension4,13. GR applications have also been noticed in the correlation between embryological development and the size of heart cavities7, the structure and kinetics of mitral and aortic valves, heart rate and blood pressure at rest, and coronary anatomy14-17. These previously mentioned studies lead us to the hypothesis that the GR could influence the lower limb vein ratios associated with the course of the common femoral vein (CFV), femoral vein (FV), and popliteal vein (POPV) and even has a role in the early diagnosis of venous pathology. We aim to pioneer in describing the potential correlation of the GR within the diameters of veins in the deep venous system of the lower limbs and its possible applications.
Methods and materials
We performed a case series study with a sample of 43 patients between September 2020 and December 2020. Patients were healthy volunteers above 18 years of age, with no medical or familial records of chronic diseases including chronic venous disease, and no abnormalities in clinical examination. As general parameters, sex, height, weight, and leg dominance were registered. Diameters of CFV, FV, and POPV were measured using a Butterfly iQ+™ ultrasound system. We took measurements in a stand-up position with CFV ultrasound located in the inguinal ligament crease, FV in the first third of the tight, and POPV below the adductor hiatus. According to Hertzberg et al., the diameter of the standard deep leg veins was most significant at the level of the CFV (mean diameter, 10.5 mm). The diameter progressively decreased until the middle superficial FV and remained relatively constant (mean diameter, 6.4-6.8 mm) more caudally18.
We took the measurements of the venous diameters at the point of greatest narrowing. The ultrasound-guided approach was performed with the M-Turbo ultrasound system (SonoSite, Bothell, WA, USA) using a linear array vascular probe with a frequency of 6–13 MHz and a width of 6 cm (Fig. 1A and B). For patients with vein duplicity, the longest diameter was taken as the value registered. We used compression to confirm the vessel was venous. Two observers were in charge of data collection; none knew the standard measurements beforehand. Then, the volunteers filled out a 20-item questionnaire on leg dominance. The form was provided by a study by van Melick et al., translated from English to Spanish, and validated by an official translator19. The study’s lead author, van Melick, granted permission to integrate the questionnaire into the present study19. The questionnaire asks about situations carrying out both unilateral and bilateral mobilization tasks, which patients must answer according to the leg they prefer to use. We performed a descriptive analysis using measures of central tendency, location (percentiles), and dispersion (standard deviation and ranges) for continuous variables. Descriptive statistical analysis was done using Stata v16.0 (StataCorp LP. College Station, TX, USA).
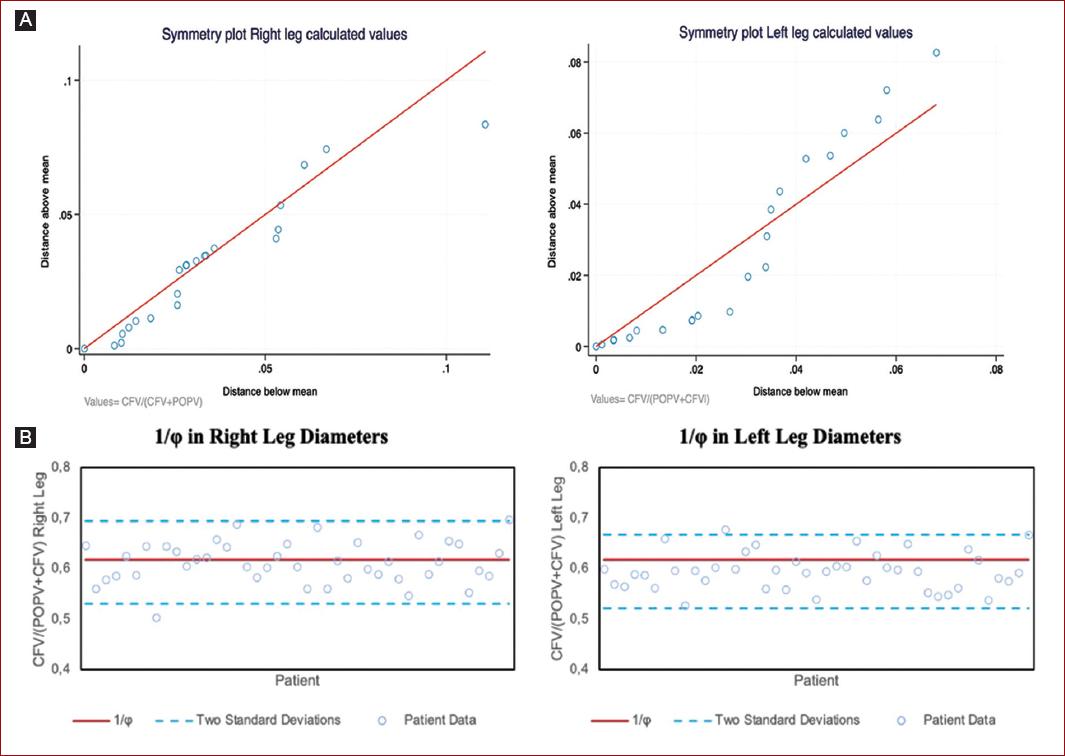
Figure 1 A: the scheme of the anterior deep venous system of the lower extremity. The red line represents the location of the ultrasound transducer. B: the scheme of the posterior deep venous system of the lower extremity. The red line represents the location of the ultrasound transducer.
Testing the GR in lower limb vein diameters
Since Phi is a value that has been intrinsically found in nature, especially in relationships between physical designs, we propose that vein diameters in the lower limb grow in size from distal to the proximal direction in a similar fashion. Therefore, the GR would be found descriptively as vein diameter values approach a venous bifurcation, more precisely in the deep venous system as superficial veins might have several genetic and epigenetic distortions. We compared vein diameters of CFV, FV, and POPV using the Golden Line formula A/B = B/(A + B), to find similarities between the solutions and GR-related figures; angle, triangle, spiral, and inverse (1/φ). The flexibility and importance of these corresponding numbers lie in that the GR allows replicating the same structure on different scales through its basic physical properties4. Moreover, we compared the greatest and most minor diameters for a closer relationship example. Between the ties of CFV and POPV diameters, values relative to 1/Phi were found:
Results
We obtained the findings shown in table 1 from the participant general data register. We found a mean calculated value of 0.611 for the right lower limb (A) and 0.59 for the left limb depicted (B) in figure 2 A and B, showing proximity between valuesand left and right mean. The closeness between values and 1/Phi between two standard deviations accounted for 95.4% of the data for the right (0.52-0.69; SD: 0.0409; SE: 0.0062) and left leg (0.52-0.66; SD: 0.0366; SE: 0.0055) value intervals cover 1/Phi is shown in figure 2 C and D except for data obtained by patients 8 and 43 for the right leg and 9, 13, and 43 for the left leg. Patient 8 had a left FV duplicity, patient 43 had a BMI of 29.3, and patients 9 and 13 had no other findings.
Table 1 Participant general data register
| IQR | ||
|---|---|---|
| Total participants | 43 | |
| Sex | Female 23 (53.50%) | Male (46.50%) |
| Average height (cm) | 169.49 | (162-176.5) |
| Average BMI | 21.88 | (19.37-24.49) |
| Dominance | Left 4 (9.30%) | Right 35 (81.39%) |
| Both 4 (9.30%) | ||
| Venous duplicity | 10 (23.25%) | |
| 4 (9.30%) of left FV | 3 (6.98%) right FV | |
| 2 (4.65%) left POPV | 1 (2.33%) right POPV | |
| Average vein diameters (cm) | 95% CI | |
| Right common femoral vein | 1.33 | (1.26-1.40) |
| Right femoral vein | 0.96 | (0.91-1.01) |
| Right popliteal vein | 0.84 | (0.79-0.88) |
| Left common femoral vein | 1.27 | (1.19-1.34) |
| Left femoral vein | 0.91 | (0.84-0.97) |
| Left popliteal vein | 0.87 | (0.81-0.92) |
| 1/Phi median value | Left leg 0.59 (99% CI: 0.57-0.60). | Right leg 1/Phi 0.611 (99% CI 0.59-0.62). |
| 1/Phi relation to data | Left (0.52-0.66; SD: 0.0366; SE: 0.0055). | Right (0.52-0.69; SD: 0.0409; SE: 0.0062). |
BMI: Body mass index, CI: Confidence interval, SD: Standard deviation, SE: Standard error, IQR: Interquartile range, FV: Femoral vein, POPV: Popliteal vein
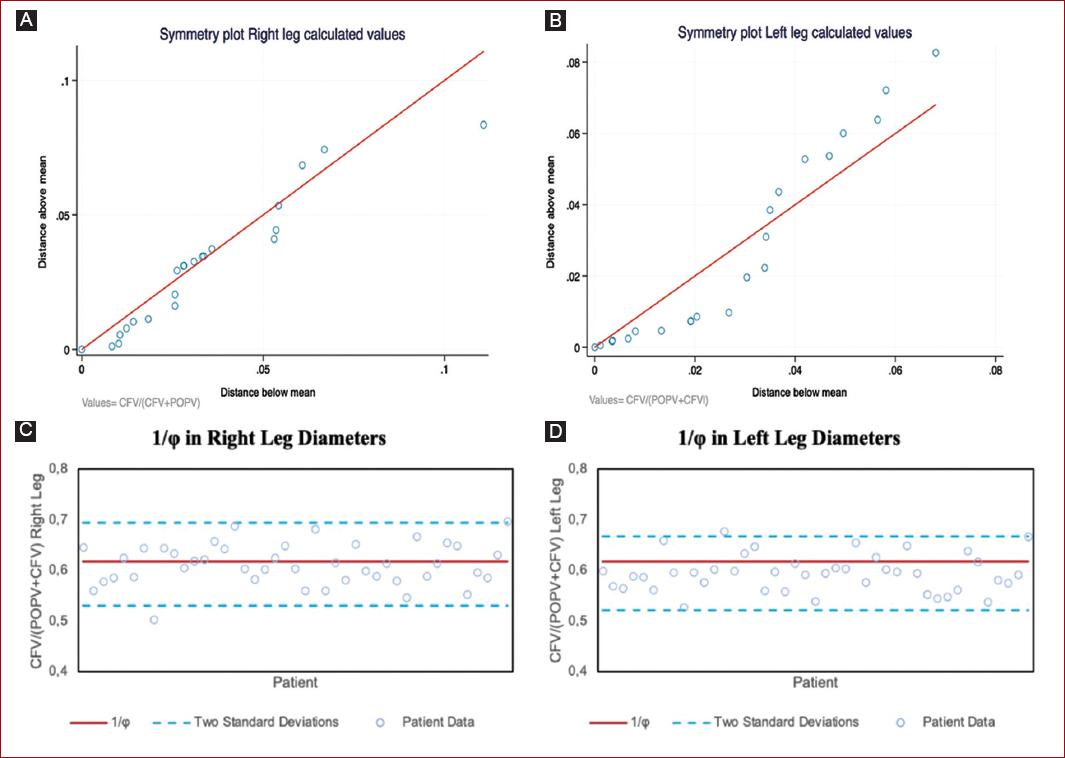
Figure 2 A:distribution between right leg data from common femoral vein (CFV)/(CFV + popliteal vein [POPV]) diameter relations and the mean of the right leg values (represented by the red line). B: distribution between left leg data from CFV/(CFV + POPV) diameter relations and the mean of the left leg values (red line). C y D: the proximity of data to 1/φ (red line) in between two standard deviations was calculated for the mean of each leg equation value (blue dotted line). C: the right leg diameters were submitted through the CFV/(CFV + POPV) formula, with a distribution between two standard deviations (0.52-0.69) from the mean (0.611), and relationship to 1/φ (0.618). D: the left leg diameters were submitted through the CFV/(CFV + POPV) formula, with a distribution between two standard deviations (0.66-0.69) from the mean (0.59), and relationship to 1/φ (0.618).
Discussion
Implementing the GR in vascular clinical practice could serve as a complementary diagnostic modality, particularly in the relatively early stage of venous disease, since an undiagnosed progression can lead to complications requiring more invasive treatments such as surgery20. As other authors state, the GR is a measure of harmony that has been applied to the anatomy of the human body and has been used in plastic surgery20-23. We first proposed a theoretical background to support the hypothesis that Phi may be a preferred number in the venous anatomy and could be associated with chronic venous disease. The main result of our study was a significant descriptive approximation of the right leg’s relationship between the diameter of CFV and POPV following the CFV/(CFV + POPV) formula.
In our sample, most left and right leg values were within two standard deviations from the media. The mean of the right limb was 0.611 cm, which is within the 99th confidence interval of 1/Phi (99% CI: 0.59-0.62). On the other hand, although left leg values were related to each other and are close to 1/Phi, they are not significant relative to the mean (99% CI: 0.57-0.60). One of the reasons we might think that the golden rule applies to the right side of the body can be explained by dominance since most of the participants were right-handed in our research. We did not find correlations between venous duplicity, leg dominance, sex, and IMC. However, values for patient 43 were outside SD for both legs (IMC = 29.3), implying a possible negative correlation for approaching Phi. Further investigation is required to quantify the significance of this finding. We tried to demonstrate a harmonic formation of the veins according to the golden number.
This number is often found in elements of nature and denotes the harmony of proportions. According to reported studies, Phi is in biology and esthetic beauty, resembling the perfect number that depicts the nature of several relations. Some of these studies have shown the likelihood of Phi being a relevant measure for arterial pressure relations in predicting pathologies such as pulmonary hypertension13.
Even though its correlation with venous pathology has not yet been studied, this study opens the hypothesis that patients with lower limb venous diameters closer to Phi could have less probability of developing venous insufficiency, among other venous pathologies regarding the deep venous system. We hypothesize that by implementing the GR in vascular surgery, it would be possible to screen or even identify venous alterations early, treat them timely, avoid the high economic cost to the health system and improve the patient’s quality of life. On the other hand, reconstructions of computed tomography angiography DICOM archives24,25 could further optimize the reported measures with the potential to improve the grafting techniques and control of thrombogenesis26 in DVT. Therefore, further research is required on this topic and the implications of using GR relations as standardized criteria.
Limitations
This study allowed the introduction of the GR hypothesis to the venous circulation. Nevertheless, the main limitation was the need for a more significant sample due to the lack of available volunteers during the COVID-19 pandemic, falling into a selection bias. In addition, the measures of vein diameters can be variable based on the equipment resolution and clinical experience, as they were physician dependent. We especially noticed this issue in FV recorded measures due to the disparity in the lower limb, even if we implemented a standardized position. We could not compare variables such as sex, leg dominance, and venous duplicity directly to GR but qualitatively, as they were categorical.
Conclusion
Deeper knowledge of GR impact proportion in the deep venous system of the lower limb is yet to be established. Nevertheless, this study demonstrated leg diameter relationships approached to 1/φ, evidencing once again that the GR is involved in physiological measures such as blood pressure and other related to anatomical measurements like the coronary arteries, heart valves, and as shown in this article, the venous system. Therefore, just as a role for the impairment in GR harmony has been seen in patients with pulmonary hypertension and arterial hypertension, these results may provide a new framework for deep vein pathology prevention by stating a normal range in GR measures versus patients with vein insufficiency. Regarding the correlation with Phi, we consider that further studies should be performed with a larger and more diverse population, including a healthy group of participants and a group with venous insufficiency, to explore the implications of leg dominance in leg diameter and the role of the lower limb venous duplicity in pathologic venous disease. We invite readers to further research for possible uses of GR as a diagnostic and interventional tool for deep venous disease complementary workup. Likewise, we extend the invitation to explore more anatomical areas and physiological relationships involved with this fascinating proportion of harmony, which may impact the care of our patients in the future.











 nueva página del texto (beta)
nueva página del texto (beta)



