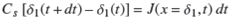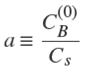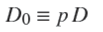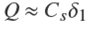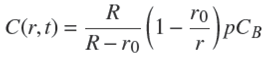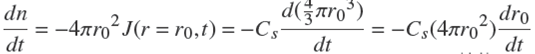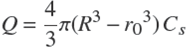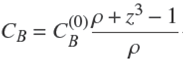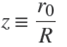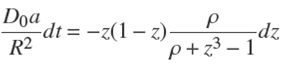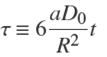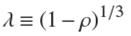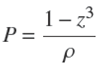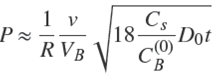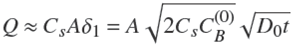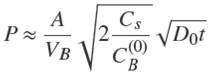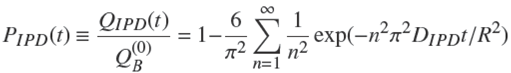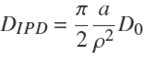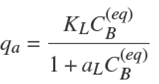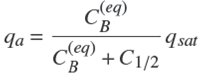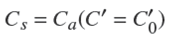2.1 Ingredients of the model

We consider an adsorbent medium (denoted by A) in contact with a perfectly stirred batch adsorber, denoted by B, containing all of the solute initially. The adsorbate diffuses freely in A until it is immobilized when it encounters a free site.

As shown in the literature (^{Ruthven, 1984}; ^{Grathwohl, 2012}), in the case of very low concentration of solute, the latter obeys a diffusion equation with an effective diffusivity, D_{e f f} = D(1 + α) (Ruthven, 1984; Grathwohl, 2012), in which D is the diffusivity of free solute and ? is the slope of the adsorption isotherm for very low concentration.

On the opposite, in the present work, the initial concentration in the batch adsorber will be sufficiently high so that the ash layer of the adsorbent operates in the plateau region of the adsorption isotherm. In this case, the degree of adsorption in the reacted zone will not vary appreciably in the course of time as long as the external concentration remains high enough.

In the model, the medium will be considered to possess a uniform concentration of sites, denoted by C_s , onto which the solute molecules form a monolayer. It will be supposed that the adsorption process is diffusion-controlled, i.e. the adsorption process is very fast compared to diffusion. The latter will be described by using an average diffusion coefficient in A, which will account for the various possible diffusion pathways in the medium (micropore, mesopore, macropore, and surface diffusion (^{Do, 1998})). The total amount of solute will be sufficiently high so that the ash layer thickness in A at equilibrium is not very small. So, the concentration of solute on the surface of A will be taken equal to that in the batch adsorber, denoted by C_B . The boundary condition for the solute concentration in A will be that the concentration of free solute is continuous at the mouth of a pore.

The classic quasi-steady state (QSS) approximation (^{Briggs and Haldane, 1925}; ^{Kramers, 1940}; ^{Crank, 1975}; ^{Simonin et al., 1991}) will be used to derive analytical results. Generally, the QSS regime does not hold at the very beginning of the process, but it settles after a short induction time.

2.2 Adsorption in a semi-infinite slab (1D case)

Before examining the spherical case, it is useful to first present shortly the model introduced by Crank (^{Crank, 1957}, 1975) in the one-dimensional (1D) case and solve it in the QSS approximation because this has not been done before in the literature and some results will then be used for the spherical case. Moreover this derivation gives more insight into the process.

We consider a semi-infinite porous slab of adsorbent that is put in contact at t = 0 with a very large bath of batch adsorber of constant concentration, CB(0) (see Fig. 1). When the solute diffuses in A in the B direction x perpendicular to the surface of A, it binds to free sites and progressively builds up a reacted zone in which all adsorption sites are occupied. We denote by δ1 the thickness of this ash layer in 1D.

Fig. 1 Sorption in a flat slab. 

The concentration of free solute, C(x,t), in this zone obeys Fick's second law which reads ∂C(x,t)/∂t = −∂J(x,t)/∂x = D∂² C(x,t)/∂x ², with t the time, D the diffusion coefficient of the adsorbate in A, and J the flux of solute molecules per unit cross-section area given by Fick's first law, J(x,t) = −D∂C(x,t)/∂x. It results from the definition of δ₁ that C = 0 at x = δ₁.

The variation of δ₁ in a time interval dt is given by the relation,

(1)

which expresses the fact that the variation of the number of occupied sites in the lapse of time dt is equal to the number of solute molecules arriving at the position x = δ₁ at time t. At this location, the adsorbent acts like a sink for the solute.

In the QSS approximation one has, ∂C(x,t)/∂t ≈ 0 in the ash layer (and C = 0 for x > δ₁). Then J(x,t) is approximately constant in space in this layer by virtue of Fick's second law, so that J = J(t). Consequently, the concentration profile is approximately linear in this zone because of Fick's first law. Because of the boundary condition, C = pCB(0) at x = 0⁺, J is therefore given by,

(2)

By combining Eqs. (1) and (2) one gets the equation,

(3)

which yields,

(4)

with

(5)

and

(6)

the effective diffusion coefficient in the porous adsorbent. In what follows it will be taken as a constant because the solute concentration will be very low (see Results and discussion section). The total uptake of solute by A (per unit cross-section area) is

(7)

because in practical applications one may neglect the concentration of free solute in A as compared to that of adsorbed solute. Therefore one gets from Eqs. (4)-(7),

(8)

We notice that in this relation Q varies as CB(0) It is not proportional to CB(0). This point is further discussed below in section 2.4.

2.3 Adsorption in spherical beads

We now consider sorbent spheres of radius R that are immersed at t = 0 in a well-stirred batch adsorber of initial concentration CB(0). In general, B the particles used in adsorption experiments may be represented by spheres with a good accuracy (^{Ruthven, 1984}). They will typically be made of a porous material like activated carbon.

In this section, we use the same type of model as in the 1D case. Its application to adsorption in spheres has been solved in the literature in the case of an infinitely large amount of solution, in which the adsorbate concentration remained constant (^{Crank, 1957}, 1975; ^{Levenspiel, 1999}). It is solved below in the case of a finite amount of adsorbate, whose bulk concentration varies with time.

Let us define the maximum site occupancy ratio of a sphere as the ratio of the total number of solute molecules to the total number of sites,

(9)

with v the volume of a sphere and VB the volume of batch adsorber per sphere (volume of the bath divided by the number of spheres).

A spherical bead is depicted in Fig. 2. We denote by r ₀ the position of the moving boundary delimiting the shrinking unreacted core, at which the concentration of free solute concentration vanishes, and by δ the thickness of the ash (reacted) shell.

Fig. 2  Sorption into a sphere. 

The free solute concentration at position r and time t,C(r,t),obeys Fick's law, ∂u/∂t=D∂² u/∂r ²,with u≡ rC(r,t) (^{Crank, 1975}). The QSS approximation in the sphere reads: ∂u/∂t ≈ 0. Then, using the latter relation and the boundary condition, C(r = R−,t) = pC_B , and solving Fick's law one obtains,

(10)

The relation for the time variation of r0, similar to EQ.(1), reads,

(11)

in which n is the number of moles of solute arriving at r0 and the flux per unit cross-section area is given by J = −D∂C/∂r.

It stems from Eqs. (10) and (11) after a simple manipulation that,

(12)

in which C_B is obtained from the conservation of total solute amount in the course of time: CB(0)VB=CBVB+Q, with Q the amount of adsorbed solute,

(13)

in which we also used Eq. (7). Then one gets,

(14)

in which we have used Eq. (9) and introduced the non-dimensional radius of the core,

(15)

Insertion of Eq. (14) into Eq. (12) gives the following separable differential equation obeyed by ɀ,

(16)

with a defined by Eq. (5). The two sides of this equation can be integrated separately (e.g. with the help of Maple), between the states (t = 0, _ɀ = 1) and (t,ɀ(t)). After some rearrangements of terms, one arrives at the following result,

(17)

in which

(18)

is a non-dimensional reduced time, and the parameter λ defined by,

(19)

has been introduced for convenience. In practice, one generally has ρ < 1. Then it is easy to show that the minimum value of ɀ satisfies the relation, ρ = 1 − ɀ ³ _min, from which one gets using Eq. (19),

(20)

which expresses the concrete meaning of the parameter ג.

The fractional uptake (proportion of solute immobilized in the sphere) is (^{Do, 1998}),

(21)

By using Eqs. (9), (13) and (15) in Eq. (21) one gets,

(22)

from which it stems that,

(23)

Therefore, τ can be expressed as a function of P and ρ alone by inserting Eqs. (19) and (23) into Eq. (17).

2.4 Formula at short times

For small diffusion times, P is small. In that case, one gets from Eq. (23) that ɀ ≈ 1 − ρP/3, which relation may be inserted into Eq. (17). A Taylor expansion of this expression for τ to the second order in powers of P leads to P≈3T /ρ, which may be rewritten using Eq. (18) as,

(24)

or, together with Eqs. (5) and (9),

(25)

In fact, Eq. (25) may be obtained in another way, by noting that for small contact times the solute is adsorbed in a shell of small thickness given by the formula in 1D (Eq. (4)) and area 4πR ², so that, Q ≈ C_s 4πR ² δ₁ , which together with Eq. (21) leads to Eq. (24) or (25).

Moreover, as a consequence, we notice that this treatment may be applied to particles of arbitrary, yet smooth, shape in the first moments of the process. Then, the amount of solute adsorbed in the particle is given by,

(26)

with A the area of the particle, and consequently,

(27)

An important result of this latter formula is that the fractional uptake, P, not only varies as t ^1/2. It is also noticed that P should decrease with CB(0) as 1/ CB(0) (for constant C_S ), which is not a trivial result. This is due to the fact that the adsorbed amount, Q (which increases with CB(0) as expected, see Eq. (26)), varies as CB(0), because the ash layer thickness, δ1, varies in this way (Eq. (4)). This property clearly distinguishes a diffusion-controlled adsorption process from a simple pure diffusion process, for which P is independent of CB(0).

2.5 Intraparticle diffusion equation

The classic intraparticle diffusion (IPD) equation (^{Boyd et al., 1947}; ^{Weber and Morris, 1963}; ^{Rudzinski and Plazinski, 2007}; ^{Wu et al., 2009}) in the case of a sphere is expressed as,

(28)

in which P_IPD is the fractional uptake of solute, Q_IPD is the uptake, QB(0) is the initial amount of solute in BQB(0)≡CB0VB, and D_IPD is an ad hoc diffusion coefficient. Eq. (28) was written in such a way that the uptake satisfies the two conditions: QIPDt=∞=QB(0).

It seems that this relation was first used by ^{Boyd et al. (1947}) to describe adsorption kinetics in zeolites. However, as said previously, it does not take into account explicitly the effect of adsorption. It is the solution to the problem of free diffusion of a solute from a batch adsorber of constant concentration into a sphere that does not possess adsorption sites (Eq. (6.20) of (^{Crank, 1975})).

At the beginning of the process, Eq. (28) may be approximated by the following equation (^{Crank, 1975}; ^{Rudzinski and Plazinski, 2007}),

(29)

In the literature, the IPD equation has generally been used in the latter form preferentially to the full formula, Eq. (28). It is worth noting that Eq. (29) coincides with the result from the present model, Eq. (24), that is P_IPD ≈ P at short times, provided that the diffusion coefficient appearing in the IPD equation satisfies the relation,

(30)

or, by using Eqs. (25) and (29),

(31)

This result shows that the IPD diffusion coefficient is in fact a lumped parameter which depends on the experimental conditions. In particular, within the present model, for a given type of sorbent particles and a given volume of bath per particle, this pseudo-diffusion coefficient is expected to be inversely proportional to CB(0). We note that a decrease of D with the initial bulk solute concentration has often been reported in the literature, e.g. in (^{Kumar et al., 2003}; ^{Rudzinski and Plazinski, 2007}, 2008).

WhenD_IPD is given by Eq.(30), then P ≈Pat short times, but PIPD becomes larger than P for longer times. It was observed that the maximum deviation grows with the value of ρ. It reaches 7.2% for ρ = 0.1, ca.13% for ρ=0.5 and ca. 20%forρ=0.9.

2.6 Application of the model to experiments

The value for the concentration of sites, Cs, to be taken in the model must be determined. To illustrate, let us assume that the adsorption isotherm of A may be parameterized using the Langmuir equation, which is generally written as,

(32)

with qa the amount of solute adsorbed in A per mass unit of A, CB(eq) the concentration of solute in the bath in equilibrium with the sorbent, and K_L and aL parameters that are characteristic of the adsorbent.

This relation may be rewritten in the following different form,

(33)

with q_sat = K_L /a_L the amount at saturation and C _1/2 = 1/a_L . This form expresses the fact that qa=qsat/2, for CBeq=C1/2 and q_a ~ q_sat when CBeq≫C1/2.

If the adsorption process is sufficiently fast, one may assume a local equilibrium in a pore between the free solute and the solute adsorbed on the wall of the pore. Then, Eq. (33) may be extended to express the space-averaged concentration of adsorbed solute at a position in a pore as,

(34)

in which C_sat is the space-averaged concentration of adsorbed solute in A at saturation and C′ is the real free solute concentration in the pore, C′ = C/p. If qsat is the mass of solute adsorbed per mass unit of adsorbent, then C_sat = q_satd, with d the apparent density of the adsorbent particles. In passing from Eq. (33) to Eq. (34), it is assumed that the concentration of free solute in the pores is equal to that in the external bath C'eq=CBeq, and the fact that the quantity of free solute may be neglected as compared to that of adsorbed solute.

If the initial concentration of solute in the batch adsorber, CB(0), is sufficiently larger than C _1/2, namely in the plateau region of the adsorption isotherm, then the concentration of free solute in A in the vicinity of the surface of A will also be much larger than C _1/2 in the first moments of the experiment. Accordingly, the local amount of adsorbed solute will be nearly constant in that zone according to Eq. (34) because C' >> C _1/2. Further away from the interface where the solute concentration drops to zero, the concentration of adsorbed solute will also become vanishingly small. However the flux of solute entering into a bead should be governed mainly by what happens near its surface, where the amount of adsorbed solute nearly does not vary with the distance. Therefore we may expect the present model to give satisfactory results when CB(0) ≫C1/2, and as long as C_B remains sufficiently larger than C _1/2.

In the present study, a natural choice for the effective value of C_S was the value of the adsorbed concentration of solute at equilibrium for a representative value of the solute concentration in the pores, C0, that is,

(35)

In wich C_α is given by Eq. (34). A sensible choice for C0´ is C'0=C'B0/2 for a description of P varying between 1 and 0. Of course, this choice is not critical if CB(0)≫C1/2, in which case one then has CS ~ C_sat for any value of C0´.

3.1 Description of experimental results

First, the accuracy of the analytical expressions derived assuming a QSS in the adsorbent was examined through comparison with results from finitedifference (FD) calculations in the case of constant external concentration. The FD algorithm was based on a diffusion-equilibration scheme used in previous work (^{Simonin et al., 1988}). The main result is that the QSS hypothesis is valid, and the analytical expressions are accurate, when a ≤ 0.1.

Then, the results obtained in this work were applied to describe experimental results. The variation of the kinetics with the initial bulk adsorbate concentration and the validity of the predictions found in the theoretical section were examined. Kinetic data were found for various adsorbents, including chitosan (^{Wu et al., 2001}), resin (^{Hosseini-Bandegharaei et al., 2010}), fly ash (^{Panday et al., 1985}), home made activated carbon (^{Demirbas et al., 2008}; ^{Periasamy and Namasivayam, 1996}; ^{Santhy and Selvapathy, 2006}) and commercial activated carbon (^{Kumar et al., 2003}; ^{Choy et al., 2004}; ^{Yang and Al-Duri, 2001}, ²⁰⁰⁵).

The present model is not supposed to be applicable to systems in which ion exchange is the mechanism of adsorption because this process involves electric coupling between the diffusing ions. This is what we found when we analyzed for instance the data about the adsorption of Pb(II) in an algae gel (^{Vilar et al., 2007}). It was observed that P varied approximately as t but it did not vary as 1/CB(0). Actually it exhibited a non-monotonous variation with CB(0).

On the other hand, satisfactory results were found in the case of experiments carried out with commercial carbon like Filtrasorb. Hereafter, we present an analysis of the data reported by ^{Choy et al. (Choy et al., 2004}), and by ^{Yang and Al-Duri (Yang and Al-Duri, 2001}) about the adsorption of two different dyes, acid yellow 117 and Cibacron reactive yellow F-3R (RY, molar mass ~ 712 g mol⁻¹⁾, respectively, in porous beads of activated carbon, Filtrasorb 400 (F400). The data of Yang and AlDuri were particularly interesting because the main physical-chemical characteristics of the beads have been reported. Besides these results with Filtrasorb carbon, we present in the next section the result obtained in the case of the adsorption of Cu(II) on chitosan (^{Wu et al., 2001}) at short times.

The numerical values for the experimental kinetic measurements were obtained by digitizing the figures presented in the references.

3.1.1 Analysis of data at short times: variation of P with time and CB0

Experimental data may be represented using the approximate expression, Eq. (24) or (27), at short times, when the adsorption rate P is typically smaller than ~0.3 or 0.4 (^{Rudzinski and Plazinski, 2007}, 2008).

It was first interesting to study the effect of varying the initial external concentration in (^{Choy et al., 2004}) and (^{Yang and Al-Duri, 2001}). In these two references the initial bulk concentrations of the adsorbate were appreciably larger than C _1/2. One gets from Table 3 of (Choy et al., 2004) that C _1/2 ≈ 4.6 ppm, which is indeed much smaller than all initial concentrations CB(0) that were in the range 50-200 ppm. In (Yang B and Al-Duri, 2001), the values for a_L and K_L in Eq. (32) give C _1/2 = 1/a_L ≈ 7.46 mg L⁻¹, which is much smaller than the values for CB(0) that ranged from ~ 34 mgL to 131 mg L⁻¹ .

In the experiments of (^{Choy et al., 2004}), most of the values of the fractional uptake did not exceed 0.4, so that the approximate expression for P (Eq. (27)) was sufficient to analyze nearly all of the data. In (^{Yang and Al-Duri, 2001}), the fractional uptake nearly reached its equilibrium value at the end of the experiments. Next, these data were analyzed here at short times and, besides, in the whole time range.

The results for the analysis of the fractional uptake P as a function of the square root of time are shown in Fig. 3 and Fig. 4.

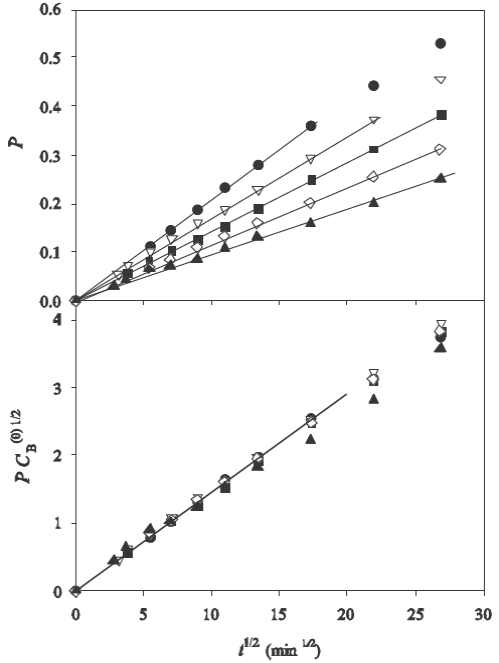
Fig. 3  Sorption rates as a function of time in rates as a function of time in the experiments of (^{Choy et al., 2004}) for various initial concentrations of solute in the bath. Upper plot: experimental values of P vs. t ^1/2; bottom plot: experimental values of PCB(0) (in ppm ^1/2 vs. t ^1/2. Symbols: (∙) CB(0)=50 ppm, (▽) 75 ppm, (■) 100 ppm, (◊) 150 ppm and (▲) 200 ppm. Lines: linear adjustments of points at times (for P < 0.4). 

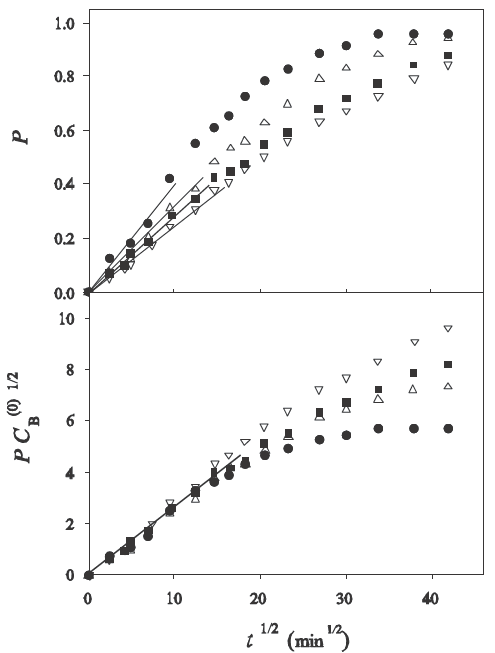
Fig. 4   Sorption rates as a function of time in the experiments of (^{Yang and Al-Duri, 2001}) for various the experiments of (^{Choy et al., 2004}) for various initial concentrations of solute in the bath. Upper plot: experimental values of PCB(0) (in mg ^1/2 L ^-1/2 vs. t ^1/2. Symbols: (∙) CB(0) = 35.4 mg L^-1, (△) 60.3 mg L^-1, (■) 87.2 mg L^-1and (▽) 131 mg L^-1. Lines: linear adjustments of points at short times. 

The upper frames show the variation of P as a function of t. The straight lines drawn for P smaller than ≈0.4 materialize the result of using the IPD equation at short times (Eq. (28)). These lines have different slopes so that the value of the IPD pseudo-diffusion coefficient, D_IPD , is observed to depend on the initial solute concentration in the batch adsorber, CB(0). In Fig. 4, D_IPD is found to decrease monotonously from ≈ 1.3 × 10⁻¹³ m² s⁻¹ for the lower concentration CB(0) =35.4 mg L⁻¹⁾, to ≈ 0.61 × 10⁻¹³ m²s⁻¹ (for CB(0) =131 mg L⁻¹⁾.

The bottom frames show the plots of the function PCB(0) as a function of t. According to Eq. (27), this function is expected to be independent of if Cs is constant, which was the case for all val|ues of CB(0) because CB(0)≫C1/2. It is observed in the bottom frames of Fig. 3 and Fig. 4 that the experimental points for PCB(0) fall on a common straight line. So this result definitely points to a diffusion-controlled adsorption process in these experiments.

Besides these findings in the case of a commercial activated carbon (Filtrasorb), experimental data were analyzed in the case of other materials, spanning a wide range of viable sorbent types, namely for dyes and Cu(II) in home-made activated carbons (^{Demirbas et al., 2008}; ^{Santhy and Selvapathy, 2006}; ^{Periasamy and Namasivayam, 1996}); and for Cu (II) in a resin impregnated with an extracting (Hosseini-Bandegharaei et al, 2001), and in fly ash (^{Panday et al, 1985}). Inmost cases, the fractional uptake was found to vary as t (or as t+A, with A a constant) at short times (for P ≤ 0.4) and to decrease with CB(0), but it did not accurately vary as 1/CB(0)

However, the case of the data for Cu(II) in chitosan (^{Wu et al., 2001}) gave an interesting result, which is shown in Fig. 5. The mechanism proposed in this reference (Wu et al., 2001) for the adsorption of Cu(II) was the chelation by unprotonated amino groups of chitosan, without ion exchange.

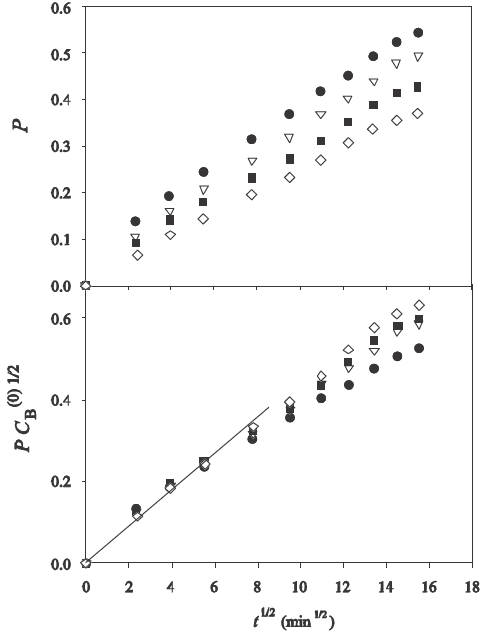
Fig. 5   Sorption rates as a function of time in the experiments of (^{Wu et al., 2001}) for various initial concentrations of solute in the bath. Upper plot: experimental values of P vs. t ^1/2; bottom plot: values of PCB(0) (in mol^1/2 m^-3/2) vs. t ^1/2. Symbols: (•) CB(0) = 0.93 mol m^-3, (▽) 1.40 mol m^-3, (■) 1.95 mol m^-3 and (◊) 2.92 mol m^-3. Lines: linear adjustments of points at short times. 

It is seen in this figure that the experimental points for PCB(0) are fairly aligned on a common straight line. This result strongly suggests that the adsorption of Cu(II) was limited by diffusion in this medium.

3.1.2 Analysis of data in the whole time range

The experimental results of (^{Yang and Al-Duri, 2001}) were also described in the whole time range by using the full equation, Eq. (17), together with Eqs. (19) and (23). For this purpose, the values of α and ρ were determined as follows. One gets the value of qsat in Eq. (33): q_sat = KL/aL ≈ 199 mg g⁻¹, from which one obtains the value of Csat in Eq. (34): Csat = 199 g L⁻¹ (with d = 1 g mL⁻¹ (^{Chang et al., 2004})). For each initial concentration CB(0) , the value of Cs (Eq. (35)) with C0´=CB0/2 was employed to compute the values of a and ρ from Eqs. (5) and (9), respectively. The set of values for a and ρ is collected in Table 1. It is observed that a is always very small, smaller than 10⁻³, so that the present model could be used because a≪ 0.1, as found from FD calculations.

Table 1   Values of input parameters in the model (in Eq. 17) for the data of (^{Yang and Al-Duri, 2001}) for RY of molar mass ~ 712 g mol^-1, and for which R=0.0536 cm and C_sat= 279.6 mol m^-3. 

Eq. (17), together with Eqs. (19) and (23), was used to calculate the concentration of solute remaining in the batch adsorber, C_B , as a function of time for various initial bulk concentrations. The value of D0 was optimized 'manually' by plotting the experimental points together with the results from the model. The plots for CB are shown in Fig. 6 for a common value, D ₀ = 5.83×10⁻¹² m² s⁻¹ or D = 1.08×10⁻¹¹ m² s⁻¹ because of Eq. (6) with the value for the porosity, p1/=2 0.54 (^{Chang et al., 2004}). We are not aware of any direct true diffusion coefficient measurements in porous carbon. However, some diffusivity values have been reported recently, that were obtained from the use of true diffusional models (not from the IPD equation): D of the order of 3 × 10⁻¹⁰ m² s⁻¹ for the fluoride ion in bone char (^{Leyva-Ramos et al., 2010}); of a few 10⁻¹¹ m² s⁻¹ for pyridine in activated carbon (^{Leyva-Ramos et al., 2015}) and for Rhodamine B in a zeolite (^{Castillo-Araiza et al., 2015}); and D in the range from 0.5 × 10⁻¹² m² s⁻¹ to 27 × 10⁻¹² m² s⁻¹ for various organic compounds in activated carbon (^{Leyva-Ramos et al., 2012}). Our value D = 1.08 × 10⁻¹¹ m² s⁻¹ therefore falls in the range of values obtained in these references. Besides, the diffusivity value of RY in bulk water should be of the order of a few 10⁻¹⁰ m² s⁻¹, as found by using the Stokes-Einstein formula (D = k_BT /6πηR) at 25°C with a radius of the order of 8-10 Å for the solute molecule. Then, it is plausible that this value may be reduced by a factor of several tens because of effects due to tortuosity, and to confinement in the micropores which constitute the major part of the pores in F400 carbon (Chang et al., 2004).

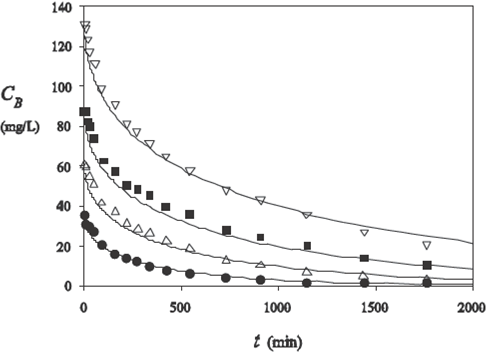
Figure 6   Concentration of solute remaining in the batch adsorber as a function of time in the experiment of (^{Yang and Al-Duri, 2001}) for several values of the initial concentration and a diameter of 0.0536 cm. Symbols: (∙) CB(0)= 35.4 mg L^-1, (△) 60.3 mg L^-1, (■) 87.2 mg L^-1 and ()131 mg L^-1. Solid lines: results for CB obtained using Eqs. (17) and (22) with D₀ = 5.83 x 10^-12 m² s^-1 (see text). 

It is seen in Fig. 6 that the model gives results in rather good agreement with experiments for the 4 initial concentrations, with C'0= CB(0)/2, in view of the asphericity and polydispersity of the beads used in these experiments (^{Yang and Al-Duri, 2001}). For the higher concentration of 131 mg L⁻¹, the agreement is satisfactory down to C_B ≈ 36 mg L⁻¹, which is ca. 3.6 times smaller than the initial value. For the lower concentration of 35.4 mg L⁻¹, the model gives a result for C_B that is in keeping with experiment at all times, even when CB falls down to concentrations of the order of C _1/2.

The agreement with experiment is a little less accurate than that reported in (^{Yang and Al-Duri, 2001}) in the framework of the branched pore diffusion model. However, this latter treatment involved 4 adjustable parameters instead of only one here (D).