Nota técnica

 

A general sectional volume equation for classical geometries of tree stem

 

Una ecuación general para el volumen de la sección de las geometrías clásicas del tronco de los árboles

 

Gildardo Cruz de León¹

 

¹ Facultad de Ingeniería en Tecnología de la Madera. Universidad Michoacana de San Nicolás de Hidalgo. Apartado Postal 580, 58000. Morelia Michoacán, México. Phone: (443) 326 03 79 E–mail: gcruzl@umich.mx.

 

Manuscrito recibido el 31 de julio de 2009
Aceptado el 18 de enero de 2010

 

ABSTRACT

This work refers to the classical theory of tree stem form. It shows the derivation of a general sectional volume equation for frustums of solids of revolution generated by the function y₂= p_nxⁿ where, p_n is a positive constant, and nany positive integer. The cylinder case presents a singular situation because of its sectional volume equation cannot be defined for n = 0 as it is known for the generating function. However, that geometry is implicit as a trivial solution of the derived equation. The known sectional volume equations for frustums of paraboloid, conoid and neiloid are particular cases of that equation for n =1, 2, and 3, respectively. The general sectional volume equation has an unexpected statistical nature. It is given as an arithmetic mean of geometric means The classical theory of tree stem form continue being present in the forest measurement teaching and research. This work could contribute to improve the understanding on that theory.

keywords: Dendrometry, applied mathematics.

 

RESUMEN

Este trabajo se refiere a la teoría clásica de la forma del tronco del árbol. Se muestra la derivación de una ecuación de volumen general de la sección de sólidos de revolución truncados generada por la función y₂= p_nxⁿ donde, p_n es una constante positiva, y n un entero positivo. El caso del cilindro constituye un caso singular pues su ecuación de volumen de la sección no se puede definir para n = 0, ya que es conocido por la función generadora. Sin embargo, esa geometría está implícita como una solución trivial de la ecuación derivada. Las ecuaciones conocidas de volumen de secciones truncadas de paraboloides, conoides y neiloides son casos particulares de la ecuación para n = 1, 2 y 3, respectivamente. La ecuación general de volumen de la sección es de una naturaleza estadística inesperada. Se da como una media aritmética de medias geométricas. La teoría clásica de la forma del árbol sigue estando presente en la enseñanza de medición e investigación forestal. Este trabajo podría contribuir a mejorar la comprensión de esa teoría.

Palabras clave: Dendrometría, matemáticas aplicadas.

 

INTRODUCTION

Tree stem and log volume estimations are some of the main goals in forest measurements. It is a difficult task because those volumes depend on their geometry (Brack, 1999). For more than a century researchers have been working extensively on the problem of tree stem geometry from fundamental and empirical points of view. In the first case one line of assessment has been by means of mechanical theories. As quoted by Larson (1963) and Dean and Long (1986), assuming that tree stem develops a particular form to maintain the bending stress constant under the influence of wind, in 1893, Metzger found a stem geometry where the cube diameter is proportional to height. Regarding that gravity determines tree stem shape the square diameter results proportional to the cube of height (McMahon, 1973). The empirical point of view can be divided on classical and current theories. In the classical theory a tree stem is modeled by parts using simple geometries of solids of revolution (Graves, 1906). Current theories suggest particular geometric models developed for each species by means of taper equations for the whole tree stem (West, 2004).

The classical theory of tree stem form has a significant position in the forest measurement literature. At least for a century it has been included in standard books of that field (Graves, 1906; Van Laar and Akça, 2007). The classical theory is considered as part of old research in forest measurements (West, 2004). However, it continues being a useful reference in research problems that depends on tree stem geometry. For instance, it has been used to derive the equation for the centroid position in the development of the centroid sampling method for tree stem volume estimation (Wood et al., 1990) and to derive a general relation between stem volume and stem surface form–factors independent of position (Inoue, 2006).

This work shows the derivation of a general sectional volume equation for the classical tree stem geometries. It is based on the classical theory of stem form, sectional methods of volume estimation, elementary algebra, and calculus. A summary of that methodology is provided below.

 

MODELS AND METHODS

Classical geometries of tree stem

Tree stem form can be modeled by longitudinal sections using elementary geometries of solids of revolution generated by the equation

where p_n and n are constants such that p_n>0 and n≥0 (Graves, 1906). Currently, only the cases n = 0, 1, 2, and 3, related to cylinder, conoid, paraboloid, and neiloid, respectively, are assigned the classical tree stem geometries (Diéguez–Aranda et al., 2003). For this work, the original conception of equation [1] will represent the classical geometries of tree stem and the study refers to any positive integer n.

 

Tree stem volume estimation

Different types of methods to estimate tree stem volume are used in forest measurements. Some of those methods consist in: i) to propose a taper equation and then to estimate the volume by integral calculus, ii) to propose a volume equation where the volume can be directly obtained as a function of height, normal diameter, and total height, and iii) to use a sectional method where the volume can be known for longitudinal sections as function of cross sectional areas and lengths (Brack, 1999).

The problem of volume estimation is critical when the volume of logs needs to be estimated at field where no information of the original tree stem standard parameters or geometry is at hand. For those common situations the use of sectional methods is unavoidable (Bruce, 1982).

 

General definition of sectional methods

For simplicity, tree stem longitudinal sections and logs will be called here tree stem segments.

Any sectional method to estimate the volume of a tree stem segment, of length L, is refereed to the volume of a cylinder and can be written as

where 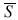is an average cross sectional area (Avery and Burkhart, 2002). What defines a particular sectional method is the form of S as function of selected cross sectional areas. Equation [2] will be essential in this work.

 

Standard sectional methods

The generally accepted sectional methods for volume estimation of tree stem segments in the forest measurement literature are the Huber, Smalian, and Newton methods. Following the notation of Chapman and Meyer (1949), for a tree stem segment, of length L, end cross sectional areas, B at the large end, b at the small end, and B_1/2 at the middle, those standard sectional methods can be respectively defined by

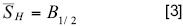
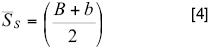
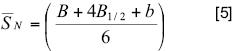
Then, V_H = 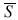_HL, V_S = 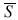_SL, and V_N = 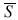_NL, are their corresponding volumes in agreement to equation [2].

 

Definition of systems and parameters for this work

Usually, solids of revolution generated by equation [1] are placed in a x y z Cartesian coordinate system with their axis of rotation along the x axis and their tips at the origin (Diéguez–Aranda et al., 2003). The systems for this work are defined as frustums of length L of those solids of revolution for any positive integer n. The end cross sectional areas will be called here, s for the small end, at x = x₁, and S for the large end, at x = x₂. By definition, x₁<x₂, then, L = x₂–x₁.

 

Sectional volume equations for the tree stem classical forms

The volumes for frustums of solids of revolution generated by equation [1], for n=0, 1, 2, and 3, as function of end cross sectional areas and length, are

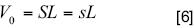
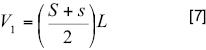
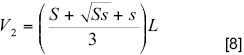
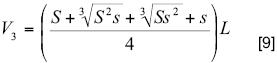
for cylinder, paraboloid, conoid, and neiloid, respectively (Graves, 1906).

In agreement to equation [2] S = S_n can be defined for equations [6]–[9]. For cylinder, S = S₀ = S = s. For frustums of paraboloid, conoid and neiloid, their mean cross sectional areas are respectively

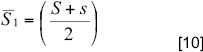
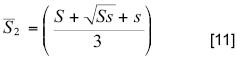
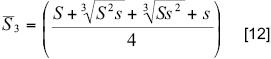
In the following, it will be shown that equations [7]–[9] are particular cases of a general sectional volume equation related to the generating equation [1] for any positive integer. The case n=0 deserves a particular discussion given at the end.

 

RESULTS

Derivation of a general sectional volume equation for classical geometries of tree stem

The main result of this work is shown in the form of a mathematical theorem.

Theorem

If V_n is the volume for a solid of revolution's frustum, of length L, and end cross sectional areas s at x = x₁, and S at x = x₂, generated by the equation , where n is a positive integer, then, V_n can be expressed in the form

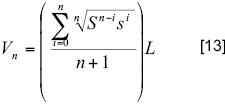
Proof

If the generating function for a solid of revolution is given by then the volume for its frustum from x = x₁ to x = x₂, given by integral calculus, is

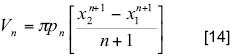
(Stewart, 2002). The relation

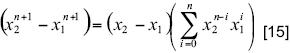
is well known in the mathematical literature (Spiegel and Moyer, 2007). Given that L = x₂ – x₁ and, from equation [1],

equation [15] takes the form

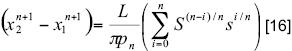
Substituting equation [16] in equation [14], results

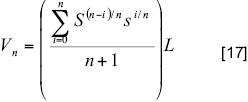
which is exactly equation [13].

 

DISCUSSION

The general sectional volume equation [13] has been derived for solids of revolution generated by the equation for any positive integer n. It can be easily shown that, for n =1, 2, and 3, the sectional volume equations [7]–[9] for frustums of paraboloid, conoid and neilod, are respectively recovered. Also, for s=0, basal area S, and L=H, their total volumes, SH/(n+1), are obtained. However, as it can be seen in equation [13] a sectional volume equation for the cylinder case n=0 cannot be defined. Nevertheless, it is not necessary because the general equation reduces to the cylinder volume equation when s=S for any n. That condition of cylinder volume recovery seems to be necessary for any sectional volume equation. In a proposal to evaluate various sectional methods to estimate butt log volumes Bruce (1982) takes that condition as a guide to select them.

Let us understand the meaning of equation [13]. In agreement to equation [2] it can be written in the form V_n = S_nL where

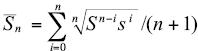
should correspond to a general mean cross sectional area. In particular, for equation [10], 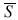₁, is the arithmetic average of the end cross sectional areas s and S. For equation [11], 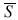₂ is the arithmetic average of end cross sectional areas, S, s, and their geometric mean SS (Uranga–Valencia, 2008). In general, if X₁, X₂, ... X_n, are the possible values taken by a variable X, for a sample of size n, their geometric mean is a measure of central tendency defined by (Chapman and Meyer, 1949; Van Laar and Akça, 2007).

The terms 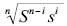for i=0,1,2,..., n, represent the geometric mean cross sectional areas for, X₁ = X₂ = ... = X_n–i = S and X_n–i+2. X_n–i+2 = X_n–i+i = X_n = s. Then, the general mean cross sectional area

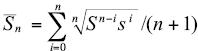
corresponds to the arithmetic average of those geometric means. Although the problem for this work was not originally defined as of statistical nature it turned on a statistical one whose complete analysis is in progress.

An additional property of equation [13] is that it represents a symmetrical function on s and S, for any n, because of

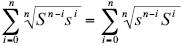
what means that segment volume is independent of its orientation along its axis of rotation. Also, the equations for the standard sectional methods obey that symmetry property. That condition could be another feature to take in account in the proposal of new sectional methods for volume estimation.

The most important contribution of this work is the general sectional volume equation [13] from what known results of the forest measurements field, since more than a century (Graves, 1906), are particular cases.

 

ACKNOWLEDGEMENTS

The author deeply acknowledges Professor Adrian Goodwin for his helpful suggestions. This work was supported by Coordinación de la Investigación Científica de la Universidad Michoacana de San Nicolás de Hidalgo. Morelia Michoacán, México.

 

REFERENCES

Avery, T.E. and Burkhart, H.E. 2002. Forest Measurements. Mc Graw Hill. USA.         [ Links ]

Brack, C. 1999. Forest Measurement and Modeling [online]. Available from sres–associated.anu.edu.au/mensuration/volume.htm.         [ Links ]

Bruce, D. 1982. Butt Log Volume Estimators. Forest Sci. 28(3):489–503.         [ Links ]

Chapman, H.H. and Meyer, W.H. 1949. Forest Mensuration. McGraw–Hill. Edition. USA.         [ Links ]

Dean, T.J. and Long, J.N. 1986. Validity of Constant–stress and Elastic–instability Principles of Stem Formation in Pinus contorta and Trifolium pratense. Annals of Botany. 58:833–840.         [ Links ]

Diéguez–Aranda, U., Barrio–Anta, M., Castedo–Dorado, F., Ruíz–González, A.D., Álvarez–Taboada, M.F., Álvarez–González, J.G. y Rojo–Albareca, A. 2003. Dendrometría. Mundi–Prensa. Madrid, España.         [ Links ]

Graves, H.S. 1906. Forest Mensuration. John Wiley & Sons. USA.         [ Links ]

Inoue, A. 2006. A model for the relationship between form–factors for stem volume and those for stem surface area in coniferous species. J. For. Res. 11:289–294.         [ Links ]

Larson, P.R. 1963. Stem Form Development of Forest Trees. For. Sci. Mon. 5.         [ Links ]

McMahon, T 1973. Size and Shape in Biology. Science. 179: 1201–1204.         [ Links ]

Spiegel, M.R. and Moyer, R.E. 2007. Algebra Superior. McGraw–Hill. México.         [ Links ]

Stewart, J. 2002. Cálculo Trascendentes Tempranas. Thompson Learning. México.         [ Links ]

Uranga–Valencia, L.P 2008. Aplicación del Modelo Cónico Segmentado a los Tipos Dendrométricos Clásicos como Función del Número de Segmentos. M.Sc. thesis, Facultad de Ingeniería en Tecnología de la Madera, Universidad Michoacána de San Nicolás de Hidalgo, Morelia Michoacán, México.         [ Links ]

Van Laar, A. and Akça, A. 2007. Forest Mensuration. Springer. Netherlands.         [ Links ]

West, P.W. 2004. Tree and Forest Measurement. Springer–Verlag. Berlín.         [ Links ]

Wood, G.B., Wiant, H. V. Jr., Loy, R.J. and Miles, J.A. 1990. Centroid Sampling: A variant of importance sampling for estimating the volume of sample trees of radiate pine. Forest Ecology and Management. 36:233–243.         [ Links ]

 

Nota

Este documento se debe citar como: Cruz de León, G. 2010. A general sectional volume equation for classical geometries of tree stem. Madera y Bosques 16(2):89–94.