First,1 I outline the differences and similarities between the geometrical theorem found in Metaphysics 9, 1051a24-27, which I will name “[1],” and Euclid´s theorem Proposition 32 of book I, to establish that they are not the same and to argue that Aristotle wrote [1] with a pedagogical-philosophical aim. Then, I cite two modern reconstructions of theorem [1] and determine which one is correct. Later, I advance a premise (A1) for [1] to assert that, for Aristotle, the deductive methodology and the previous knowledge of premise A1 are sufficient to discover a concrete mathematical-scientific knowledge without having to modify [1] or add other instruction(s) to it. Then I will briefly analyze the actuality and potentiality of mathematical-scientific knowledge in order to differentiate its nature from that of mathematical issues that do not accept the actuality-potentiality change.
This will allow me, opposing some academic consensus, to conclude that for Aristotle, theorem [1] is enough, as we found it in Metaphysics 9, 1051a24-27 (and against the suggestion of emending and/or enlarging the passage), to actualize the universal mathematical knowledge stating that the angles of all triangles are equal to two right angles. (I understand Aristotelian universal knowledge, following Ian Mueller, as a conceptual knowledge that “can be formulated syllogistically” (1970, p. 171), that can be discovered syllogistically, and that is valid in all places and circumstances.)
The theorems
Aristotle asserts that if all geometrical theorems (τὰ διαγράμματα; Met. 9, 1051a22) were in actuality, discoveries in geometry would not be possible. To prove his position, he describes a particular geometry theorem in Met. 9, 1051a24-27: “Why is the triangle two right angles? Because the angles around one point are equal to two right angles. So if the line parallel to the side had been drawn up, it would have been clear immediately on seeing it.”2 I will refer to the theorem, exactly as we found it in the mentioned passage, as [1]. Grosso modo, Aristotle’s [1] establishes that if we draw up a specific parallel to a side of one triangle, we can “discover” the following geometrical theorem (therefore, I repeat, understanding τὰ διαγράμματα-a concept used by Aristotle a few lines before: Met. 9, 1051a22-as “geometrical theorems”, not as particular geometrical figures), which is part of the mathematicalscientific universal knowledge: the (size of the) three inside angles of every existent triangle combined are equal to two right angles or 180 degrees (90 degrees from one right angle and 90 degrees from the other).
The geometer Euclid includes a remarkably similar theorem to Aristotle’s [1] in his Elements. The geometrical compilation titled Elements was written around 300 BC (therefore, later than when Aristotle lived) and has had an enormous influence on Western philosophy and science in general; it established an extremely useful basis for the development of geometry. We must clarify that, in fact, Euclid made a compilation of several theorems proposed and established by previous geometers. Nevertheless, not all the theorems that we find in Elements are prior to Euclid; a few of them were indeed created or imagined or discovered by him, for example, the “fifth postulate”.3 It is also the case that most of the theorems in Elements had been available to Aristotle before the existence of such text.
The systematic methodology that Euclid utilized in his approach to geometry was a relative novelty,4 but the problems he studied were not: we are talking about an approach that later would be used by other researchers, that is, the demonstrative method to discover/generate mathematical and/or geometrical knowledge, in which the scientist starts from basic principles and then utilizes them to demonstrate even more complex propositions. This “demonstrative logic” is a mathematical method previously defended (and used) by Aristotle.
Demonstrative logic explains how a hypothesis is proved; it does not explain how it ever occurred to anyone to accept the hypothesis as something to be proved or disproved. […] Demonstrative logic is the subject of Aristotle´s two-volume Analytics, as he said in the first sentence of the first volume, the Prior Analytics […]. He repeatedly referred to geometry for examples. However, shortly after having announced demonstration as his subject, Aristotle turned to deduction, the process of extracting information contained in given premises-regardless of whether those premises are known to be true or even whether they are true. After all, even false propositions imply logical consequences (cf. A 18); we can determine that a premise is false by deducing from it a consequence we already know to be false. A deduction from unknown premises also produces knowledge-of the fact that its conclusion follows logically from (is a consequence of) its premises-not knowledge of the truth of its conclusion (Corcoran, 2009, p. 3).5
Before I continue, I must say that I subscribe to the interpretation that, in Aristotle’s philosophy, mathematicals (mathematical objects or issues) are not platonic ideas or forms; neither are they identical to sensibles.6 Aristotle’s position considers geometers as “separators” of geometrical properties (Mueller, 1970, p. 168). We will see that, in Metaphysics 9, 1051a24-27, this vision of the treatment of geometry is accomplished. For instance, with the instruction of drawing a sole parallel to a triangle’s side, a universal geometrical property of triangles would be actualized or would become evident. Therefore, I agree with Henry Mendell (1984, pp. 359-360) that in the aforementioned passage the philosopher’s objective:
[…] is to show how the constructions made knowledge possible. The ekthesis being complete, all one has to do is see the appropriate relations and recall the right theorems for the proof and the theorem to be evident. He offers two examples [in Met. 1051a21-33] where one does not see the proof until one has all the constructions laid out which are needed for the proof. Any reconstruction of his diagram or proof must take into account the fact that all one is given is the initial figure determined by the statement of the theorem, a triangle or an angle in semicircle. Any construction to be made must be stipulated only then. And then the proof must be evident, given the theorem appealed to.
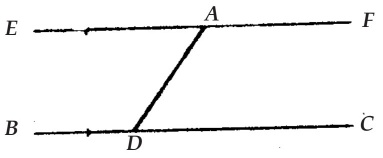
It is known that one of the theorems compiled by Euclid is remarkably similar to theorem [1]; such Euclidian theorem is the Proposition 32 of book I. Henry Mendell (1984) has already concluded that Aristotle’s theorem is neither equivalent nor based on Euclid. Mendell proposed that [1] is based on Pythagoric theorems, a proposition with which I also disagree as I will explain later. Furthermore, in contrast with Mendell and others, I will also advance a concrete pedagogical-philosophical reason for the nonequivalence between theorem [1] and the longer theorem Proposition 32 of book I (or longer versions). This “proposition”, which from now on I will refer to as “P32”, states the following:
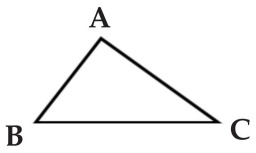
In any triangle, if one of the sides be produced, the exterior angle is equal to the two interior and opposite angles, and the three interior angles of the triangle are equal to two right angles. Let ABC be a triangle, and let one side of it BC be produced to D; I say that the exterior angle ACD is equal to the two interior and opposite angles CAB, ABC, and the three interior angles of the triangle ABC, BCA, CAB are equal to two right angles. For let CE be drawn through the point C parallel to the straight line AB. Then, since AB is parallel to CE, and AC has fallen upon them, the alternate angles BAC, ACE are equal to one another. Again, since AB is parallel to CE, and the straight line BD has fallen upon them, the exterior angle ECD is equal to the interior and opposite angle ABC. But the angle ACE was also proved equal to the angle BAC; therefore the whole angle ACD is equal to the two interior and opposite angles BAC, ABC. Let the angle ACB be added to each; therefore the angles ACD, ACB are equal to the three angles ABC, BCA, CAB. But the angles ACD, ACB are equal to two right angles; therefore the angles ABC, BCA, CAB are also equal to two right angles (Elementa, I.32 [P32]).7
Figure 1, provided below, illustrates Euclid´s proposition.
Comparing the passage of Met. 1051a24-27 with Elements I, P32, one notable difference can be appreciated: in Aristotle´s [1] we do not find the phrase “let one side of it BC be produced to D” (τῶν πλευρῶν προσεκβληθείσης ἡ ἐκτὸς). That is, the principal difference between the two theorems is that P32 proposes the enlargement of one side of a triangle (B´C´, that is, to make the line C´D´), and it is only in a second part of the proposition that Euclid speaks about drawing a parallel. The major similarities between both theorems are as follows:
a). the geometer must draw a single parallel to one side, and
b). it is concluded that there are two rights (ὀρθαὶ) in all triangles (τρίγωνος).8
Conjectures
I now propose some possibilities that could address why Aristotle did not include the phrase “let one side of it BC be produced to D” (included in P32 or other previous equivalent theorems) in his [1]. I provide six conjectures for explaining this problem where the fifth and sixth could be pedagogical-philosophical reasons:
1). “Mistakenly,” Aristotle did not write the “complete” proposition.9
2). Aristotle assumed that all his readers would know the “complete” proposition (i.e., as we find it in Euclid), and he did not deem it necessary to enunciate its other parts.
3). A section of [1] got lost because of the transference of manuscripts or similar reasons.
4). As the Euclidean compilation was written after the publication of Metaphysics, Aristotle did not write the proposition “incompletely” because Euclid was the first to add that, to prove the theorem, side B´C´ needs to be elongated.
5). Aristotle intentionally wrote the “incomplete” proposition for some other, unknown, (philosophical) reasons.
6). Aristotle intentionally “amputated” the theorem to emphasize that with (enough) deduction, it could actualize/unveil geometrical knowledge.
I will now try to argue that conjecture 6 is the strongest of all (but not the only plausible one, as it is compatible with conjectures 4 and 5). I claim that Aristotle could have thought that, by establishing the drawing of a sole parallel (without elongating any side), his readers would see clearly, using deduction-understanding Aristotle’s (evident) deduction as the “final conclusion” derived logically from some premises (Corcoran, 2009, p. 2)-, that the three angles of all triangles are equal to two rights, and would therefore clearly see an instance of universal and scientific knowledge. I consider this method of representing [1] to support the interpretation of Aristotle’s “scientific knowledge” as “knowledge of universals” and “explanatory knowledge”; therefore, it could be seen as supporting Pieter Sjoeder Hasper and Joel Yurdin’s (2014) view of “scientific knowledge” as knowledge that allows one to “fully grasp the real universal, the salient feature which figures in explanations”.
To explain this, two different reconstructions of [1], one by W. D. Ross (1924) and one by Myles F. Burnyeat (1984), will be useful. I analyze both in the subsequent sections. Another expert on geometry, the mathematician Mario Bunge (2001, p. 11), states, regarding [1], that we must give primacy just to the drawing of a parallel of one triangle´s side; however, he does not state why we must give it such primacy. He does not offer a more complete interpretation of [1] either. Additionally, Bunge does not shed light on the problem of the difference between [1] and P32, nor on the topic of discovering mathematical knowledge through deduction in Aristotle.10
Two reconstructions of [1]
W. D. Ross (1924, pp. 269-270) also reconstructs Aristotle´s [1] based on the description of the theorem as it is found in Euclid’s P32 (even though this could make his explanation inadequate chronologically, as the Elements did not exist in Aristotle’s time):
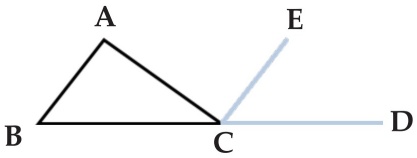
24-26. The proposition is Euc. i. 32. The given figure is [figure 2]
We have only to “divide” (in this case to divide the space surrounding the triangle) in order to see the reason why the interior angles of the triangle must be equal to two right angles. [See figure 3.]
Produce BC to D and draw CE upwards (ἀνῆκτο) parallel to BA. Then the angle CAB=ACE and ABC=ECD (Euc. i. 29). Therefore BCA+CAB+ABC=BCA+ACE+ECD, which=BCA+ACD [;] which= two right angles (i. 13). Therefore the interior angles of the triangle=two right angles. Of the two supplementary lines which had to be drawn, Aristotle mentions only CE. In Euclid this theorem is the second part of a proposition of which the first part is that “in every triangle, if one of the sides be produced, the external angle is equal to the two interior and opposite angles”, so that CD is supposed to be already drawn; and Aristotle probably knew the proposition in its Euclidean form.
Ross affirms that [1] is equal or corresponds to P32 although, in Met. 1051a24-27, it is not stated that two lines must be drawn to show that “the angles around one point are equal to two right angles”. Ross does not offer enough evidence to support that [1] is a shorter version of P32-his text lacks this important specific sentence. At most, Ross could conjecture that P32 was based on [1] (and not vice versa) and that Euclid “completed” the idea (this can be said in favor of my 4th conjecture); however, Ross´s figure 2 does not correspond to [1] as it has two extra lines drawn beside the triangle, while Aristotle only alludes to drawing up one line.
Euclid used previous texts from other geometers and mathematicians to write his Elements and it is very plausible that he used Metaphysics (and/or Prior Analytics) as a source for his Elements (Barnes, 1981, p. 18).11 However, Euclid could also have used pre-Aristotelian works in his own work: “[…] Euclid’s Elements and, in particular, Euclid’s geometry were merely instances of the application of a previously thought out/ discovered/known method, and, thus, […] the axiomatico-deductive [sic] method existed prior to the axiomatico-deductive formulation of geometry” (Agashe, 1989, p. 109). Such pre-Aristotelian works would have been the basis for Aristotle to write his geometrical examples in Prior Analytics (Mueller, 1974, p. 35) and Metaphysics. In any case, it is also probable that Euclid added another phrase to Aristotle´s [1] in order to elaborate P32.
It is not possible to confirm that Aristotle knew [1] in the form we see it in P32; ergo, we cannot confirm, without doubt, that Aristotle mistakenly wrote it down “incompletely” or that some parts of the proposition were lost. Therefore, it is not possible to confirm nor deny, for now, conjectures 1 and 3. Ross also affirms that figure 3 is the illustration of [1]; however, this is incorrect if we take [1] literally, as [1] does not include the Euclidean instruction to elongate BC. In 1051a, the line CD is neither supposed, nor stated, nor suggested “to be already drawn”, as Ross suggests.
I insist: it is very plausible that Aristotle influenced Euclid, while the inverse influence is not possible. Such Aristotelian influence is notable in the expository and/or methodological similarities between theorems [1] and P32, among other similarities. Aristotle´s axiomatic method or demonstrative logic, in which the “scientist” starts with definitions of his fundamental terms and then such definitions are used as premises to aid in the deduction or actualization of other parts of the definition (Sorabji, 2003, p. 269), was thoroughly adopted by Euclid. It is very plausible that Aristotle also influenced Euclid in the way he conceived the architecture and development of his entire book Elements. The philosopher influenced the geometer in the general epistemological development of his scientific thought.
There is also the possibility that Aristotle could have known [1] in the way we see it in P32 and decided to write it in a shorter form that is also shorter than the versions we find in other sections of the Aristotelian corpus, such as in Posterior Analytics, as Aristotle may have conceived it to be sufficient simply to draw a parallel to corroborate that all angles inside one triangle are equal to two right angles; this speaks in favor of conjecture 6, as the deduction would provide the remaining scientific-universal knowledge without the need to draw any additional lines. Additional possibilities include that Aristotle may have known [1] as it is provided in Metaphysics, or that Aristotle understood the theory in yet another presentation, and yet, however, decided to write it in the way it is shown in the manuscripts in order to emphasize the power of the deduction in demonstrative logic.
“Logic was […] seen [in ancient times] as an engine to derive new, ‘less obvious’ truths from old, ‘more obvious’, ‘self-evident’ truths” (Agashe, 1989, p. 113)-this is the power of deductive logic that Aristotle wants to emphasize with his description of [1]. In other terms, if [1] was modified from a version prior but identical to the version in P32 (which could have been copied identically by Euclid in his Elements), or if it was modified from yet another version, the philosopher may have considered that drawing a sole parallel was enough to prove the theorem and to prove the force of the deduction in order to unveil mathematical knowledge, i.e., there was no need to draw any additional line or specify any other instructions.
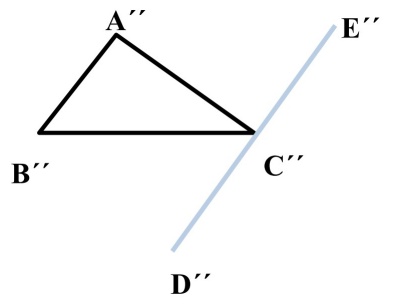
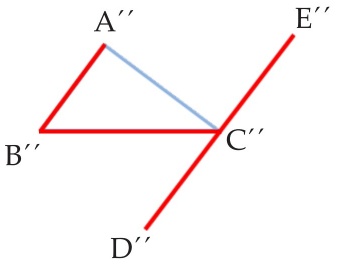
I will now present Burnyeat’s reconstruction of [1] and then explain how this reconstruction supports conjecture 6. Burnyeat’s illustration of Met. 1051a24-27 is as follows:
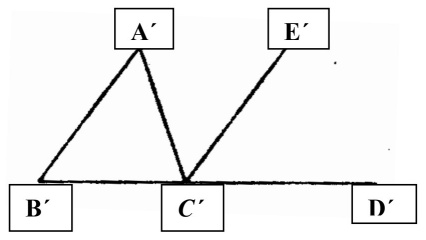
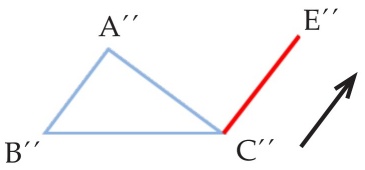
I suggest two clear reasons to consider Burnyeat´s reconstruction is correct: i) the Aristotelian text instructs to draw only one line up, specifically “the line parallel to the side”, and in Burnyeat´s figure 4 there is a single line drawn additionally to the triangle; ii) Burnyeat’s figure 4 does not contradict the idea presented in Metaphysics, as Aristotle never states where the parallel is to start, and only affirms that the parallel should be “drawn up” (ἀνῆκτο). I must pinpoint, incidentally, that i) also contradicts Henry Mendell’s interpretation and reconstruction of, [1] as the parallel proposed by Mendell (1984, p. 361) is not drawn up but horizontally.
In Burnyeat’s illustration (figure 4), we see that the straight line D´´E´´ is parallel to the side B´´A´´ and that the angle C´´A´´B´´ is equal to the angle A´´C´´E´´, i.e., C´´A´´B´´ = A´´C´´E´´. Then, A´´B´´C´´ = B´´C´´D´´, and we conclude that B´´C´´A´´ + C´´A´´B´´ + A´´B´´C´´ = B´´C´´A´´ + A´´C´´E´´ + B´´C´´D´´ = two right angles. Thus, the internal angles of the triangle are equal to two right angles.
Therefore, also in geometrical terms, it is not necessary for Aristotle to enunciate the theorem as it is found in P32, that is, in a longer form than [1]: it could be evident, drawing only one line, that all internal angles of any triangle are equal to two right angles. Aristotle claims that [1] is “immediately” understood. However, I propose that such an understanding is only immediate as long as we know a prior premise; specifically, we must have the knowledge of another theorem, one equivalent to Euclid’s Proposition 31.
A premise for [1]
I now intend to explain how the knowledge of a premise equivalent to Euclid’s Proposition 31 must lead to a “more immediate” understanding of the actualization of mathematical knowledge in theorem [1].12
As I have said, Aristotle took theorem [1] from pre-Euclidean works. More precisely, such works could have also been the raw material for Euclid’s Elements. Therefore, the Euclidean Proposition 31 of book I could have been based in other, already existent theorem(s) such that could be used by Aristotle. Proposition 31 of the Elements states the following:
Through a given point to draw a straight line parallel to a given straight line. Let A be the given point, and BC the given straight line; thus it is required to draw through the point A a straight line parallel to the straight line BC. Let a point D be taken at random on BC, and let AD be joined; on the straight line DA, and at the point A on it, let the angle DAE be constructed equal to the angle ADC; and let the straight line AF be produced in a straight line with EA. Then, since the straight line AD falling on the two straight lines BC, EF has made the alternate angles EAD, ADC equal to one another, therefore EAF is parallel to BC (Elementa, I. 31).13
The knowledge provided by a theorem equivalent to Euclid’s Proposition 31, which I will name A1, is sufficient for the deductive process of [1] where, after drawing up a single line, we will “immediately” see the conclusion of [1], i.e., we will understand the new knowledge provided by this theorem. Of course, to understand A1, we must also have basic knowledge of geometry; however, my point is that, in understanding a proposition/premise identical to Euclid’s 31, given A1, it is necessary to make a geometrical deduction and immediately see that the triangle´s internal angles are equal to two right angles, even though it is not always necessary, in deductive logic, to know a premise to produce new knowledge regarding the conclusion of a given deduction. “A deduction from unknown premises also produces knowledge of the fact that its conclusion follows logically from (is a consequence of) its [unknown] premises” (Corcoran, 2009, p. 3).
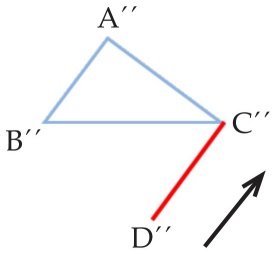
Knowing A1 is necessary to emphasize that the line B´´C´´ of Burnyeat’s reconstruction (figure 4) incises two straight lines simultaneously: B´´A´´ and D´´E´´. For pedagogical reasons I’ll allow myself to make a change, though I am conscious that the position of a geometrical figure does not affect the verification of a (Euclidean) geometrical theorem, I suggest rotating the lines B´´C´´, B´´A´´ and D´´E´´ of figure 4 by 90 degrees in order to appreciate that they will form a similar figure to figure 5. Observe the red lines in figure 6 and compare them with figure 5 by rotating them 90 degrees.
If we apply theorem A1 to figure 6, we will see that the interior angle A´´B´´C´´ is equal to exterior angle B´´C´´D´´. A1 would tell us: make an angle incising the point (vertex) C´´ of a triangle´s side (B´´C´´) with the same measurements of the triangle´s interior angle A´´B´´C´´; then, draw up D´´C´´. Proceeding this way, we will automatically know that D´´C´´ is parallel to B´´A´´.
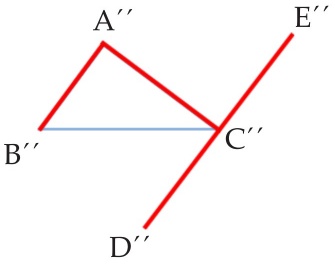
Furthermore, A1 also shows that line A´´C´´ of Burnyeat’s figure 4 simultaneously incises two straight lines, B´´A´´ and D´´E´´, in a similar way to how, in figure 5, the line AD incises two lines: BC and EF. Ergo, the straight line A´´C´´ also makes a similar figure to that of line AD, which can be appreciated by rotating the following figure 8 by 90 degrees (observe the red lines):
Following A1, we will have that the interior angle B´´A´´C´´ is equal to exterior angle A´´C´´E´´ (as in figure 5, the angle EAD is equal to the angle ADC, and angle FAD is equal to ADB). A1 would tell us: make an angle incising the point C´´ of a triangle´s side (A´´C´´) with the same measurements of the triangle´s interior angle B´´A´´C´´; then, draw up C´´E´´. In doing this, we will automatically know that C´´E´´ is parallel to B´´A´´.
A consequence of A1’s way of constructing one sole parallel (D´´E´´), as seen in figures 7 and 9, is that A´´B´´C´´ = B´´C´´D´´ and C´´A´´B´´ = A´´C´´E´´. Then, we also immediately have that: A´´B´´C´´ + C´´A´´B´´+ B´´C´´A´´ = B´´C´´D´´ + A´´C´´E´´ + B´´C´´A´´ (as shown in Burnyeat´s figure 4). With the drawing of this sole parallel of a triangle’s side, D´´E´´, it is evident that the angles of the same triangle (or of any triangle) are equal to two right angles, as the angles surrounding a point (in this case C´´) are always equal to two right angles (this last claim is found literally in Aristotle´s geometrical theorem [1]) because: If B´´C´´D´´ + A´´C´´E´´ + B´´C´´A´´ (the surrounding angles of C´´) = two right angles, and if A´´B´´C´´ + C´´A´´B´´ + B´´C´´A´´ = B´´C´´D´´ + A´´C´´E´´ + B´´C´´A´´, ergo, A´´B´´C´´ + C´´A´´B´´ + B´´C´´A´´ = two right angles. This is how we prove that, by drawing only one parallel of one triangle´s side, we clearly and evidently see that the addition of the three angles of all real triangles in the universe is equal to 180 degrees.
Actuality and potentiality
Aristotle considers that universal knowledge can be formulated syllogistically14 and that [1] can be presented as a syllogism; hence, we have the following deduction: when a triangle with points (vertices) named A´´, B´´, and C´´ undergoes the drawing of a parallel (D´´E´´) for a side B´´A´´, where C´´ is the point of intersection between the parallel and the triangle, we have the following consequences:
a). the three formed angles surrounding point C´´ (one of them already existent: angle BCA of the triangle, and two newly formed angles) are always equal to two rights,
b). the three angles inside the triangle (ABC, BCA, and CAB) have the same measurement of the angles surrounding point C´´,
c). therefore, we arrive at the “universal knowledge” that the three angles of all triangles are equal to two rights.
I agree with Ian Mueller’s description of Aristotelian “universal knowledge” (but I disagree with his position that in Aristotle’s philosophy of mathematics, geometrical figures per se could be actualized,15 as I will insist in the following paragraphs. What is actualized is the geometrical theorem-διάγραμμα). It is more probable that Aristotle planned to place more emphasis on the idea of knowledge’s potentiality when he wrote about geometrical exemplifications/theorems. If this is true, the Greek philosopher established that drawing a single line is sufficient to actualize universal knowledge.
[…] out of ordinary geometric reasoning arises a universal knowledge, e. g., the knowledge that any triangle has interior angles equal to two rights. Universal knowledge is conceptual and can be formulated syllogistically. However, it has no object over and above the objects of ordinary geometric reasoning, and in fact conceptual syllogistic reasoning is only a reformulation of ordinary reasoning (Mueller, 1970, p. 171).
I propose that the objective of enunciating [1] the way we find it in Metaphysics is to emphasize that universal scientific knowledge is in potentiality and can be immediately transformed into actuality by just drawing a straight line and with the power of deduction (therefore, the exact form in which [1] is asserted, a shorter “variant” of P32-and a more mature Aristotelian variant (Mendell, 1984, p. 359)-is also an excellent way to cause more impact on the readers). I want to suggest that Aristotle’s purpose in writing [1] as he did it is to demonstrate that the knowledge of more and more complex premises is in potentiality inside geometrical figures and/or mathematical propositions. But with this, we must not understand that some lines (parts of a geometrical figure) are in potentiality in some geometrical figures, much less that specifically line C´´D´´ in Ross’s figure 3 is in potentiality in [1], only that a new scientific knowledge (a new theorem) is in potentiality.
Regarding the actualization of knowledge, Aristotle establishes that “[a]t all events it is clear that it is prior in account (for it is because it can be active that what is capable primarily is capable […]; and there is the same account also in the other cases, so that it is necessary for the account and the knowledge of the one to precede the knowledge of the other)” (Met. 9, 1050a13-18). This could mean that, in order to actualize geometrical theorems/knowledge, we use geometrical figures and, in using them, we must have prior geometrical knowledge (as for understanding [1] we must know a theorem equal to Proposition 31 of book I, as well as other theorems, such as Euclid’s proposition 27 of book I).
Aristotle clearly confirms that the “objects of mathematics” are not corporeal objects:
Thus we have sufficiently shown (a) that the objects of mathematics are not more substantial than corporeal objects; (b) that they are not prior in point of existence to sensible things, but only in formula; and (c) that they cannot in any way exist in separation. And since we have seen that they cannot exist in sensible things, it is clear that either they do not exist at all, or they exist only in a certain way, and therefore not absolutely; for “exist” has several senses […]. [S]o this is also true of geometry. If the things of which it treats are accidentally sensible although it does not treat of them qua sensible, it does not follow that the mathematical sciences treat of sensible things-nor, on the other hand, that they treat of other things which exist independently apart from these (Met. 13, 1077b13-17 and 1078a2-5).16
Based on Bechler’s (1995) reflections, before proceeding to some conclusions, I must highlight some important points for understanding why Aristotelian mathematical and geometrical “entities” lack the potentiality and actuality of sensibles (bodies):
[…] mathematical entities cannot be interpreted as standard attributes (i.e., such as “red”, and “heavy”) of sensible things, for there is the irreconcilable difference that not only are they not sensible attributes, but rather they qualify sensible things qua what they actually are not. […] [M]athematical predicates are possessed by the sensible thing not only qua something it is not ever actually, but, also qua something it cannot possibly be, that is, irrespective of its consistency-potentiality. […] [M]athematics is not “a science of sensibles” (1078a2), for it deals neither with actual nor with standardly potential (i.e., such that can actualize) properties of sensibles (1995, pp. 161-162).
“Since mathematical entities are not substances, they cannot be material. The triangle which geometry studies is not a wooden thing, nor any other material thing” (1995, p. 177). With this in mind, we can definitively cast away the “intuition” that the line C´´D´´ of Ross’s figure 3, or the elongation of B´´C´´, is potential.
Ergo, I interpret as inaccurate the argument that says that Aristotle did not have to establish that elongating BC is necessary to actualize the knowledge that all triangles have two rights inside because he thought all triangles’ sides are elongated potentially: mathematical lines or figures could not be in potency (only mathematical knowledge). For Aristotle, neither a parallel of B´´A´´ nor the other side of the triangle (nor any part of any geometrical figure) is in potency.
Conclusion
In this article, I argue that the stronger conjecture to explain why [1] looks as it does is a pedagogical-philosophical one, conjecture 6 (leaving aside the possibility that conjectures 4 and 5 could also be correct). For Aristotle, drawing up a parallel of one triangle’s side is enough to actualize the “all triangles have two right angles” theorem, and for him, such theorem is understandable and evident for any reader (with some specific knowledge about geometry). Therefore, my somehow heterodox posture about [1] is that this theorem needs no emendation and/or elongation. I claim that [1] has a condensed form compared to other versions because Aristotle needed that condensation to emphasize the “power” of deduction.17 I have simultaneously given strong reasons for supporting the correctness of Burnyeat’s explanation of [1] and for rejecting Ross’s reconstruction.
Lastly, I want to summarize some of the arguments that I consider essential to make the interpretation of Burnyeat the most acceptable: (I) Metaphysics 9, 1051a24-27 states that we have to draw a sole parallel and not any other line, and the illustration of Burnyeat (figure 4) draws just a parallel; (II) the parallel suggested by Burnyeat does not contradict what is said in 9, 1051a24-27, because this passage does not tell us where to start the parallel or how long it should be, which gives us the liberty to draw it as we want except for one thing: the line should be drawn up (ἀνῆκτο); (III) it is necessary to know a theorem equivalent to the one we find in Euclid’s Proposition 31 (A1) for the readers to see “immediately,” after we draw up a parallel of one side of a triangle, that the angles of such triangle are equivalent to two rights.
Aristotle wanted to emphasize the potentiality of (mathematical) knowledge, and he achieved such emphasis by demonstrating that knowledge is in potency in a geometric figure, a triangle, and it will turn into actuality by drawing just one straight line, in this case a parallel. To accomplish this emphasis, we should not draw any other line around the triangle. Contrary to Ross’s proposal (figure 3) or P32 (figure 1), and to Mendell’s conjecture that [1] is based on Pythagoras’s theorem (i.317- 321), the latter could also be dismissed as equivalent to [1] because it does not fulfill the instructions set by the term ἀνῆκτο. In his theorem, Pythagoras talks about drawing a horizontal parallel on top of the triangle.
Aristotle’s philosophy of mathematics does not intend to demonstrate through geometry that the sensitive/corporeal points, lines, or figures are per se in potency, but rather that knowledge/theorems is the one “thing” that is. The parallel D´´E´´, as proposed by Burnyeat, actualizes immediately and clearly, with some previous notions of geometry and other premises (mainly the theorem equal or similar to Proposition 31), a universal and scientific knowledge-which therefore partly leads to the acceptance of the perspective of “particularist” interpreters of Aristotle, who “emphasize Aristotle’s claims that scientific knowledge is of universals while experience is of particulars, and that scientific knowledge alone involves universal single judgements” (Hasper & Yurdin, 2014, pp. 128-129).











 nova página do texto(beta)
nova página do texto(beta)