Servicios Personalizados
Revista
Articulo
Indicadores
-
 Citado por SciELO
Citado por SciELO -
 Accesos
Accesos
Links relacionados
-
 Similares en
SciELO
Similares en
SciELO
Compartir
Investigación económica
versión impresa ISSN 0185-1667
Inv. Econ vol.67 no.264 Ciudad de México abr./jun. 2008
The Political Economy of Policy Reform: The Case of the Dominican Republic
Economía política de las reformas: el caso dominicano
Julio G. Andújar-Scheker*
* Universidad Católica Santo Domingo (UCSD), <julio.andujar@empirica.com.do>.
Received January 2007
Accepted January 2008.
Resumen
El siguiente artículo presenta una visión teórica de la economía política de las reformas en países en desarrollo con regímenes presidenciales. Considerando la experiencia de la República Dominicana durante los noventa, se desarrolla un modelo que intenta replicar las interacciones entre agentes políticos durante un proceso de discusión de reforma en el Congreso de un país. El modelo tiene como punto de partida la decisión discreta de un Presidente entre liberalizar o mantener un status quo proteccionista. Si opta por liberalizar, el Presidente somete a un Congreso antireforma su propuesta. La decisión del Congreso de aprobar o rechazar la propuesta depende de las contribuciones de dos lobbies rivales que se enfrentan en un juego no-cooperativo antes de la decisión del Presidente. El modelo muestra como el comportamiento de los lobbies depende del comportamiento de su rival y como sus decisiones afectan la elección presidencial. Explica además, por qué en países en desarrollo con larga tradición autoritaria las reformas son pospuestas indefinidamente.
Palabras clave: economía política; reformas estructurales; política endógena; República Dominicana.
Clasificación JEL: C7, D7, O5
Abstract
This paper provides a theoretical framework for the analysis of the political economy of policy reform in developing countries with presidential regimes. Drawing from the Dominican Republic's reform experience in the mid-nineties, I build a model aimed to replicate interactions between political agents throughout the discussion process of a policy reform in the Congress of a developing country. A reform-committed president is the agenda-setter and decides between total liberalization and a protectionist status quo. If the president decides to liberalize, he submits to an opposition-led Congress a tariff-reform. Congress' decision on approving or rejecting the proposal depends on the contribution of rival lobbies, which plays a non-cooperative game within the overall game. The model shows how lobby's behavior depends on the decision made by its rival and how the equilibrium of the lobbying game ultimately determines the president's choice. In addition, it explains why in developing countries with a large authoritarian history, reforms are constantly delayed.
Key words: political economy, structural reforms; contest, endogenous policy; Dominican Republic.
Introduction
Economic reforms redefined deeply rooted political relations in Latin America and the Caribbean in the eighties and nineties. Trade liberalization, along with market-oriented reforms, set forth the conditions to end the inward-looking development strategy applied in the region with the support of import-substituting industrials, urban workers and populist governments. In most countries, reforms needed Congress approval. This led to real political battles in a region learning the pros and cons of the democratic ruling. One of those battles took place in a small Caribbean island, the Dominican Republic (DR).
In 1990, the dr launched a reform program based on key policies recommended by the Washington Consensus.1 The platform included short-term policies oriented to restore and maintain macroeconomic stability and long-term or structural adjustments aimed to enhance market competition. While the government brought short-term policies to a close, it hardly finished structural changes to avoid political confrontation. Weak institutions and the existence of an inadequate setting for political decision-making allowed the postponement of long-term reforms.
Major changes to the institutional framework and hence, the possibility of relaunching long-term reforms, came only after an electoral crisis triggered political disruption in 1994. As a result of the political unrest the leadership of the country negotiated a multiparty agreement, Pacto por la Democracia, which set up new electoral rules, including a second ballot of voting and the prohibition of presidential reelection. Under these rules a minority party reached power in 1996, an unprecedented event for this young democracy.2 Once in office, the new incumbent submitted to an opposition-led Congress a comprehensive reform of the tariff and tax systems, thus reviving the interrupted first wave of structural adjustments.
Since for the most part Congressmen are self-driven politicians, ex ante, Congress has an incentive to reject the president's proposition. Its final decision, however, depends on the contributions of interest groups. While possible political interactions in such setting are well documented for truly democratic nations, this bargaining process constitutes a new experience for a traditionally authoritarian country with weak institutions like the DR.3 The aim of this paper is precisely to model political interactions within a framework that resembles the institutions of developing nations characterized by a history of authoritarianism and presidential regimes.
The rest of the paper is set out as follows. Section II places the model in the context of the new political economy literature. Section III presents a brief discussion on lobby formation and on the political economy of policy reform in the DR. Section IV turns to the model describing the overall political game, where an opposition-led Congress decides on a reform bill sent by the president. Section V focuses on a reduced-form game played by opposing lobbies within the overall game. Section VI shows the results of the overall game, meaning it discusses the president's decision and the final outcome of the political game. Finally, Section VII summarizes the findings of the paper and its relevance regarding political economy issues in reforming the economy of developing countries.
The political economy of reforms: a brief survey
Tommasi and Sturzenegger (1998) argue that three ingredients mold the political economy of a stylized country: 1) there are powerful pressure groups; 2) these groups influence public policies; 3) pressure groups induce income redistribution toward their constituents. While the study of the reform experience in the Dominican Republic helps to identify these powerful pressure groups and the mechanisms they use to rework public policy in their favor, the political economy modeling leads to establish potential behavior in different scenarios. Hence, it improves the chances of approval through minimizing the source of conflicts.
Overall, the model consists of a political game where a reform-committed president, decides between protectionism and trade liberalization. Ex ante the president favors trade liberalization, which for a small open economy is welfare maximizing. However, lobbies' contribution to Congress, as well as a fixed cost on reforming, could lead the president, ex-post, to keep the protectionist status quo. The contributions of lobbies arose from a reduced-form game played within the general framework.
A few branches of the economic literature provide important insights for modeling within this context. The obvious starting point is the Public Choice approach. Public Choice (Mueller, 1993) is the economic study of nonmarket decision-making or simply the application of economics to political science. The turning point of this literature is Arrow (1951), later enriched by Downs (1957), Buchanan and Tullock (1962) and Olson (1965). As the application of economics to political behavior, Public Choice brought in concEPTs as rational choice and equilibrium analysis to politics.
Public Choice or the economics of politics expanded during the seventies and eighties under the label of New Political Economy (NPE). NPE based its analysis on the study of political interactions that resembles advanced industrial democracies. Finlay (1991) asserts that the institutional framework covered in NPE research corresponds more specifically, to that ruling in contemporary United States. The first NPE models, built to study political behavior in developing countries, appeared in the late eighties and early nineties with the so-called New Political Economy of Policy Reform (NPEPR).4 Helpman and Persson (2001) identify three groups of models within the NPEPR literature: electoral, lobbying and legislative models. A great deal of the modeling in this paper relates to the second group.
In addition to the Public Choice and NPE approaches, modeling in this context draw from Endogenous Policy Theory (EPT). Brock, Magee and Young (1989) define EPT as a theory that determines a policy through the use of rational maximizing behavior by participants in the political process.5
Pant (1997) classifies endogenous policy models into three groups: models of public interest, models of self-interest and political market models. Within the third group modeling can be demand-determined, supply determined or full market approach. Following this classification, the model developed in this paper is a political market model of the demand-determined type.
For the reduced-form game I also draw from the Contest Literature, as the lobbying game is a social interaction where two players (opposing lobbies) exert efforts (contributions) in hopes of winning a prize (the policy).6 Economists use Contest Theory to model other economic and social interactions. While Hirschleifer (1989) and Skaperdas (1992) relied on contest theory to solve economic conflicts, Loury (1979), Stiglitz and Dasgupta (1980) and Dinopoulos and Syropoulos (1998) used it to model R&D rivalry for a profitable innovation. Rosen (1986) applied contest theory to study employment tournaments. Dixit (1987) and Nitzan (1994) analyzed contests on public goods.
A key feature of contest modeling is the choice of a contest success function (CSF), which provides each player's probability of winning a prize as a function of all players' efforts.7 CSF's choice is frequently described by the logit function, which defines the probability of winning a prize as the ratio between one player's efforts over the total pool of efforts in the contest. In our setting, the probability of winning the lobbying contest is given by the probability of Congress approving reforms. I use a logit function in the model to define this probability.
Summarizing, the model set out in this paper uses the Public Choice Approach to mimic political interactions over a policy reform between a reformer president and an opposition-led Congress. It builds within this framework a reduced-form lobbying game, that classifies as a lobbying model under the NPEPR classification, as a demand-driven political market model under EPT's categorization and as a lobbying contest in the domain of Contest Theory. The key features of both, the general framework and the reduced-form lobbying model, are described in section 4. Meanwhile, I turn to lobby formation and issues on the political economy of policy reform in the DR.
Reforming the economy in the Dominican Republic
Reform experience in the DR drives modeling in this paper. This is consistent with Williamson (1994), which states that for political economy issues on policy reform, case studies that consist in a careful examination of the specific reform processes in individual countries represent the only possible practical methodology. In this section, I draw relevant information from a country study presented in Andujar-Scheker (2005) to explore lobby formation and political economy issues on the DR reform process.
Under the intellectual leadership of the Economic Commission for Latin America and the Caribbean, most countries in Latin America (LA) adopted a development strategy based on import substitution.8 The import-substitution strategy (ISI) proved to be successful until the mid-sixties when it began to falter as an efficient economic setup. Despite its problems, ISI remained in place in many countries until economic growth hindered during the 1980s' debt crisis.9 With international credit cut-off and existing policies causing severe inflation, a new wave of reforms expanded throughout the region. Hence, the story of la's reforms is tied to ISI policies and so it is the political economy of it.
ISI adoption in the DR coincides with that of la. For historical reasons, however, the means to promote it were different. While most countries in the region used tariff and quotas to protect their economies, a United States (US) military intervention forbade the use of such policies in the DR. Instead, governments relied on contracts, containing special concessions, to provide protection to industrial firms. Direct contracts accelerated the formation of industrial lobbies. As industrial lobbies played a key role in the process I intend to model, understanding how contracts led to the formation of these interest groups is of the essence to comprehend the political interactions considered in this paper.
Contracts and anti-reform groups
A series of events linked to DR's external debt policy, led to the takeover of the Dominican Customhouse by US Authorities, in 1907.10 As customhouses remained in the hands of the US Government until 1947, tariff policy became a prerogative of us Authorities for almost half a century, forcing governments in the DR to find new ways of promoting industrialization. In a move to please a request from Dictator Rafael L. Trujillo, Congress passed a constitution bill that gave the government the right to assign special concessions through contracts to investors in the industrial sector.11
While contracts led to the emergence of an industrial sector, they did so in a pervasive way. Trujillo or prominent members of his family owned either totally or partially most of the new industries created under the contract regime. When the dictatorship crumbled down in 1961, people cried out for the confiscation of Trujillo's properties, including those held in partnership with the big industrialists. Aware that this might end in the nationalization of their property, industrialists grasped the need to organize lobbying groups to transform into law, privileges granted through contracts.
Efforts toward the formation of anti-reform lobbies led to the creation of Asociación Industrial de la República Dominicana (AIRD) and Consejo Nacional de Hombres de Empresa (CNHE) in 1962. The actions of these industrial lobbies resulted in the approval of Law 299 of Industrial Protection in 1968. This piece of legislation along with a tariff bill passed in Congress in 1970 constituted the core of the legal framework for protectionism in the DR during the next twenty years. Hence, reforming the economy entailed the removal of such framework and a frontal battle against powerful industrial groups.
Despite important changes in the structure of the economy in the seventies and eighties, the first attempt to do away with protectionism came in 1990 in the midst of an economic and political crisis. A set of first generation reforms were easily passed in a political setting where a single party dominated both, Congress and the presidency. While passing reform was easy from a political standpoint, the content of it exacerbated differences between old industrialists and businessmen linked to the commercial sector. Contrary to the stance of the former, the latter supported the reforms. Eventually, these groups went separate ways and reform supporters created Unión Nacional de Empresarios (UNE), the first organized pro-reform group in the DR.
In 1996, a new government proposed a second generation of reforms. This time, however, the political setting for reform approval was far more complicated. Different parties ruled Congress and the presidency. At the same time, two opposing lobbies, an anti-reform interest group led by AIRD and CNHE and a pro-reform lobby headed by UNE, represented business interests. While common in advance democracies, the new scenario was hardly known in the DR, a nation used to pass reforms in either dictatorial governments or democratic regimes with a single party controlling both, Congress and the presidency. Modeling in this paper is based on the interactions that arose at Congress from a tariff-reduction proposal the president made in 1996, in a political context like the one described above. The schematic representation of the game follows.
Schematic representation of the game
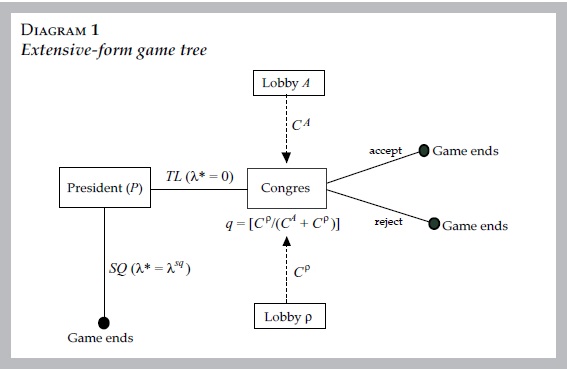
The extensive-form game tree in diagram 1 shows, in solid lines, the core of the model. The president of a small open economy, P, faces a discrete choice between total liberalization (TL) and status quo (SQ). To achieve TL, P has to set A —the share of tariff revenue over total government revenue— equal to zero. Theoretically, any reduction in A for a small open economy is welfare improving. While in this context a reduction in X is welfare-enhancing, the model should work as well in cases when the policy parameter is assumed to be welfare-reducing. To attain SQ, P must keep the actual state of the world represented by λ* = λsq, a share of tariff revenue high enough to protect local industries.
P is the agenda-setter and moves first in the overall sequential game. An opposition-led Congress moves second, meaning it either accepts or rejects P's proposal with some probability q. If P chooses SQ the game ends automatically and the actual state of the world remains in place. If P chooses to liberalize, the game ends following Congress' decision on the proposal. While P's decision is endogenized in the model, Congress's behavior is determined exogenously. Congress acts like a black-box where the final policy decision is taken. Asymmetry between the conduct of P and Congress requires further explanation.
A long history of authoritarianism in Latin American has led, in some cases, to Presidentialism, a phenomenon that concentrates great power in the hands of a democratically-elected president [Inter-American Development Bank (AIDB), 2006]. In an effort to fit this feature into the model, I grant strong leadership to P in the political game through two key assumptions. One of them is the extreme asymmetry just described. The other, which I tackle later, considers P a Stackelberg leader vis-à-vis the rival lobbies of the reduced-form game.
The feasibility of the overall game calls for some extra assumptions. Since the worst payoff the president could get by pursuing TL is exactly equal to the best payoff he would achieved with the alternative strategy, P sees SQ as a weakly dominated strategy. Thus, P will propose unless a cost on proposing is imposed. I assume there is a fixed cost, F, on proposing, which could be read as the cost of putting together a proposal or alternatively, as the opportunity cost the president bear when distracted from other activities. By the same token, an opposition-led Congress has an incentive to reject the policy unless it is motivated otherwise. I assume Congressmen are self-interested politicians, whose decision depends on lobbies' funding. As a result, a reduced-form lobbying contest, preceding the overall game, determines Congress' choice.
Dashed lines in the schematic representation of the game represent the reduced-form lobbying game. In the lobbying contest, two opposing lobbies exert efforts (contributions) to win a prize (the policy). Lobbies move simultaneously, choosing optimal levels of contributions, provided rival's choice. An anti-reform lobby, A, contributes to decrease q or the probability of policy approval while a pro-reform lobby, ρ, contributes to increase it. In such setting, a common point where every lobby maximizes its own expected net benefit given the other lobby's choice represents a Nash equilibrium.
Once the lobbying game is finished, P incorporates the equilibrium outcome to his expected net benefit function on proposing. Recall P acts as a Stackelberg leader vis-à-vis the lobbies in the model. This assumption accounts for the existence of presidentialism in developing countries with a long authoritarian history.12 In the next section, I turn to the lobbying game estimating each lobby's reaction function and the equilibrium of the reduced-form contest.
The lobbying game: estimating the reaction process
In the lobbying contest opposing special-interest groups expend efforts (contributions) to win a prize (a policy). Let E(BLi) and Ci where i = {A, ρ}, be the expected payoff of the policy and the total contributions of the lobbies, respectively. Thus, each lobby's problem consists in choosing a level of C that maximizes E(BLi). Dixit (1987) defines any bribery to receive a contract from a government as a contest and uses two types of functions to define the probability of winning such prize: the probit and logit functions. I use the latter to define q or the probability of Congress approval. With this in mind, let's turn to the pro-reform lobby maximization problem.
The pro-reform lobby (ρ) supports TL, so it tries to influence q in such a way that a new level of λ equal to zero is approved. Let q or the probability of Congress approval be equal to {Cρ/ (CA + Cρ)} and 1-q or the probability of Congress rejection be equal to {CA/ (CA + Cρ)}.13 The pro-reform lobby maximization problem boils down to:
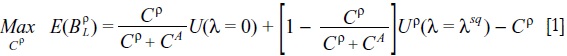
where:
λ: Proportion of total tax revenue collected through tariffs.
Ci:Lobby contributions to Congress and i = {ρ, A}.
q: Probability of Congress approval.
Uρ(λ = 0): Expected utility received by ρ if the policy is approved.
Uρ(λ = λsq): Expected utility received by ρ if the policy is rejected.
Uρλ < 0, Uρλλ < 0
The first order conditions of [1] show how the pro-reform lobby decides the total amount of contribution it will provide to Congress.
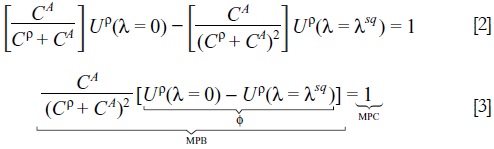
where: Φ > 0, Φ' < 0, <Φ" < 0 and CA ≥ 0.
Lobby ρ optimally chooses the level of contributions that equates Marginal Political Benefits (MPB) to Marginal Political Costs (MPC). MPC is constant and equal to one given our assumption of zero lobby organizational cost.14 MPB, on the other hand, depends on two elements: lobbies' contributions (Ci) and the pro-reform lobby expected utility differential (Φ) from alternative policies. I relate this utility differential to the size of the lobby and use the alternative interpretation indistinctly. The larger the lobby, the more it has to gain from pursuing its favored policy. Total liberalization increases ρ's welfare through the expected utility differential, so as long as MPB exceeds 1 the pro-reform lobby would be willing to increase contributions.
To find the reaction function of ρ I solve for Cρ as an explicit function of CA. The outcome shows the best response of the pro-reform lobby to contributions of the anti-reform lobby.

Notice that if A decides not to contribute, ρ's best response will also be not to contribute. Hence the origin is a point on the reaction curve. To figure out the complete locus of points of p's reaction curve, I totally differentiate [4] to obtain the slope of the reaction function:
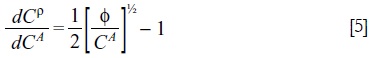
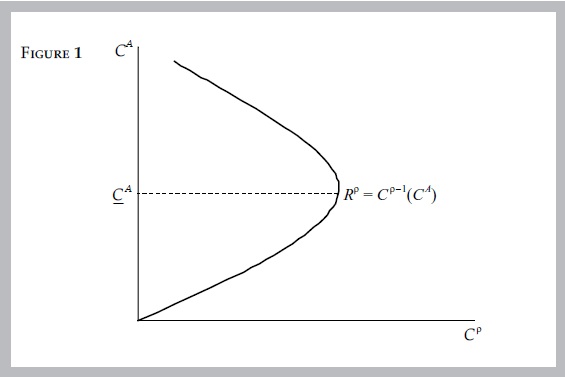
The shape of lobby ρ's reaction curve, Rρ, depends on anti-reform contributions and on the size of the pro-reform lobby. Clearly, the slope of the curve will be different for low than for high levels of CA. Let CA be the level of anti-reform contributions that makes ½[(Φ/CA]½ equal to [1]. Then, for levels of CA below CA, the best response curve is upward sloping. For levels of CA above CA, the best response curve is downward sloping.
Observe in figure 1 that for values of CA below CA, lobby ρ responds to a more aggressive behavior of its rival (an increase in CA) with a greater contest effort (an increase in Cρ). Following the industrial organization literature,15 lobby ρ perceives anti-reform contributions as strategic complements. On the contrary, for levels of CA above CA, lobby p's best response to more aggressive lobby A's behavior is to reduce its general effort, so it perceives anti-reform contributions as strategic substitutes.
Using the same logit form for the probabilistic function, I turn to the anti-reform lobby maximization problem. The expected net benefit is given by:
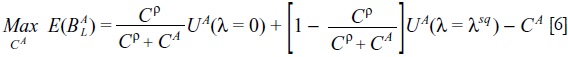
where:
UA(λ = 0): Expected utility received by A if the policy is approved.
UA(λ = λsq): Expected utility received by A if the policy is rejected.
UAλ > 0, UAλλ< 0.
The first order conditions of [6] show how the anti-reform lobby decides the total amount of contribution it will provide to Congress.
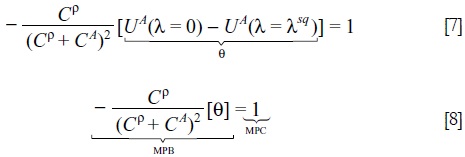
where: Φ = [UA(λ = 0) - UA(λ = λsq)] < 0, θ'(λ) > 0 and θ"(λ) < 0.
As expected, lobby A maximizes its expected net benefit by comparing MPB to MPC. Again, the assumption of zero lobby organizational cost leads to MPC equal to [1], while MPB depends on lobbies' contributions (Ci) and A's expected utility differential (θ) from alternative policies. Solving CA as a function of Cρ gives US the explicit best response function for the antireform lobby.

Like in the pro-reform lobby solution, if the rival decides not to contribute, A's best response will also be not to contribute. Hence the origin is a point on the reaction curve. To figure out the complete locus of points of A's best response curve, I totally differentiate [9] to obtain the slope of the reaction function:
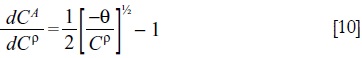
Once more, the slope depends on the utility differential of the lobby and on the rival's contribution level. While the utility differential of lobby A could also be related to its size, this relationship is not as direct as in the case of lobby ρ. Recall θ or the utility differential of the anti-reform lobby is by definition, a negative value. As a result, the size of the anti-reform lobby should be approximated with -θ instead of θ. This is crucial once we turn to the comparative static of the model.
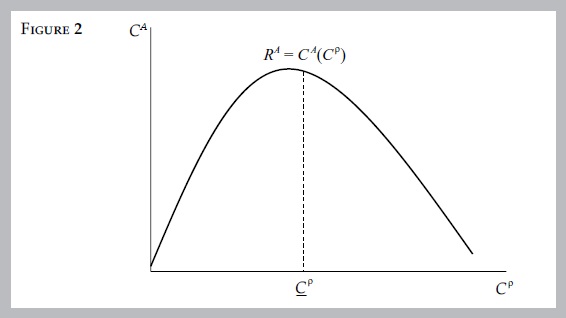
Let Cρ be the level of pro-reform contributions that equates ½[-θ/Cρ]½ to [1]. For levels of Cρ below Cρ, ½[-θ/Cρ]½ is greater than [1], so the slope is positive and the anti-reformer sees ρ's contributions as a strategic complement. On the other hand, for levels of Cρ that exceeds Cρ, the element ½[-θ/Cρ]½ is smaller than [1], the slope is negative and the anti-reformer sees ρ's contributions as a strategic substitute. The reaction curve of the anti-reform lobby, RA, is represented by the inverse U-shaped curve depicted in figure 2.
The next step is to find the equilibrium of the lobbying game or the point at which no lobby has an incentive to switch its position given its rival's location. The intersection of the two reaction curves defines such point. Recall equations [4] and [9], the best response functions of the lobbies, plugging one into the other I get the Nash equilibrium levels of contributions, Cρ* and CA*, as functions of Φ and θ. Keep in mind these parameters stand for the expected utility differentials or the size of the lobbies.
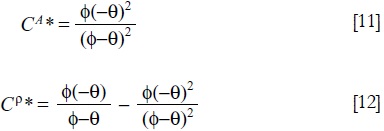
The Nash equilibrium is given by equation [13]:
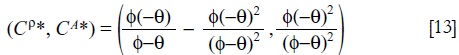
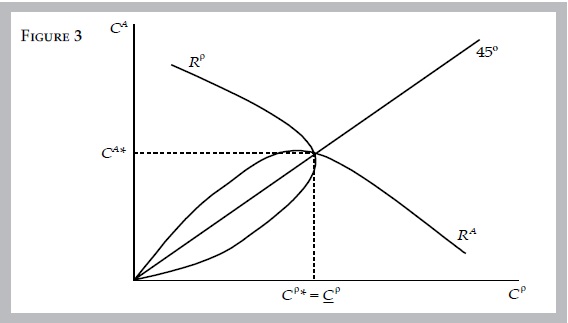
At equilibrium, Cρ* could be greater, equal or less than CA*. Hence, there are three possible diagrammatic representations. The first is the perfect symmetry case, depicted in figure 3, where both lobbies contribute exactly the same amount. In this case, equilibrium lies on a 45-degree line, containing the locus of points that satisfy Cρ* = CA*.
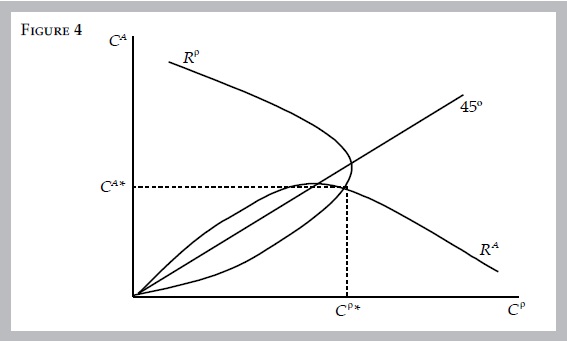
A second case, depicted in figure 4, shows the intersection of the reaction curves to the right of the perfect symmetry line. At equilibrium, Cρ* exceeds CA*. Following Dixit (1987) I argue that this equilibrium portrait the case where the pro-reform lobby is considered the favorite and the anti-reform lobby, the underdog, of the tariff contest.16 Baik and Shogren (1992) showed that for such a case the favorite's expected payoff E(BLρ) is decreasing when one moves up along its reaction curve. Thus, as contributions by lobby A increase the expected benefit of the pro-reformer decreases given Cρ.17
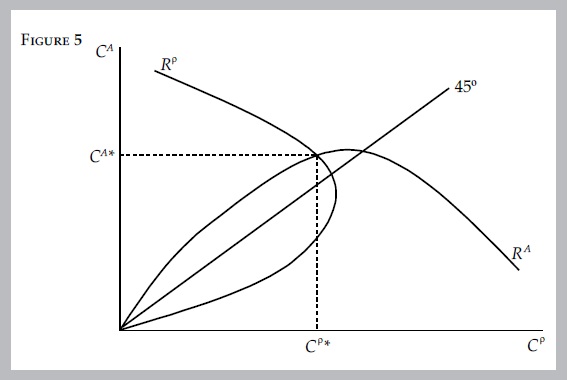
The third possible case arises when CA* exceeds Cρ* and equilibrium lies to the left of the 45-degree line. In such case, shown in figure 5, the anti-reform lobby becomes the favorite and the pro-reform lobby, the underdog, in the tariff contest. I emphasize results in this particular case, since it resembles the setting in which Congress discussed policy reforms in the DR in the mid-nineties. At that time, anti-reform groups, born under the ISI years, exerted a larger influence on policy issues than recently formed pro-reform groups.
Proposition I. When a reform-committed President faces a discrete choice between total reform and status quo and the probability of Congress approval assumes a logit form of function, the reduced-form lobbying game equilibrium depends on the size of the lobbies in such a way that:18
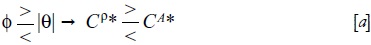
At the initial stage of policy discussion in the Dominican affair, |θ| exceeded ]), therefore CA* exceeded Cρ* and equilibrium resembled figure 5. A plausible interpretation of this is that as the membership of Lobby A exceeded that of Lobby ρ, the anti-reform lobby has more to lose than what its rival has to gain from the lobbying contest. Provided the historical background of protectionism in the DR, a reasonable argument is that loosing the privilege of high tariffs was tougher for those who enjoyed it for years, than what gaining liberalization represented for a pro-reform sector, which never had it before.
The other asymmetric case illustrated in figure 4 arises when Φ exceeds |θ| at the initial stage of policy discussion. This means, lobby ρis larger than lobby A and derives a greater amount of utility from total liberalization than what the anti-reform lobby loses. Although it is difficult to think of a case in ISI-developing countries where the pro-reform lobby is larger than the anti-reform lobby at the initial stages of liberalization discussion, this might occur in the middle of an economic and social crisis. Economic and social crises increase the number of individuals willing to bring down the status quo.19
Dixit (1987) explains that in any contest with a logit functional form, the favorite has a strategic incentive to over commit effort. In the case depicted in figure 5, lobby A will most likely overexert if precommitment is allowed.20 This outcome could be related to the Dominican reform process of the mid-nineties, where the anti-reform block overexerted even before the president submitted tariff reform to Congress. Since the asymmetric case, represented by figure 5 resembles the Dominican affair, let turn to the impact of changes in the size of the lobbies on this equilibrium.
Comparative statics: changes in lobbies' sizes
Recall equations [4] and [9], which stand for the best response functions of the pro-reform and the anti-reform lobbies, respectively. Also bear in mind that Φ could be related to the size of the pro-reform lobby and -θ, to the size of the anti-reform lobby. To ease interpretation in the comparative static exercise, let σ be equal to -θ. By definition σ represents the size of lobby A. Rewriting equation [9] to incorporate this definition leads to new reaction functions represented by [4] and [9']:
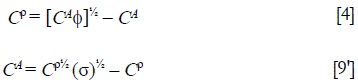
To find how changes in Φ affects equilibrium, I totally differentiate these best response functions and obtain a system of two equations with two unknowns.
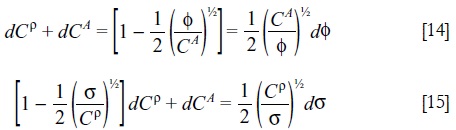
Rewriting the system in matrix form, I get:
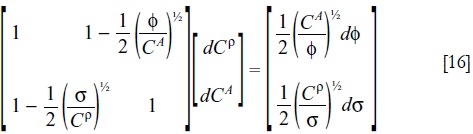
A sufficient condition for stability of the system described in [16] is that the first matrix on the left-hand side has a positive determinant. Hence, the system would be stable only if (Cρ/σ) > (CA/Φ). I assume this condition holds for the asymmetric equilibrium that resembles the Dominican case.21 The estimation of the determinant yields [17]:
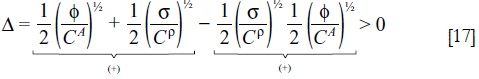
Holding σ constant and applying Cramer's rule, I get the effect of changes in parameter Φ over the different levels of contributions:
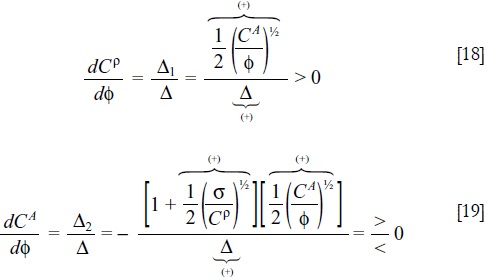
Proposition II. An increase in the size (utility differential) of the pro-reform lobby, Φ, holding constant the size of the anti-reform lobby, σ, leads to:
a) An increase in pro-reform contributions, Cρ.
b) An increase or decrease in the anti-reform level of contributions, CA, depending:b.1. On the original size of the anti-reform lobby, σ.
b.2. On the initial level of pro-reform contributions Cρ.
Intuitively, an increase of the membership of the pro-reform lobby implies that the number of individuals demanding liberalization increases, exercising pressure on lobby ρ to foster contributions. Will the anti-reform group compete (increase contributions)? The comparative static exercise suggests that A's willingness to compete depends on its relative size when equilibrium is reached. The smaller Cρ or the larger σ, the more likely an increase in Φ will lead to larger anti-reformer contributions. From [19], it is straightforward to obtain the condition under which the anti-reform lobby will compete.
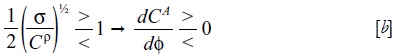
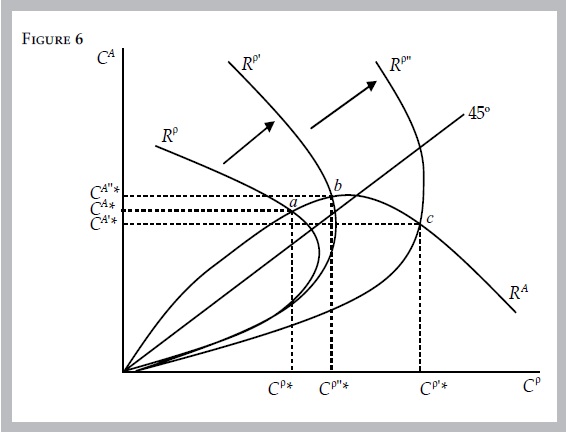
If the original equilibrium of the game is similar to that in figure 5, an increase in Φ shifts the reaction curve of ρ outward, in such a way that Cρ rises unambiguously, but the final effect on CA is unknown. Figure 6 shows these changes. Depending on the magnitude of the shift of Lobby ρ's reaction curve, anti-reform contributions will end up at a point like b, where they increase, or at a point like c, where they decrease. The equilibrium in point b illustrates the case where the initial size of lobby A was large enough to make it compete with the increase in pro-reform contributions. Point c, on the contrary, represents an equilibrium where initially σ was relatively small, reducing A's willingness to compete against a stronger adversary.
To find how changes in σ affects equilibrium, I set dΦ = 0 and apply Cramer's rule to the system of equations depicted in [16]. The outcome leads to proposition III.
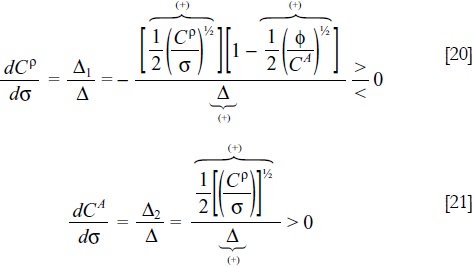
Proposition III. An increase in σ or the size of the anti-reform lobby, holding constant the size of the pro-reform lobby, Φ, leads to:
a) An increase in anti-reform contributions, CA.
b) An increase or decrease in the pro-reform level of contributions, Cρ, depending:b.1. On the original size of the pro-reform lobby, Φ.
b.2. On the initial level of anti-reform contributions CA.
Intuitively, an increase in σ or lobby A's membership leads to a greater demand for rejection of the policy. As a result, the anti-reform lobby boosts contributions to maintain the status quo. Will the pro-reform lobby compete? The reaction of lobby ρ depends on its relative size and the amount of contributions of A. For very high levels of Φ and/or low levels of CA, the pro-reform lobby reacts to the increase in the size of its rival, providing more contributions.22 The opposite happens for low levels of Φ or high levels of CA. These results are summarized in condition [c]:
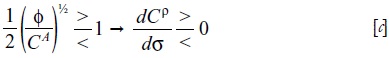
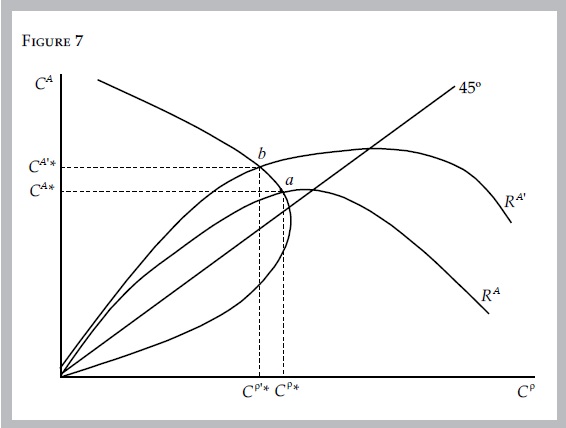
Figure 7 shows the effect of an increase in σ when the original equilibrium is such that CA* exceeds Cρ*. If originally, lobby ρ is not large enough (either Φ is very low or CA is very high), an increase in the size of the anti-reform lobby, σ, would increase CA and reduce Cρ. The new equilibrium is given by point b and CA" and Cρ* represent the new levels of contributions.
In the Dominican reform process of the mid-nineties, the reaction of the anti-reform lobby when public opinion turned against the reform was exactly like the one suggested in the model. One can conveniently argue that as public opinion turn to favor the status quo, the membership of the anti-reform block enhanced, exerting pressure to increase contributions.23 Since the reaction of the pro-reform lobby was to compete, the model hints that at the moment the proposal was made, the size of the pro-reform lobby was relatively large and/or the amount of contributions from lobby A were relatively low.
Recall from section III that the dawn of the pro-reform group came only after a splitting up of the anti-reform group during the first stage of reforms in the early nineties. Hence, it is plausible that five years after this partition, the size of the pro-reform lobby increased and the contributions of the anti-reform lobby decreased. Let's move to the overall game and the choice of the president.
The overall game: the final solution
Recall that the lobbying-contest is a reduced-form game within the overall game. In the general framework, the president, P, leads the lobbies and fits into the expected net benefit function from sending or not a proposal to Congress, the equilibrium levels of contributions, Cρ* and CA*. Such function is described in [22]:

When proposing P faces a fixed cost, F. A convenient way to think about F is as the cost of elaborating and organizing a proposal. Alternatively, one can think of F as the opportunity cost P copes with when distracted from other activities. Due to this cost, a proposal is not free of sacrifice. Therefore, in some cases P will rather keep the status quo. Moreover, the president decision depends on a comparison between the expected gains of proposing vis-à-vis the expected gains of no proposing. Rewriting [22], I set up the conditions under which P will propose:

where:
ψ = [UP(λ = 0) - Up (λ = λsq)]; q* = Cρ*/(Cρ*+ CA*)
UP(0): Expected utility for the president if policy is approved.
Up (λsq): Expected utility for the president if status quo is maintained.
Proposition IV. If q*ψ is greater (lower) than F, P's expected net benefit is positive (negative) and therefore he will propose (not propose).
Given that both, F and ψ, are constants, P's proposal depends directly on q*, a probability determined in the lobbying game. Recall q*=Cρ*/(Cρ*+CA*). Therefore, the equilibrium levels of lobbies' contributions influence P's decision. Intuitively, P is a self-driven politician and cares about the final outcome in Congress. P sees a policy approval as a political triumph. Furthermore, he is aware that such approval rests on the contribution of opposing lobbies. Accordingly, he monitors the lobbying contest before deciding whether proposing or not.
Since the size of the lobbies determines contributions, it is crucial to understand how these parameters affect P's decision. Equation [13] embodies the Nash equilibrium of the lobbying contest as a function of parameters )) and θ. To conveniently accommodate of these parameters to the size of the lobbies, again I use σ = -θ in the Nash equilibrium result, obtaining equation [13']:
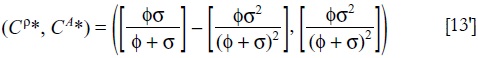
Plugging [13'] into [23] allow us to rewrite the expected net benefit the president get from proposing or not, in terms of the relevant parameters.
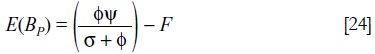
Differentiating equation [24] with respect to Φ and σ yields equations [25] and [26]. These equations show how a change in the size of the pro-reform lobby (Φ) or in the number of affiliates of the anti-reform lobby (σ) affects the expected net benefit of the president.
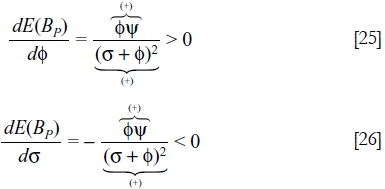
Proposition V. An increase in the size of the pro-reform lobby, Φ, increases the expected net benefit of the president and his willingness to propose. An increase in σ or the size of the anti-reform lobby decreases the president's expected net benefit and his willingness to propose.
Intuitively, a larger constituency of the pro-reform lobby exerts more pressure for contributions, increasing lobbying activity in favor of liberalization and eventually, the probability of Congress' approval. Provided it is more likely the reform will pass, P's willingness to propose increases. Similarly, an increase in σ or a larger membership of the anti-reform lobby increases CA and hence, the probability that the reform will be rejected in Congress. As a result, P's willingness to propose decreases.
Proposition VI. Let (Φ) and σ be the levels of (Φ) and σ that makes the expected net benefit of the president, E(Bp), equal to zero. Then, for a given fixed cost F and utility differential, ψ:
a) Values of Φ below Φ or values of σ above σ makes q*ψ< F, so the expected net benefit for the president becomes negative and there is no proposal.
b) Values of Φ above Φ or σ below σ makes q*ψ> F, so the expected net benefit for the president becomes positive and he proposes to Congress.
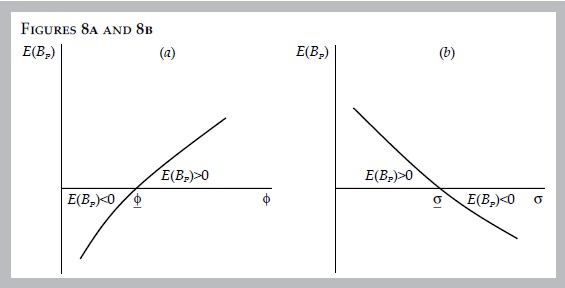
Figures 8a and 8b summarize the findings presented in proposition VI. The slopes of [25] and [26] give the shapes of the curves.
Notice that (Φ) and σ constitute minimum and maximum sizes of the respective lobbies after which P will make a proposal. These sizes depend, at the same time, on the actual sizes of the lobbies. For instance, an increase in the size of lobby A, σ, decreases the expected net benefit of the president and his willingness to propose. With a larger A, the president requires a larger minimum size of ρ to propose. Hence, Φ becomes larger. Using a similar reasoning, one can argue that a is affected by changes in the actual size of the pro-reform lobby, Φ.
Proposition VII. Let Φ = H(σ) and σ = J(Φ), then H'(σ)>0 and J'(Φ)>0. An increase in the size of the anti-reform (pro-reform) lobby leads to an increase in the minimum pro-reform (maximum anti-reform) lobby under which P will propose.
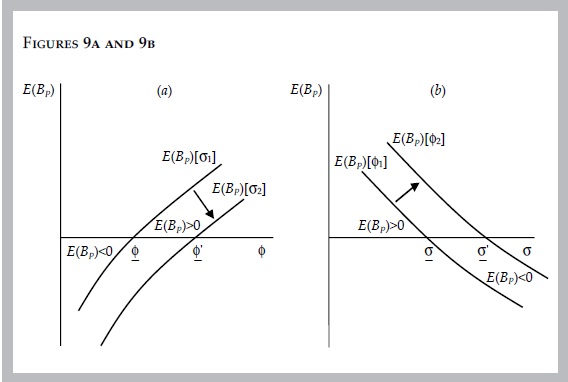
Figure 9a illustrates the effect of an increase in σ on P's expected net benefit. As a result of this increase, the E(BP) curve shifts to the right, leading to a situation where P receives a lower expected net benefit per size of the pro-reform lobby,Φ.
Intuitively, a larger constituency of lobby A has more to lose in case the reform is enacted. Hence, it contributes more, reducing the probability of Congress approval. P is aware it is less likely the reform will pass. Thus, the minimum size of the lobby ρ after which the president proposes increases.
Figure 9b, on the other hand, shows the impact of an increase in the actual size of the pro-reform lobby, Φ, on the maximum A's size after which P will propose. A larger Φ shifts the expected net benefit curve outward, increasing σ to σ'. Intuitively, an increase in the number of individuals supporting reform makes P to propose in a wider range of cases than before.
The sizes of the lobbies and the actual probability of reform
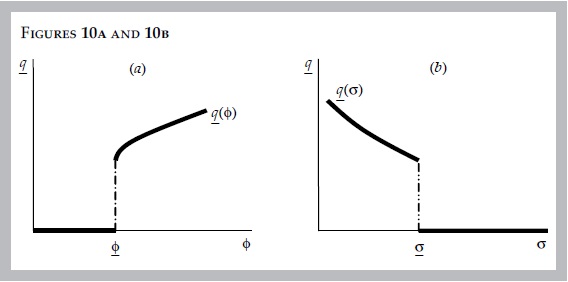
Let q be the actual probability that P will propose. Since values of Φ below Φ and σ above σ yield a negative expected net benefit for P, q must be zero in this range of values. For the same reasons, values of Φ above Φ and a below a generate a positive expected net benefit for P and hence a positive q. Moreover, for such lobbies' sizes, q should be exactly equal to the probability of Congress approval, q.
Figure 10 illustrates the relationship between the actual probability of reform, q, and the parameters that define the size of the lobbies, Φ and σ. The shape and curvature of the graphs for levels of Φ above Φ and σ below σ are given by equations [27] to [30]:

Being q the actual probability of reform, it is of the essence to find out how small changes in the size of the lobbies affect it, when σ is close to σ or Φ is close to Φ. In other words, it is necessary to explain why there is a jump in q like those observed in figures 10a and 10b. While P is playing status quo, the dominant strategy for values of Φ below Φ and values of σ above σ, the lobbies are continuously contributing to Congress amounts that correspond to lobbies' sizes below Φ or above σ.
As a result of these contributions, Congress is more willing to approve the reform than otherwise would be. Hence, the probability of Congress approval is already positive and becoming larger as σ and Φ move toward σ and Φ. As soon as the game reaches these values, the president proposes. At this stage, the actual probability of reform, q, becomes equal to the probability of Congress approval, q, which is already is very high.
Conclusion
Drawing from the Dominican Republic's reform experience of the mid-nineties, this paper presented a model where a President, committed to reform, is allowed to choose between total liberalization and status quo. Unless the opportunity cost of reforming is very high, the president will opt for liberalization. In his reform attempt, the president leads two opposing lobbies, which play a simultaneous move lobbying game.
The reduced-form lobbying game yields three possible unique equilibrium, depending on the relative size of the lobbies. A Nash equilibrium where the membership of lobby A exceeds the number of affiliates to lobby ρ is more representative of the Dominican reform process. Despite the equilibrium chosen, an increase in the size of the membership of a lobby increases its own contributions. It also increases or decreases the contributions of its rival depending on the relative size of its membership and the initial level of contribution of the lobbies. The model shows a committed President will be more (less) willing to propose the greater the size of the pro-reform (anti-reform) lobby.
Finally, I was able to show the existence of minimum and maximum lobbies' sizes, ) Φ and σ, after which the president proposes. A proposition implies a cost for the president when he is distracted from other important issues. Therefore, the president will propose only if his allied, the pro-reform lobby, is large enough to compete with his adversary, the anti-reform lobby.
As part of a future research agenda, some extensions or modifications of the model could be considered. On one hand, the decision-making process of Congress could be endogenized through the definition of an optimization function for a representative Congressman. Within this setting, Congress' decision could be a function of lobbies' contributions and other factors such as political costs. On the other hand, the model could be transformed by stripping the president from its leading role. A possible assumption is a weak president, uncommitted with economic reform, who is led by either lobbies or Congress. Within this Stackelberg setup, the president will be a follower replicating discussion processes in countries with either powerful and well-organized interest groups or a strong legislature.
Another possible variation is to endogenize the parameters that define the sizes of the lobbies. This could enrich the model and lead to quite different results, particularly in the way the sizes of the lobbies determined contributions and ultimately, modifying the president's decision.
A final comment related to the research agenda is of the essence. While I highlighted some possible extensions or modifications of the model, it is crucial to admit that there are many more possibilities. A reason for this is that research on the political economy of policy reform in developing countries is relatively new. Most of the political economy research on reform approval has been written for industrialized nations, where political rules are clearer and easier to model. Despite limitations inherent to political ruling in developing countries, I must admit that the incipient political economy literature for these types of nations has contributed substantially to organize the thinking on the politics of public policies. In building this model, such was my goal for the Dominican case.
References
Alesina, A. and A. Drazen, "Why is Stabilization Delayed?", American Economic Review, vol. 81, no. 5, December 1991, pp. 1170-1188. [ Links ]
Andújar-Scheker, J. G, "Reformas Económicas y Negociaciones Políticas: Apuntes sobre la Experiencia Dominicana de los Noventa", Ciencia y Sociedad, vol. 30, no. 1, enero-marzo 2005, pp. 7-57, [ Links ].
Arrow, K., Social Choices and Individual Values, New Haven, Yale University Press, 1951. [ Links ]
Baik, K.H. and J.F. Shogren, "Strategic Behavior in Contests: Comment", American Economic Review, vol. 82, no. 1, March 1992, pp. 359-362. [ Links ]
Brock, WA., S.P. Magee and L. Young, Black Hole Tariffs and Endogenous Policy Theory: Political Economy in General Equilibrium, Cambridge, Massachusetts, Cambridge University Press, 1989. [ Links ]
Bruton, H., "A Reconsideration of Import Substitution", Journal of Economic Literature, vol. 36, no. 2, June 1998, pp. 903-936. [ Links ]
Buchanan, J. and G. Tullock, The Calculus of Consent, Ann Arbor, Michigan, The University of Michigan Press, 1962. [ Links ]
Bulow, J., J. Geanakoplos and P. Klemperer, "Multimarket Oligopoly: Strategic Substitutes and Complements", Journal of Political Economy, vol. 93, no. 3, 1985, pp. 488-511. [ Links ]
Cardoso, E. and A. Helwege, Latin America's Economy: Diversity, Trends and Conflicts, Cambridge, The Massachusetts Institute of Technology (MIT) Press, 1997. [ Links ]
Dasgupta, A. and K. Nti, "Designing an Optimal Contest", in European Journal of Political Economy, vol. 14, 1998, pp. 587-603. [ Links ]
Dinopoulos, E. and C. Syropoulos, "International Diffusion and Appropriability of Technological Expertise", in M.R. Baye (ed.), Advances in Applied Microeconomics, vol. 7, JAI Press, 1998, pp. 115-137, [ Links ].
Dixit, A., "Strategic Behavior in Contests", American Economic Review, vol. 77, no. 5, December 1987, pp. 891-898. [ Links ]
Downs, A., An Economic Theory of Democracy, New York, Harper and Row, 1957. [ Links ]
Drazen, A. and V Grilli, "The Benefits of Crises for Economic Reform", American Economic Review, vol. 83, no. 3, June 1993, pp. 598-607. [ Links ]
Finlay, R., "The New Political Economy: Its Explanatory Power for LDCs", in G. Meier (ed.), Politics and Policy Making in Developing Countries: Perspectives on the New Political Economy, San Francisco, California, International Center for Economic Growth Press, 1991, pp. 13-40. [ Links ]
Helpman, E. and T. Persson,. "Lobbying and Legislative Bargaining", Advances in Economic Analysis & Policy, vol. 1, no. 1, 2001, pp. 1008-1008. [ Links ]
Hirschleifer, J., "Conflicts and Rent Seeking Success Functions: Ratio vs. Difference Models of Relative Success", Public Choice, no. 63, 1989, pp. 101-112. [ Links ]
Inter-American Development Bank (AIDB), Latin America After a Decade of Reforms, Economic and Social Progress in Latin America, Washington, D.C., AIDB, 1997. [ Links ]
----------, The Politics of Policies, Economic and Social Progress in Latin America, Washington, D.C., AIDB, 2006. [ Links ]
Loury, G., "Market Structure and Innovation: A Reformulation", Quarterly Journal of Economics, vol. 93, no. 3, March 1979, pp. 395-410. [ Links ]
Meier, G.M. (ed.), Leading issues in Economic Development, New York, Oxford University Press, 1995. [ Links ]
Moya Pons, F., Empresarios en Conflicto: Política de Industrialización y Sustitución de Importaciones en la República Dominicana, Santo Domingo, Amigo del Hogar, 1992. [ Links ]
Mueller, D.C., The Public Choice Approach to Politics, Brookfield, United States, E. Elgar, 1993. [ Links ]
Nelson, D., "Endogenous Trade Theory: A Critical Survey", American Journal of Political Sciences, vol. 32, no. 3, August 1988, pp. 796-827. [ Links ]
Nitzan, S., "Modeling Rent-Seeking Contest", European Journal of Political Economy, no. 10, 1994, pp. 41-60. [ Links ]
Olson, M., The Logic of Collective Action, Cambridge, Harvard University Press, 1965. [ Links ]
Pant, H.M., Tariff Determination in the General Equilibrium of Political Economy: A Bargain-Theoretic Approach to Policy Modeling, England, Ashgate Publishing Co., 1997. [ Links ]
Rodrik, D., "Understanding Policy Reform", Journal of Economic Literature, vol. XXXIV, March 1996, p. 9-41. [ Links ]
Rosen, S., "Prizes and Incentives in Elimination Tournaments", American Economic Review, no. 76, September 1986, pp. 701-715. [ Links ]
Skaperdas, S., "Cooperation, Conflict and Power in the Absence of Property Rights", American Economic Review, no. 82, 1992, pp. 720-739. [ Links ]
----------, "Contest Success Functions", Economic Theory, no. 7, 1996, pp. 283-290. [ Links ]
Stiglitz, J. and P. Dasgupta, "Uncertainty, industrial Structure and the Speed of R & D", Bell Journal of Economics, no. 11, Spring 1980, pp. 1-28. [ Links ]
Tirole, J., The Theory of Industrial Organization, Cambridge, The MIT Press, 1988. [ Links ]
Tommasi, M. and F. Sturtzenegger (eds.), The Political Economy of Reform, Cambridge, The MIT Press, 1998. [ Links ]
Williamson, J. (ed.), The Political Economy of Policy Reform, Washington, D.C., Institute for International Economics, 1994. [ Links ]
The author would like to thank Devashish Mitra, Ali Cem Karayalcin, Nejat Anbarci, Diego Méndez-Carbajo, Rolando Guzmán, Frank Fuentes, Dayana Lora, Apolinar Veloz, Rodrigo Fuentes, Manuel Amador and two anonymous referees for comments on an earlier version of this paper. Opinions, however, are responsibility of the author.
1 A restricted list of first stage reforms would include fiscal discipline, trade liberalization, securing of property rights, openness to foreign direct investment, tax reform, privatization, exchange rate unification, financial liberalization, deregulation and public expenditure reorientation. See Williamson (1994).
2 Inter-American Development Bank (1997) shows that issues related to the electoral systems such as the degree of government fragmentation, the number of parties represented in the legislature and the ability of minority to obtain political representation, are instrumental in shaping political outcomes.
3 Formally, the Dominican Republic has been a democracy since 1966. For many years, however, it has retained its authoritarian ways on account of institutional weaknesses such as, loopholes in the electoral system and a constitutional flaw that have granted special powers to the president. As a result of these weaknesses, previous reform attempts in the Dominican Republic have passed either with unconditional Congress' support or through presidential decrees.
4 See Rodrik (1996).
5 Frequently, the endogenously determined policy is a tariff, so EPT is known as Endogenous Tariff Theory. Nelson (1988) presented a complete critical survey of this literature.
6 This definition is in Dasgupta and Nti (1998), p. 587.
7 CSF was axiomatized by Skaperdas (1996).
8 See Meier (1995), Cardoso and Helwege (1997), Bruton (1998) and Rodrik (1996).
9 Cardoso and Helwege (1997) explains how, with the exception of a few countries that achieved some openness under military regimes (Argentina, Chile and Uruguay), the rest of la maintained ISI policies despite a Gross Domestic Product (GDP) contraction of 0.8% in the period 1980-1989.
10 Reader interested in why and how US Authorities took over customhouses is referred to Andujar-Scheker (2005).
11 Special concessions consisted mainly on domestic tax exemptions provided to the few big industries operating in the economy at the time (Moya Pons, 1992).
12 Recall policy reforms in the DR is the key driver of modelling in this paper. As mentioned earlier, the DR Constitution grants enormous discretionary power to the president, limiting the extent of democracy.
13 I am aware of the limitations of choosing this type of function. In axiomatizing the logit function, Skaperdas (1996) assumes that the winning probability of each player in a contest depend on the difference in efforts. In our model, this accounts for q depending only on the size of the difference in contributions. This strong assumption suggests that q should be equal for a case where CA = 10 and Cρ= 100 and a case where C = 1 000 000 and Cρ = 1 000 090.
14 Specifically, total lobbying costs equate the total amount of contributions.
15 See Tirole (1988) and Bulow etal. (1985).
16 Being a favorite in the lobbying contest implies having a probability of winning the prize that exceeds 50% in the Nash equilibrium.
17 In this setting, whenever the anti-reform lobby increases or its level of effort, the probability of Congress' approval q decreases, therefore reducing the pro-reformer's expected payoff.
18 The algebraic manipulation to obtain condition [a] is available upon request to the author.
19 The correlation between crisis and reform is not unknown to the economic literature. Drazen and Grilli (1993) suggest that crisis enable societies to enact measures that would be impossible to enact in normal conditions. Alesina and Drazen (1991) explain the reasons why this happens.
20 Overexerting is a plausible outcome as long as we do not endogenized the order of moves between the two players. Baik and Shogren (1992) found that by endogemzmg the order of moves between a favorite and an underdog, the latter will always move first and the former, second. With this order of moves instead of overexerting both players will under exert with respect to the Nash equilibrium.
21 This is not a strong assumption since the size of the pro-reform lobby, for countries that followed an import-substituting development strategy, is larger than the size of the anti-reform lobbies.
22 The reader must be aware that depending on the original equilibrium, the comparative statics exercise could yield results where the smaller lobby (pro-reform, in this case) contributes more than the larger lobby. A possible explanation for this situation could be a serious free-riding problem in the larger lobby. Another explanation could be larger efficiency gains for the smaller than for the larger lobby due to reform approval.
23 Initially, the National Council of Businessmen (CONEP), former CNHE, represented the anti-reform block in the DR process. As public opinion turn against the reform, several organized groups jumped into the anti-reform wagon increasing the size of the lobby.