1.Introduction
Many research efforts have been devoted to positive-negative ion plasma, composed of ions with negative and positive charges and electrons, since it has an important role in studying various plasma science fields. This kind of plasma can be found in low-temperature laboratory experiments and many astrophysical plasma environments 1-8. The (e-p) plasma, which contains positrons and electrons, can also include positive ions. This (e-p-i) plasma is created in the laboratory and also found in many astrophysical contexts like galactic nuclei and others (see 9-11). The (e-p-i) plasma model in the presence of negative ions was discussed in many other works as in Ref. 12-16. The predicted nonlinear excitations that occur in e-p-i plasmas may include electromagnetic solitons, electrostatic oscillations solitons, which depending on the nonlinear plasma in magnetized or unmagnetized cases, including the Zakharov-Kuznetsov (ZK), Korteweg-De Vries (KdV), and the nonlinear Schrodinger (NLS) equations 17,18.
The ZK equation has great importance in studying the propagation of acoustic waves in the magnetized plasma 19,20. Various methods were used for obtaining many types of solutions to the nonlinear evolution equations (N.L.E), such as the extended tanh method, (G´/G) method, the general expansion method, and the extended homogeneous balance (ext.HB) method 21-29. Wang et al. had proposed the HB method, which is an effective algebraic method for extract a wide class of analytical solutions 30,31. Many N.L.E. had been solved using the extended homogeneous balance. In this paper, the ext. HB method has been used to solve the ordinary differential equation (O.D.E.) reduced from the ZK equation with computer algebra system such as Mathematica to extract various kinds of analytical solutions for ZK equation, the obtained solutions have new solutions and cannot be obtained by many other methods like tanh, extended tanh, G´/G expansion methods.
Many researchers have studied the nonlinear propagation of the ion-acoustic waves for a two-component plasma consisting of classical ions and electrons. Owing to the importance of pair-ion plasma, which is composed of positive and negative ions, as well as the study of the ion-acoustic nonlinear solitary waves for magnetized plasma, it is of paramount interest. In the present work, we have studied the nonlinear properties of the propagation ion-acoustic (IAW) solitary waves in positive-negative ion plasma with positrons and electrons. The ZK equation is derived and has been solved using the ext.HB method to study the small-but-finite amplitude solitary wave. The effects of various physical parameters have been checked on the characteristics of the solitary waves in such plasma, which can be found in many astrophysical plasma systems as in the Earth’s ionosphere.
The paper is organized as follows: In the following section, the governing equations for the suggested plasma model are presented, and the ZK equation is derived to describe the system. The extended homogenous balance method is used to solve the ZK equation in Sec. 3; different kinds of solutions are obtained as periodic, rational, blow up, and solitary wave solutions, while we focus on the solitary type solution. The discussion is presented in Sec. 4, and a summary of these results is presented in the last section.
2.Model equations and ZK equation
The suggested magnetized multi-component plasma model consists of electrons,
positrons, and fluid ions with positive and negative charges within a magnetic field
The Poisson equation
while positrons and electrons distributions are given by
Such that sp = Tp/Te, where Tp and
Te are the positron and electron temperature, respectively. The mass
ratio is defined as
where
where λ is the wave velocity and
where
The phase velocity relation can be deduced from Poisson equation
The next-order in
The coefficients of nonlinear and dispersion terms are given as
3.Solution of ZK equation
Now, we shall illustrate the approach of the ext.HB method to obtain a class of exact solutions, including the solitary wave kinds for the ZK equation.
Let us first consider the ZK equation
we can reduce ZK equation to an O.D.E., using the transformation
where
By balancing the highest order with the highest degree in the last equation, we can see that the solution should be in the form
with
where αi and b
i
are constants, while k, M, and P are the parameters to be determined latter,
It is noted that equation (*) is the Riccati equation, which can be solved to give us many forms of solutions depending on the following cases (see for more details):
Case I: when P = 1, M = 0
Cases (II, III, and IV): when
By substituting (22) in the integrated O.D.E., we obtain an equation in ω. Then, the coefficients of ωi(i = 0,1,2,…) will be equated to 0 to get a system of algebraic equations that can be solved using MATHEMATICA, to give us:
The first set:
The second set:
In Ref.32, Abdelsalam et al., the method was explained in detail, and four cases regarding the Riccati equation were discussed. Here, these cases are not discussed as full details of these four cases are already published. Hence, for the first set, we can obtain case I solutions with M = 0, P = 1, these solutions (for ZK equation) are: for k > 0,
and
while for k < 0
for k = 0
Now, the compatibility condition for these solutions in the three other cases (II & III & IV) is
Substituting from the values in the first set in the last equation to get a relation for p i :
which helps us to obtain the following solutions:
For case II:
and
For case III, the solution will be
with the condition that p1 = 1.
However, the case IV solutions are
with the condition that p1 = 1, and
with the condition that p1 = 2.
For the second set, there no solutions are satisfying case I. Similarly, we can obtain for the second set
Hence, the obtained solutions for ZK Eqn., will be
with condition p1 = 1,
for p1 = 1 , and
for p1 = 2.
4.Numerical results and discussion
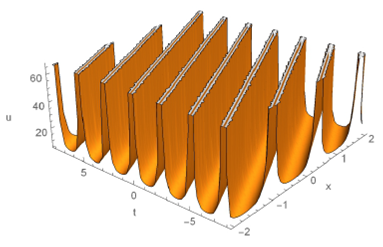
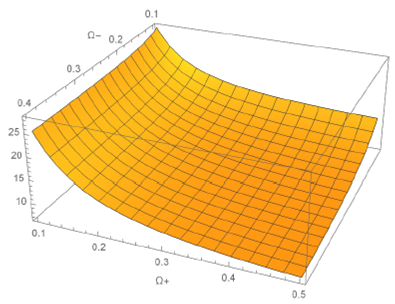
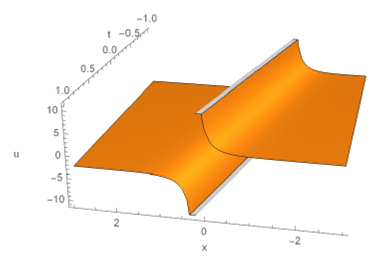
To investigate the nonlinear dynamics of IAWs, the reductive perturbation technique is used to obtain the ZK equation for a collisionless, four-component magnetized plasma model composed of negative and positive ions with electrons and positrons. The ext.HB method has been used to obtain various types of traveling wave solutions for ZK equation as periodical, singular, rational, and solitary wave solutions. For example, Eq.(25) represents a periodical solution as depicted in Fig.1, and it is clear that the solution (29) is a rational-type solution. In Fig.2, we can notice that the solution (33) is an explosive/blowup solution, while the balance between the dispersion term and the nonlinear term in the PDE gives the soliton solution represented in (27), the balance between nonlinearity and dispersion may be disturbed by plasma quantities variations (e.g. density, pressure, temperature, etc).
Now, to study the properties of the ion-acoustic waves IAWs, we should focus on the soliton wave pulses represented in (27),
where the amplitude of the soliton pulse is expressed as
In Fig. 3, we notice that by increasing the
electron concentration (ηe), the values of the soliton pulse amplitude
and width will decrease, while in Fig. 4, we
can see the role of the electron and positron temperature in variation the profile
of the soliton pulse, it is clear that increasing sp makes the soliton
pulse shorter but has no effect on the width of the wave shape. In Fig. 5, it is clear that the soliton shape is
taller and wider by increasing the negative ion concentration μ. However, increasing
the values of the magnetic field
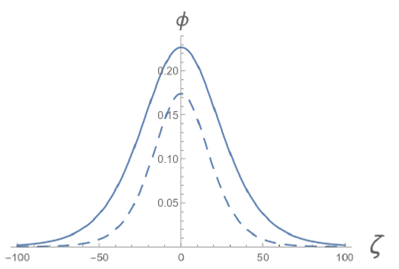
Figure 3 The variation of solitary wave profile for η e = 0:4 (solid line) and η e = 0:6 (dashes line).
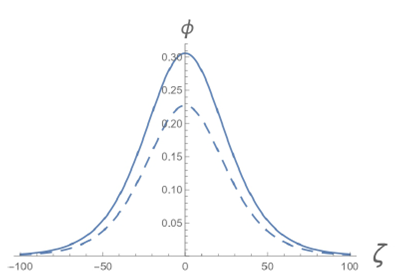
Figure 4 The variation of solitary wave profile for s p = 0:8 (solid line) and s p = 0:84 (dashes line).
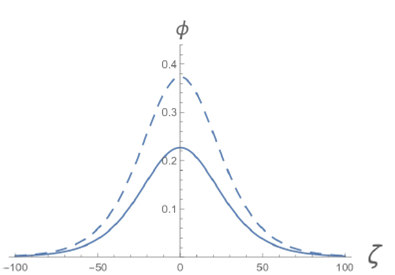
Figure 5 The variation of solitary wave profile for μ = 0:88 (solid line) and μ = 0:9 (dashes line).
Physically, when the amplitude increases, then the nonlinearity increases and vis versa. When the physical parameter increases, the amplitude then more energy is pumped into the plasma system, and the nonlinearity increases. We can notice that when the negative ion concentration μ increases, more energy is pumped into the plasma, which leads to enhance the amplitude.
5.Summary
In this study, the properties of the IAWs have been investigated for four-component magnetized plasma contains fluid ions with negative and positive charges in addition to electrons and positrons. The solitary pulses have been described by the ZK equation, which is solved analytically using the ext.HB method. The used method extracts many types of traveling solutions as periodical, singular, rational, and solitary wave solutions. The ZK equation has new solutions that cannot be obtained by many other methods like tanh, extended tanh, G´G expansion methods. By the numerical analysis of the obtained solitary wave solution, the effects of some physical parameters have been checked to the propagation and the shape of the produced acoustic waves. The increase of the concentration of electrons makes the pulse shape shorter and narrower, while it will be taller and wider by increasing the negative ions, and we can see that the magnetic field affects only the width of the solitary pulse. However, the effect of the electron-to-positron temperature ratio will be on the amplitude of soliton pulse shape. The present study should be helpful to understand the properties of the nonlinear IAWs in laboratory plasmas and many astrophysical plasma systems as in Earth’s ionosphere.











 nueva página del texto (beta)
nueva página del texto (beta)