1. Introduction
Nonlinear evolution equation systems are commonly employed as models to explain physically complex events in several fields of sciences, particularly in solid-state physics, fluid mechanics, chemical physics, optical physics, plasma physics, etc. Having a better comprehension of the underlying events together with their advanced applications in theoretical studies and simple usage in practical life, it is highly significant to find exact solutions of given systems. Although there exists no general and unified method to determine exact solutions for each nonlinear evolution system researchers use a variety of different approaches ranging from the truncated Painleve expansion [1], Darboux transformation method [2], the inverse scattering transformation method [3], homogeneous balance method [4], Backlund transformation method [5], Jacobi elliptic functions method [6], projective Riccati equation method [7], Hirota's bilinear method [8] to many other methods.
One of the important nonlinear evolution equation systems encountering in the differential geometry is known as geometric flow. This flow can be obtained through the curvature or binormal motion of space curves concerning the time parameter. Curvature motion implies that a curve moves in the direction of a normal vector proportional to its curvature. This type of evolution equation contains many notable geometric partial differential equation systems. These equation systems are mainly classified as average mean curvature flow, mean curvature flow, Willmore flow, and surface diffusion flow. Some of these evolution systems have a particular solution under some circumstances, for instance, Willmore flow leads surfaces whose squared to mean curvature is minimal and the mean curvature flow yields to minimal surfaces [9]. Binormal motion, however, implies that a curve moves in the direction of binormal vector proportional to its curvature. This type of evolution equation includes detailed information related to geometric and dynamical features of curves. For example, Balakrishnan et al. [10] demonstrated a possible connection between the soliton evolution and geometric phase, which stems from the rotational path dependence of Frenet-Serret orthonormal frame concerning Fermi-Walker non-rotating frame, by using the binormal motion of curves. Scrodinger flow is also induced by the binormal motion. The generalization of the universal theory of Schrödinger flow in Riemannian manifold, symplectic manifolds, Kahler manifolds, para Kahler manifolds, and other structures were intensively investigated in the literature by many researchers [11, 14].
The concept of Berry phase or geometric phase in quantum systems has gained much attention following the pioneer study of Berry [15]. Berry proved that a quantum system depending on some parameters and evolving in time can pick up a topological phase in addition to the usual dynamical phase. This topological phase is related to the motion of the quantum system in the space. This result has become the center of several other experimental and theoretical investigations relating the other geometric events in physics to Berry phase. The remarkable example of such a situation is involved in the motion of a charged point-particle in the existence of a time-dependent magnetic vector field, since an extra phase is gained by the point-particle depending on the geometry of the magnetic field. Another classical example for that case can be observed on the propagation of polarized light along an optical fiber. In particular, Ross [16], Kugler and Shtrikman [17] have focused on the geometric nature of the rotation of polarization in the optical fiber, by considering the fiber as a space curve showed that the phase dependence of this phenomenon can be explained in terms of parallel transportation along the optical fiber. The geometric phase of the optical vortices in coiled optical fibers were studied by Alexeyev and Yavorsky [18].
The organization of the paper is as follows. Section 2 is devoted to present brief information about the geometry of the unit 2-sphere S2 to provide the basic background. The goal of the third section is to supply a somewhat more fundamental differential geometric approach to compute the geometric phase of the polarized light ray, traveling along with the coiled optical fiber lying on the unit 2-sphere S2. In Sec. 4 it is aimed to clarify the geometric nature of the electric field and magnetic field vectors of the polarized light ray along with the coiled optical fiber. Investigating the relation between the evolution equations of the electric field and magnetic field vectors of the polarized light ray traveling in a coiled optical fiber and NLS equations is the subject of Sec. 5. In Sec. 6 we propose perturbed solutions of the nonlinear Schrodinger's evolution equations that govern the propagation of solitons through the electric field (E) and magnetic (M) field vectors.
2. Preliminaries
The characterization of the motion of particles via a space curve is a very efficient method to comprehend many physical events. These events can be modeled by connecting the motion of the particle with a space curve in a given spacetime.
Ordinary space is one of best fitted geometric settings for many physical phenomena, such that it has been intensively studied by both differential geometers and physicists. Since the unit 2-sphere is a submanifold of the ordinary 3-space, we firstly give geometry of the curves in the ordinary 3-space.
Ordinary 3-space is a vector space endowed with a standard metric
where π = (π1, π
2
, π
3), θ = (θ1, θ
3
, θ
3) ∈ ℝ3 are arbitrary vectors in the space. The norm function of a vector π is given by ||π|| =
Now, we can present a geometric definition of the unit sphere 𝕊2 as the analog of the ordinary 3-space in the following manner.
Hereafter, we consider a smooth regular curve lying fully on the unit 2-sphere. In the theory of differential geometry, one of the most efficient ways of exploring the intrinsic feature of the curve is to consider its orthonormal frame. It is constructed by several orthonormal vectors and associated curvatures depending on the dimension of the space. The curve satisfying the spherical frame equation is called a spherical curve. Finally, we are ready to establish the orthonormal frame of spherical curves lying fully on the unit 2-sphere.
Let Ψ : 𝕀 → 𝕊2 be a unit speed regular spherical curve, that is it is an arc-length parametrized and sufficiently smooth. Then the spherical frame is defined along the curve Ψ as follows
where μ = det (Ψ, t, tv) is the geodesic curvature of Ψ. The vector product of spherical vector fields is given by
For more details, see [19] .
3. A geometric phase of the polarized light ray propagating along with a coiled optical fiber on the unit 2-sphere 𝕊2
In this section, we mainly focus on the behavior of the polarization of light traveling along with a coiled optical fiber on the unit 2-sphere 𝕊2. We aimed to obtain the geometric phase of the polarized light through Fermi-Walker parallel transportation law and spherical coordinate system in various situations.
We begin by describing notations and definitions of the polarized light ray coupling into a coiled optical fiber lying on the unit 2-sphere S2. Here, the polarized light ray is supposed to arc-length parameterized and the arc-length parameter is denoted by v. It is also assumed that each ray corresponds to a space curve for a given specific refractive index function N(Ψ), which is the solution of the following differential equation.
From now on, we shall suppose that there exists a sufficiently smooth space curve θ : Ψ = Ψ(ν) satisfying the Eq. (3) and standing for a ray of light traveling in a coiled optical fiber.
Our primary goal is to observe the evolution of the electric field vector concerning arc-length parameter ν as light ray propagates along the coiled optical fiber. Now, let E represents the normalized complex electric field vector having a three-component of the spherical triad at the point Ψ(ν) on θ i.e.
Case 1. In the first case, the normalized complex electric field vector E is assumed to lie on a plane perpendicular to t. As a consequence of Maxwell's equations, the evolution of the electric field vector E concerning ν obeys the following formula due to the Fermi-Walker transportation law [20].
If spherical frame vectors (t, n,Ψ) and electric field vector E are respectively regarded as being a three-component real column vector and complex three-component column vector, then the Eq. (4) can be expressed in the form of Schrodinger's equation as follows [21] .
Apart from the evolution Eq. (5), there exists following restriction on E along with the ρ(ν).
This restriction, together with the Eq. (5), implies that the dynamical phase of the system vanishes. The vanishing of the dynamical phase is an attractive and special feature of the system, and it is caused by the transverse nature of electromagnetic waves, whose mathematical expression is given by the Eq. (6) on the unit 2-sphere whose stereographic projection is a 2-dimensional plane. This fact leads to conclude that the measured geometric phase automatically yields the total phase and vice versa. To compute the geometric phase, we firstly consider a finite segment of the space curve from a point ρ(ν0) to a point ρ(ν1). Then E(ν) is determined by the selection of E(ν0) for ν ∈ [ν0, ν1] and leads to an ultimate E(ν2). As a consequence, the geometric phase of the system is given by
where E(ν 0)* is the complex conjugate of the E(ν 0). To find the geometric phase of the system defined, in this case, we need to solve the Eq. (4). For this purpose, we choose the electric field vector E as the linear combination of the normal and binormal vectors of the spherical frame triad in the following way.
where EE* = 1 and polarization coefficients satisfy that |𝜙1|2 + |𝜙2|2 = 1. We obtain the following results for polarization coefficients.
If one considers Eqs. (7-9) then the geometric phase is computed by 𝜓 G = 0.
Case 2. In this case, the normalized complex electric field vector E is assumed to lie on a plane perpendicular to n. It is deduced from Maxwell's equations that the evolution of the electric field vector E concerning ν obeys the following formula of the normal Fermi-Walker transportation law [20]
To find the geometric phase of the system defined, in this case, we need to solve the Eq. (10). For this purpose, we choose the electric field vector E as the linear combination of the tangent and binormal vectors of the spherical triad in the following way.
where EE* = 1 and polarization coefficients |𝜚1|2 + |𝜚2|2 = 1. If one follows a similar approach as in the first case, then the following consequence is obtained for polarization coef ficients of the system.
If one considers Eqs. (7,10 - 12), then the geometric phase is computed by
4. Magnetic field vectors of the polarized light ray traveling along with a coiled optical fiber on the unit 2-sphere 𝕊2
In flat spacetime, the motion of a charged point particle was highly active and popular research field since the early study of Poincare, Abrahams, and Lorentz. As such, Einstein's study of special relativity was inspired on the electrodynamics of moving objects. Poincare and Lorentz were also motivated and guided by the equations of Maxwell to investigate spacetime transformations. This gave rise to the radical unification of Minkowski spacetime. In this context, it is fairly true that Maxwell equations played a key role to comprehend the profound connection between the dynamics of the major physical fields and interactions. Further researches, in turn, led to connect the spacetime geometry with the electromagnetic field. As a conclusion, it is observed that the field equations are entirely general at the very base of the electromagnetic theory, regardless of considering of any affine structure or metric of spacetime, however, its comprehension in spacetime by way of essential connections admits constitutive relations between the causal framework of spacetime and electrodynamics.
The study of magnetic fields and their associated magnetic curves is one of the research topic situated at the interaction between physics and differential geometry. A closed 2-form 𝒦 on the ordinary space (ℝ3, ·) is called a magnetic field. The Lorentz force on (ℝ3 , . ·, 𝒦) is defined by the skew-symmetric one-to-one tensor field Φ on ℝ3 fulfilling that
where 𝒵, S ∈ 𝒳 (ℝ3). Thanks to these fundamental facts, a trajectory produced by the magnetic field can be described as an arc-length parametrized space curve ξ on ℝ 3 if it satisfies following Lorentz equation
where ξ = ξ(ν). In the physical context, it is said that magnetic curve ξ is the trajectory of a point charged particle under the influence of K in the magnetic background (ℝ 3, ·, 𝒦).
In the case of the three-dimensional space, vector fields and 2-forms can be described via the volume form dv h , and the Hodge star operator ⋆ of the manifold. Hence, divergence-free vector fields and magnetic fields are in (1-1) correspondence. Therefore, for any vector field M on the three-dimensional space, the Lorentz equation can be given by the following formula
where div(M) = 0 [22].
In this context, we define magnetic curves generated by the electric field vector E, along with the polarized light ray coupling into a coiled optical fiber on the unit 2-sphere 𝕊2, by considering the Lorentz force. These curves are called spherical magnetic curves (SEM-magnetic curves) along with the paper since it is used the definition of both electric and magnetic field vectors during the generation process.
The direction of the state of the polarized light ray is referred by the direction of the electric field vector E in the coiled optical fiber. The SEM-magnetic curve of the Ψ = Ψ(ν) along with the polarization plane of a light ray propagating in the coiled optical fiber in the space is defined by
where M is any vector field in the space with div(M) = 0.
This definition is given naturally from Eqs. (13-15).
In the first special circumstances, we firstly suppose that E lies on a plane perpendicular to t along with θ : Ψ = Ψ(ν). Ev can be computed by using Eqs. (4-9) as follows.
Under the assumption of the Eq. (16) and following identities
one can compute the Lorentz force Φ in the spherical triad (t, n, Ψ) of the SEMt-magnetic curve of the Ψ in the following manner.
Now, one can characterize a special magnetic field vector that contains magnetic trajectories of the SEMt-magnetic curve of the Ψ. To do that, one should first consider an arbitrary divergence-free vector field M on the unit sphere 𝕊2. Then we can assume that M is written as a linear combination of the spherical triad vectors along with the SEMt-magnetic curve of the Ψ such that it does not vanish on any points of the curve. From the Eq. (16) it is known that ΦM=0. Thus it is readily obtained that
where π ί , 1 ≤ ί ≤ 3 are arbitrary sufficiently smooth functions. Finally, from Eqs. (18,19) one can conclude that SEMt-magnetic curve of the Ψ is a magnetic trajectory of a magnetic field vector M if and only if divergence-free vector field M is in the following form.
Secondly, we suppose that E lies on a plane perpendicular to n along with θ : Ψ = Ψ(ν). If one considers Eqs. (10-17) then the Lorentz force Φ in the spherical triad (t, n, Ψ) of the SEMn-magnetic curve of the Ψ is given by
In this case, one can also conclude that SEMn- magnetic curve of the ρ is a magnetic trajectory of a magnetic field vector M if and only if divergence-free vector field M is in the following form.
5. The evolution of electric and magnetic field vectors and its connection with NLS equations
The research of geometric evolution equations can be seen in various physical systems, such as the kinematics of a polymer chain, the motion of a vortex filament, low dimensional magnets, interface dynamics, magnetic spin chains, etc. Such interrelations between physical systems and geometric evolution have led to comprehend many significant consequences belonging to the underlying dynamics of the aforementioned mechanisms. Space curves have been heavily considered as the main tool to develop the theory of evolution equations. For example, Lamb [23] focused on a space curve, whose motion is represented by various linear equations. He showed that, under some circumstances, the evolution equations of moving curve are equivalent to the sine-Gordon equation, nonlinear Schrödinger (NLS) equation, and nonlinear heat system (NHS) equation. Murugesh et al. [24] and Wei-Zhong et al. [25] defined some other classes of evolution equations for moving curves and gave corresponding integrability and compatibility conditions to discover soliton equations. Ding and Inoguchi [26] obtained that unit speed non-lightlike space curves obeying a binormal motion satisfy Minkowski Heisenberg model for unit pseudo-sphere and unit hyperbolic 2-space embedded in Minkowski space. Then Gürbüz [27, 28] introduced three distinct families of evolution equations for non-lightlike curves according to the Darboux frame and Frenet-Serret frame in three-dimensional Minkowski space. However, it should be noted that all these research efforts have followed the remarkable paper conducted by Hasimoto [29]. In that paper, Hasimoto defined such a special transformation by combining the curvature and torsion functions of the thin vortex filament, that it can be reduced to NLS equations.
In this section, we demonstrate the relation between the evolution equation of the electric field (E) and magnetic field (M) vectors of the polarized light ray traveling in a coiled optical fiber, which is supposed to correspond a moving space curve lying on the unit sphere 𝕊2, and NLS equations. To be more specific, we firstly consider the basic identities governing the motion of the coiled optical fiber, and we find the evolution of the electric field (E) and magnetic field (M) vectors of the polarized light ray. Then, we introduce a specially defined Hasimoto function for each type of motion to relate the evolution equations of the electric field (E) and magnetic field (M) vectors into the special type of NLS equations
Theorem 5.1. Let 𝒪 be a coiled optical fiber that describes a sufficiently smooth space curve θ : Ψ = Ψ(ν, r) lying on the unit sphere 𝕊2 such that polarized light ray is coupled into the 𝒪. Let suppose that E lies on a plane perpendicular to t, along with θ : Ψ = Ψ(ν). If Ψ obeys the first kind of binormal motion (tr = t vv ) then the evolution equation of the electric field vector (E) of the polarized light ray traveling along with Ψ satisfies NLS equations and the magnetic field vector (M) satisfies following evolution equation.
where 𝒜 is a smooth function depending on both (r, v).
Proof. If E lies on a plane perpendicular to t then it is already known from Eqs. (8,9) that
On the unit sphere 𝕊2 , a spherical frame equation is introduced as an orthonormal triad (Ψ, n, t) of unit vectors to investigate the intrinsic features of a space curve Ψ, which is given by Eqs. (1,2). If Ψ is supposed to obey the first kind of binormal motion, then Ψ both depend on the arc-length and time parameters. The first kind of binormal motion of a space curve is given by
To define time-dependent spherical frame equations for a moving space curve Ψ = Ψ(ν, r), obeying the first kind of binormal motion, we find it convenient to improve the following method. Due to the orthonormality condition we have ( t · n) = 0 and (t · Ψ) = 0. If we differentiate both equalities concerning the time parameter then it is obtained that (t · n r ) = - (t r · n) and (t · Ψr) = - (t r · Ψ). If we also consider the fact that (n · n) = 1 and (Ψ · Ψ) = 1 and take the partial derivative of each equality we also obtain that (n · nr ) = (Ψ · Ψr,) =0. Under the light of this information, the rest of the time evolution equation of the spherical triad is computed by
where 𝒜 is an arbitrarily chosen sufficiently smooth function depending on both (r, v). This background is enough to proceed the next stage and find the time evolution equation of the electric field vector (E) of the polarized light ray traveling along with Ψ. To do that we should first consider the compatibility condition, which strictly asserts that E vr = E rv . Then we describe specially designated complex quantities, which carry a complete characterization of the moving curve. These quantities are called as the first kind of Hasimoto functions and they contain geodesic curvature term in the following way.
If one uses Eqs. (23-26) then a straightforward calculation yields that
where
Finally, if 𝒜 is assumed to have the form 𝒜 = - ίδ 0 δ 1 , then we obtain one of the AKNS- hierarchies equation as a special case of the Eq. (28) as follows.
When E is assumed to lie on a plane perpendicular to t along with Ψ, the magnetic field vector (M) is computed by using the Eq. (20) as M=n + μΨ. If Eqs. (24,26) are considered and the compatibility condition Mvr = Mrv is used then it is obtained that
Theorem 5.2. Let 𝒪 be a coiled optical fiber that describes a sufficiently smooth space curve θ : Ψ = Ψ(ν, r) lying on the unit sphere 𝕊2 such that polarized light ray is coupled into the 𝒪. Let suppose that E lies on a plane perpendicular to n along with θ : Ψ = Ψ(ν). If Ψ obeys the second kind of modified binormal motion (nr = n vv ) then the evolution equation of the electric field vector (E) of the polarized light ray traveling along with Ψ satisfies an NLS-type equation and the magnetic field vector (M) satisfies following evolution equation.
where C is a smooth function depending on both (r, v).
Proof. If E lies on a plane perpendicular to n then it is already known from Eqs. (11,12) that
To define time-dependent spherical equations for a moving space curve Ψ = Ψ(ν,r), obeying the second kind of modified binormal motion, we assume that nr = nvv. Then this motion of a space curve implies that
Due to the orthonormality condition we have
Thus the time evolution equation ofthe spherical triad is computed by using Eqs. (32,33) as follows.
where C is an arbitrarily chosen sufficiently smooth function depending on both (r, v). Then we can define the second kind of modified Hasimoto functions as follows.
If one uses Eqs. (31 - 35) then a straightforward calculation yields that
where
When we suppose that E lies on a plane perpendicular to n along with Ψ, the magnetic field vector (M) is computed by the Eq. (25) as M =μΨ. If Eqs. (32-34) are considered and the compatibility condition Mvr = Mrv is used then it is obtained that
6. Optical Soliton Perturbation in Coiled Optical Fiber with Evolution Equations of Electric and Magnetic Field Vectors by Traveling Wave Hypothesis Approach
In this section, we propose perturbed solutions of the nonlinear Schrodinger's evolution equation governing the propagation of solitons through the electric field (E) and magnetic field (M) vectors of the polarized light ray traveling in a coiled optical fiber. The traveling hypothesis approach is employed to compute analytical soliton solutions. The numerical simulations are also provided to supplement the analytical outcomes. Here we consider evolution equations of the electric field and magnetic field vectors given in Theorem 5.2 since it is a much rare case. We attempt to discover the dynamics of optical soliton propagation of E and M along with the coiled optical fiber.
Let suppose that E lies on a plane perpendicular to n along with the coiled optical fiber. Then the evolution equation of the electric field of the polarized light ray traveling along with coiled optical fiber satisfies following NLS-type equation given by the Eqs. (37,38).
We implement the traveling wave transformation method presented by Biswas [30] for the Eq. (38) in the following way.
where Q describes the speed of the wave.
By placing the Eq. (40) into the Eq. (37), it is obtained that
If one solves the Eq. (41) it is further computed that
If we reconsider the Eq. (37) then we get by the traveling wave transformation method that
where κ, s and Q respectively, describe the frequency, wave number and the speed of the wave. Thus real and imaginary sections can be deduced by the following approach. The real sections are as follows.
Solving the Eq. (44), we obtain that
The imaginary sections are as follows.
Solving the Eq. (47), we obtain that
Then it is finally obtained that
In Figs. (1,2) , it is represented 3D simulations of the soliton propagation of the electric and magnetic field vectors of the polarized light ray along with the coiled optical fiber on the unit sphere for the solution of the real and imaginary section respectively.
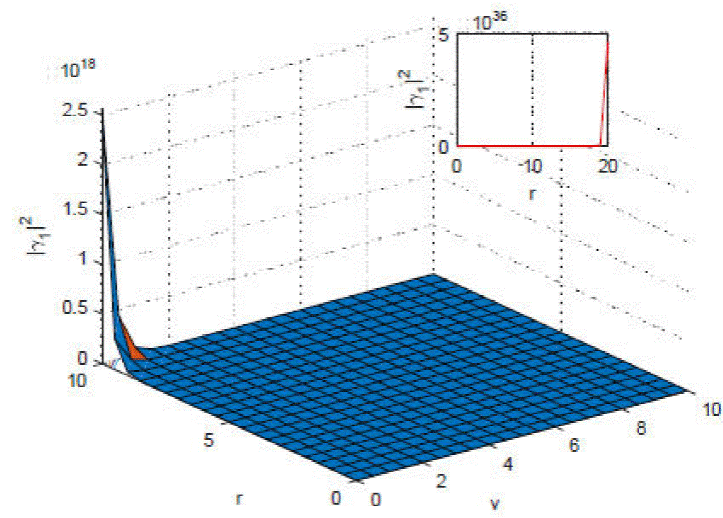
Figure 1 Analytical solution (46) of the Eq. (39) is given for κ = -2, s = 1, Q = 1.2, c 3 = c 4 = 1.
7. Conclusion
In this study, we derive the evolution equation of the electric field and magnetic field vectors of the polarized light ray, and we use such analogy to obtain optical soliton solutions in coiled optical fiber on the unit 2-sphere 𝕊2. As is known the stereographic projection maps a unit 2-sphere 𝕊2 onto a plane ℝ2 . Then a natural question arises whether the soliton solutions on the unit 2-sphere 𝕊2 remains similar in the plane or not. The answer is negative since the Riemannian curvature of the unit 2-sphere is nonzero as opposed to the Rieman-nian curvature of the 2-dimensional plane ℝ2. By using this knowledge we will also look for the solutions of the evolution equations of the electric field and magnetic field vectors of the polarized light ray in the different spacetimes with different dimensions whose Riemannian curvature is not the same as 𝕊2 such as De-Sitter spacetime, anti De-Sitter spacetime, 2-dimensional plane ℝ2 .











 nueva página del texto (beta)
nueva página del texto (beta)



