1. Introduction
The classical Lienard equation [1]
and the equivalent system
with F (x) =
In [11] the system (1) was studied in an attempt to unify, in a general result, the methods known for particular cases on an important qualitative aspect: the boundedness of solutions. The Lienard system (1) is often taken as the typical example of a nonlinear self-excited vibration problem and, since its appearance (cf. [12]) it has become a paradigm of nonlinear analysis. It is clear then that the study of (1) is of paramount importance in physics and other sciences.
However, the case of Lienard-type systems having non integer derivatives has received scarce attention, although the nature of many processes and phenomena makes them suitable for modeling with fractional differential equations. Over the years many mathematicians have built up a large body of mathematical knowledge on fractional calculus, but despite fractional calculus is a natural generalization of classical calculus, and although its mathematical history is equally long, it has, until recently, paid a negligible role in applications. One reason for this could be that until recently, until a few years ago, the basic facts about fractional calculus were not readily accessible even in the mathematical literature (see [13, 14], for example).
On the other hand, if we take into account the existence of different fractional derivatives, both global (Gronwald-Letnikov, etc.) and local (conformable and non-conformable), versus classical derivatives, one may ask: How significant is the use of fractional models in the study of processes and phenomenon? How advantageous is the use of these non-integer order derivatives? Is any information lost when using fractional derivatives instead of classical derivatives? In fact, fractional calculus is now applied successfully to address several problems of physics, see some examples in Refs. [15-19].
Therefore, the purpose of this paper is to investigate the qualitative behavior of systems of type (1) under non-usual assumptions; that is, the functions evolved in (1) are the same but the derivatives considered are of different types (i.e., integer order and fractional order such as global, Gronwald-Letnikov and local, conformable and non-conformable), and elucidate the questions posed above. To that end we apply fractional derivatives to six known examples of Lienard-type systems, each one showing relevant dynamical properties: In example I the origin (0,0) is an asymptotically stable equilibrium position, in example II the system has a stable limit cycle, system III has no continuable solutions, example IV has unbounded solutions, example V requires additional conditions for the existence of a stable limit cycle, as compared to example II, and example VI is a particular case of a linear system with constant coefficients. Thus, starting with numerical experiments we conclude with some theoretical-methodological remarks.
2. Fractional approach to Liénard-type systems
Fractional calculus is a generalization of integration and differentiation to non-integer-order fundamental operations
The three most frequently used definitions for the general fractional differintegral are the Griinwald-Letnikov (GL) definition, the Riemann-Liouville, and the Caputo definition (see [14]). Other definitions are associated with well-known mathematicians, for instance, Weyl, Fourier, Cauchy, Abel, etc.
2.1. Definitions and properties of fractional derivatives
The Grünwald-Letnikov definition is given as (with a = 0)
with
or the analogous representation
where [·] denotes the integer part. In the case of discrete times
and
we have
To overcome some difficulties of the classical fractional derivative, Khalil et al. [20] came up with an interesting idea that generalizes the familiar limit definition of the derivative that allows to successfully introduce a conformable fractional derivative (see Definition 2..2 below). Thus, below, a definition of a conformable generalized fractional derivative of a function at a point t is introduced and some useful results are obtained.
Definition 2..1 Let I be an interval
Classical calculus assures that if f is a function defined in a neighborhood of the point t and there exists D n f (t), then
Therefore, if α = n ∈ ℕ and f is smooth enough, Definition 2..1 coincides with the classical definition for the n-th derivative.
The following conformable fractional derivative is introduced in [20].
Definition 2..2 Given
The latter conformable fractional derivative is a particular case of Definition 2..1 for α ∈ (0,1]. An important difference between Definition 2..1 and the conformable derivative T a is that our definition permits direct inferences for the fractional derivative of any positive order, whereas in Definition 2..2 the fractional derivative can be applied only to the last derivative.
Theorem 2..1 Let I be an interval
Proof 2..1 If we take
and there exists G α f (t) if and only if there exists f'(t), since t > 0.
The following result contains some basic properties ofthe derivative G α (see [21,22]).
Proposition 2..2 Let I be an interval
(1) af + bg is G α -differentiable at t for everya,a,b ∈ ℝ and G α (af+bg) (t) = a G α (f)(t) + b G α (g)(t).
(2) If α ∈ (0,1], then f g is G α -differentiable at t and G α (fg)(t) = f (t) G α g(t) + g(t) G α f (t).
(3) If α ∈ (0,1], then f g(t) ≠0, then f/g is G α -differentiable at t and G α (f/g)(t) = [g (t) G α f(t) - f(t) G α g(t)]/g(t)2 .
(4) G α (λ) =0, λ ∈ ℝ.
A similar argument to the one in the proof of Proposition 2.. 2 (see [22]) provides the chain rule for this fractional derivative for α ∈ (0, 1]. Let g be a G α -differentiable function at t > 0 and f a differentiable function at g(t). Then,
Some theorems of the classical calculus have their equivalents in the fractional calculus.
Theorem 2..3 Let a ≥ 0 and f : [a,b] → ℝ a continuous function such that f (a) = f (b) and f is G α -differentiable on (a, b) for some α ∈ (0, 1]. Then there exists c ∈ (a, b) such that G α f(c) = 0.
Úsing the previously developed ideas we can demonstrate the following classical result.
Proposition 2..4 Let a ≥ 0 and f, g : [a,b] → ℝ continuous functions which are G α -differentiable on (a, b) for some α ∈ (0,1]. If G α f (t) = G α g(t) for all t ∈ (a, b), then there exists a constant C such that f(t) = g(t) + C forevery t ∈ [a, b].
Although the index law for iterated derivatives is not fulfilled, the following result provides a general rul to compute iterated derivatives.
Let I be an interval
The integral operator J" is defined for every locally integrable function f on I as
The following results contain some basic properties of this integral operator (see [21, 22]).
Proposition 2..5 Let I be an interval
Proposition 2..6 Let I be an interval
for everycontinuous function f on I and a, t ∈ I.
For additional details on fractional calculus we refer to [23-25].
The nonconformable derivative (see [26]) is defined as:
Definition 2..3 Given a function f : (0,∞) → ℝ and α ∈ (0,1], the
If f is
So for discrete times we have
Also, note that
2.2. Some Lienard-type systems
For the numerical experiments we present below we use the standard Euler method that for the classical Lienard system of Eq. (1)
provides the discrete orbits
Moreover, we consider here the general system
where x (α) and y (ß) are fractional operators and 0 < α, β ≤ 1 . Therefore, in the conformable case [from (8) and Theorem 2..1] we have
with solutions
while in the non-conformable case [from (7) and (8)]
the solutions are written as
Note that the standard Euler method has a computational cost of n and an error of O(h 2). But for the Grünwald-Letnikov case, we use an iterative Euler method with computational cost of n 2 and error of O(h): from (3) and (8) we have
with solutions
Below we use a time step of size h = 10-6. We verified that we obtain qualitatively the same solutions when using smaller values of h.
Now we consider six relevant cases of Lienard-type systems subject to different conditions and within the framework of different notions of derivatives (integer order and fractional order, global and local). For them, we will take as a base some works that have presented diverse qualitative results on these systems, under the general following conditions:
Case I. The classical Lienard system. Consider the system (1) under assumptions (a) and (c). In this case, the equilibrium point (0,0) is asymptotically stable (see for example [27] and [28] for additional results). Here we consider as an example the system
In Fig. 1 we present numerical solutions of the Lienard system of Eqs. (9) for (a) classical, (b) Grünwald-Letnikov, (c) conformable, and (d) non-conformable derivatives. The classical derivative solution is also included in panels (b-d) as a reference (black thick curve). Red thin curves in panels (b-d) are solutions for the orders α = 0.975, 0.925, and 0.9 such that their proximity to the black curve is greater the closer to 1 is the order of its derivative; as expected. The blue dot indicates the initial condition. The rest of the figures, Figs. 2 to 6 corresponding to Cases II to VI, respectively, display the same structure as Fig. 1 for comparison purposes.
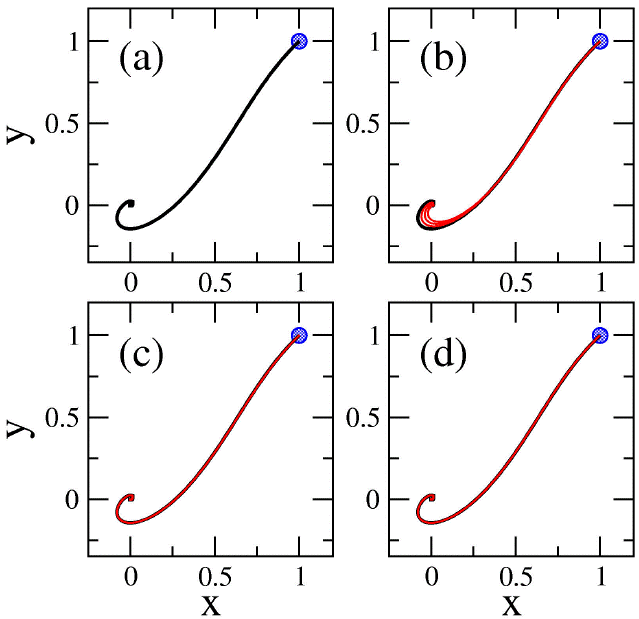
Figúre 1 Numerical solutions of the Lienard system of Eqs. (9) for (a) classical, (b) Grünwald-Letnikov, (c) conformable, and (d) non-conformable derivatives. Red thin curves in panels (b-d) are solutions for the orders α = 0.975, 0.925, and 0.9. The classical derivative solution is also included in panels (b-d) as a reference (black thick curve). The blue dot indicates the initial condition.
Notice that if we consider the system
instead of (9), we get x'' - (x 2 + 2)x' + 2x 3 = 0, which does not satisfies either (a) or (c) and has the unbound solution x = e 2t .
Case II. The study of the existence of limit cycles is one of the fundamental topics of the Qualitative Theory since Poincaré treated it at the end of the XIX century. In [29] the author studies the existence of limit cycles and its algebraic character, being a fundamental example the system
The numerical solutions of this system are shown in Fig. 2.
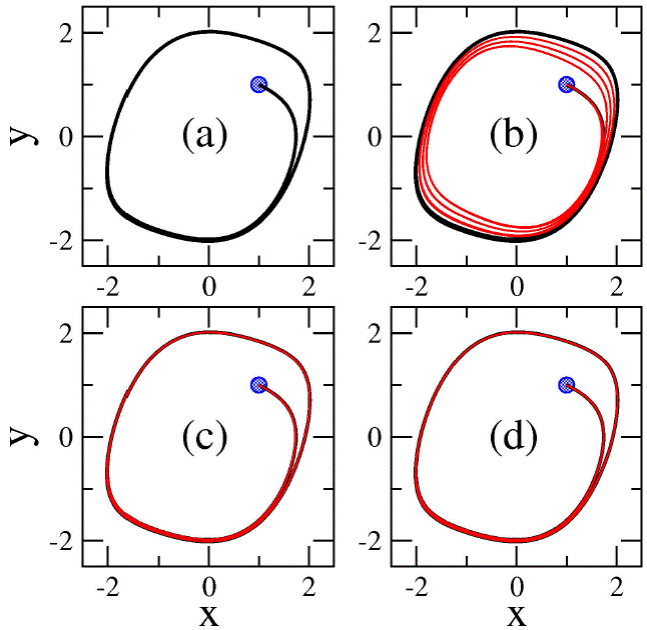
Figúre 2 Numerical solutions of the Lienard system of Eqs. (11) for (a) classical, (b) Grünwald-Letnikov, (c) conformable, and (d) non-conformable derivatives. Red thin curves in panels (b-d) are solutions for the orders α = 0.975, 0.925, and 0.9. The classical derivative solution is also included in panels (b-d) as a reference (black thick curve). The blue dot indicates the initial condition.
Case III. Hricisakova in [30] studied the system (see also [31]):
This system under the assumptions xf( x) > 0, xg( x) > 0 for all x ≠ 0, and f and g continuous on ℝ, may have a negative solution which is not continuable to the right as the following example shows: x '' + 3xx ' + x 3 = 0, has the solution x(t) = t - 1 . In general x(t) = (x α)-1 with t < α is a noncontinuable solution of the above equation and x(t) = (x α)-1 , t > α, is also a noncontinuable solution of this equation.
The numerical solutions of system (12) are shown in Fig. 3.
Figúre 3 Numerical solutions of the Lienard system of Eqs. (12) for (a) classical, (b) Grünwald-Letnikov, (c) conformable, and (d) non-conformable derivatives. Red thin curves in panels (b-d) are solutions for the orders α = 0. 975, 0.925, and 0.9. The classical derivative solution is also included in panels (b-d) as a reference (black thick curve). The blue dot indicates the initial condition.
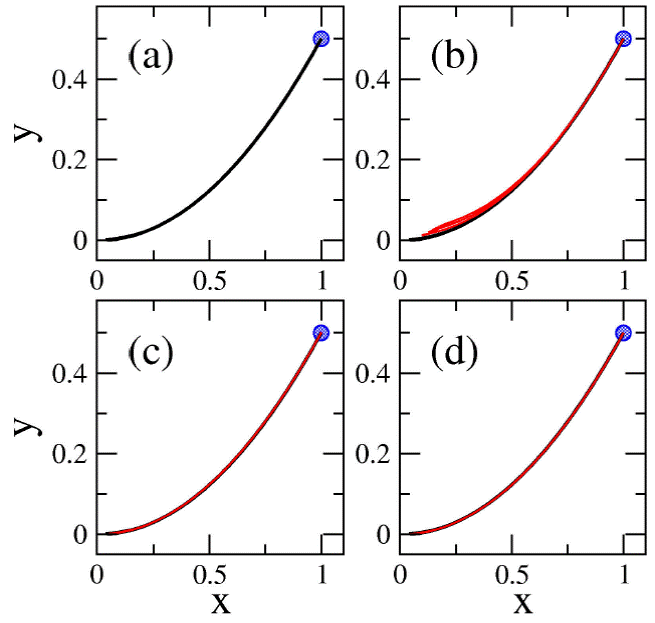
Case IV. Yu and Zhang in [32] studied the system (example 1, page 54):
which satisfies condition (c) and has the solution y =
Then, in Fig. 4 we present numerical solutions of (13).
Figúre 4 Numerical solutions of the Lienard system of Eqs. (13) for (a) classical, (b) Grünwald-Letnikov, (c) conformable, and (d) non-conformable derivatives. Red thin curves in panels (b-d) are solutions for the orders α = 0.975, 0.925, and 0.9. The classical derivative solution is also included in panels (b-d) as a reference (black thick curve). The blue dot indicates the initial condition.
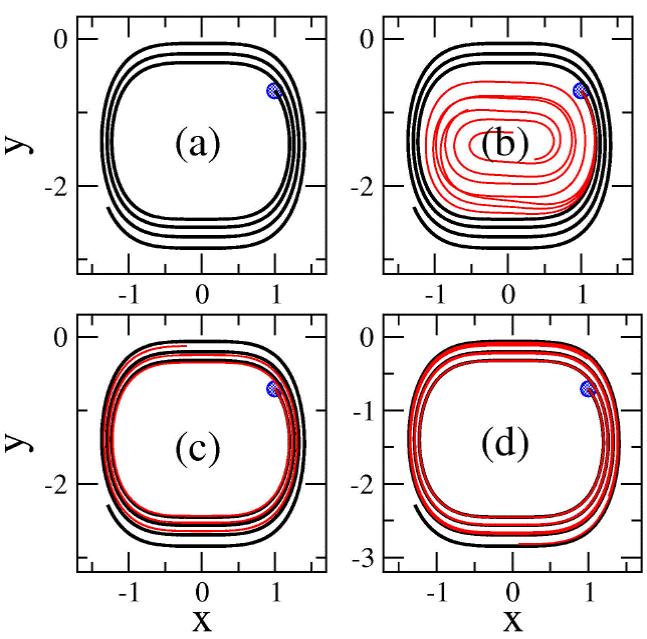
Case V. Kooij and Jianhua considered in [12] the system:
which has a stable limit cycle at the origin (see example 4.1, page 272 of [33]). They showed that if the condition on the monotonicity of F(x) is violated, then an additional condition to guarantee the uniqueness of the limit cycle is needed.
The numerical solutions of (14) are presented in Fig. 5.

Figúre 5 Numerical solutions of the Lienard system of Eqs. (14) for (a) classical, (b) Grünwald-Letnikov, (c) conformable, and (d) non-conformable derivatives. Red thin curves in panels (b-d) are solutions for the orders α = 0.975, 0.925, and 0.9. The classical derivative solution is also included in panels (b-d) as a reference (black thick curve). The blue dot indicates the initial condition.
Case VI. The linear system
can be considered as a limiting case for the Lienard system of Eq. (1). Its roots (-1 ±
Finally, Fig. 6 shows numerical solutions of system (15).

Figúre 6 Numerical solutions of the Lienard system of Eqs. (15) for (a) classical, (b) Grünwald-Letnikov, (c) conformable, and (d) non-conformable derivatives. Red thin curves in panels (b-d) are solutions for the orders α = 0. 975, 0.925, and 0.9. The classical derivative solution is also included in panels (b-d) as a reference (black thick curve). The blue dot indicates the initial condition.
3. Theoretical-methodological remarks
In addition to the standard Lienard system of Eq. (1) we consider here the more general system of Eq. (8):
where the notation x (α) and y (β) labels a fractional operator; for example the Grünwald-Letnikov fractional derivative (with 0 < α, β ≤ 1). From (8) we have,
Consider the orbit T(x,y) of (1) and the curve C(x,y) which is the image of T by the application of Φ. We can show that C is an orbit of (8) as follows.
In the region x > 0, if a point (x, y) belongs to the curve C we have from (8) that
Thus the curve C in the region x > 0 is an orbit of (8) obtained by multiplying the orbit of (1) by a "factor" which contains a fractional integral of complementary order to the derivative considered; i.e., the Jacobian of the isomorphism. If the orbit T crosses the y-axis in some point (0, y 1) then the orbit C crosses the y-axis in (0, Ф(0,у 1)), with y 1 > 0 or y 1 < 0.
Let C ' (x, y) be an orbit of (8) passing through the point (0, Ф(0,у 1)) and T'(x, y) be the inverse image of C' by the application of Φ. From unicity of solutions of (1), T and T' coincide and C and C ' therefore also coincide by injectivity of Φ (the Jacobian y (𝛽-1) /x (α-1) ≠ 0). Thus Φ maps orbits of (1) into those of (8).
A similar result is obtained in the region x < 0. Therefore notice that if xF(x) > 0 and xg (x) > 0, for x ≠ 0, then there exists an isomorphism Φ of the phase plane of the system (1) on the phase plane of the system (8), which is a one to one correspondence between all orbits of (1) and those of (8).
4. Conclusions
Identifying the parameters that lead to stable or unstable behaviors in dynamical systems is of paramount importance; since stability represents the ability to return to the state of equilibrium after changes or temporary perturbations, according to external and internal factors. Also, the study of the behavior of orbits around critical points for the identification of possible stationary states in dynamical systems is of main relevance: When a small perturbation is made to a given system, and there is a departure from the critical point, if the system is in a state of stable equilibrium it will approach again the critical point; on the contrary, it is in a state of unstable equilibrium.
Here, we made a stability analysis of Lienard-type systems where the perturbation is the application of non-integer differential operators. It is clear that the Jacobian of the fractional differential operator can "distort" the geometric behavior of the orbits around the equilibrium points, thus we identified in which cases this distortion influences importantly the dynamics of the system (as compared to the dynamics under the integer differential operator).
It is well known that obtaining analytical solutions for given dynamical systems, mainly in the nonlinear case, is practically impossible. Thus, a numerical approach together with a qualitative analysis becomes a suitable strategy; hence, the relevance of the methodology used in this work. Here we have studied the behavior of Lienard-type systems using different derivative operators: integer and fractional. The fractional derivatives we used were Gränwald-Letnikov (GL), conformable (G), and non-conformable (N), while the ordinary derivative was also shown as a reference. The main disadvantage of GL derivative is the non-compliance of several classical properties of the ordinary derivative, which makes it unable to "replicate" the case studies of the integer-order. The local fractional G and N derivatives, do not have that disadvantage thus have some points in favor. For example, the G derivative is conformable which ensures an "equivalence" with the results of the integer-order at all finite times. In the case of the N derivative, although it is non-conformable, at infinity behaves like the ordinary derivative, which is of paramount importance in the Qualitative Theory. Moreover, we have demonstrated theoretically that there is a "certain geometrical similarity" between the trajectories of Lienardtype systems with different differential operators; also, with numerical examples we showed that some cases suffer more distortions than others.
Moreover, from the figures presented in Sec. 2.2, we can make the following methodological observations:
(1) If the equilibrium position is globally asymptotically stable (or completely unstable), the orbits of the system "resist" the deformations and still offer a good approximation when α and β are close to 1.
(2) If the equilibrium position is not structurally "good", the distortion manifests itself easily; i.e., the factor y (1-ß) /x (1-α) strongly influences the configuration of the orbits.
Finally, can we contribute to answer the questions posed at the beginning of the paper? Well, we realized that the study of the equilibrium points of the ordinary system provides hints of the behavior of the system under fractional differential operators; i.e., the study of a fractional model should be accompanied by the qualitative study of the corresponding ordinary system. Indeed the previous analysis of the corresponding ordinary system may considerably help to understand and improve processes or phenomena studied in the frame of fractional derivatives.











 nueva página del texto (beta)
nueva página del texto (beta)


