1. Introduction

Multiple scattering is generally not considered in X-ray or neutron diffraction from polycrystals, due to the negligibly low intensity expected from this effect; however, measurements of pole figures show significant differences in many cases when density maxima from first and second orders are compared to each other, indicating that primary or secondary extinction could be present^1-3. If secondary extinction is dominant, a way to verify it could be to test if the curve of crystallite orientations for the second diffraction coincides with high populated zones of the Orientation Distribution Function (ODF) of the textured sample. As well known of diffraction from polycrystals, the intensity at a point of a Debye-Scherrer ring hkl, or the pole density at a point on the pole figure, is caused by the superposition of the intensities diffracted by many crystallites, all of which satisfy the Bragg condition for the planes (hkl). This crystallites have in common the normal direction of planes (hkl), but differ otherwise in orientation so that their orientations lie along a curve in the Eulerian space, i.e. the pole figure is a projection of pole densities along a path through the ODF corresponding to a 2 π-rotation around the diffraction vector ⁵. This happens also for the second diffraction process, being the incident vector orientation the only difference, so that the diffracting crystallites lie also along a curve in the Eulerian space. So the aim of this work is to evaluate the orientation curves for the main reflections for a secondary diffraction, and for a general sample orientation given by the conventional angles (χ,φ). This scope was proposed by Palacios et al., ¹, however, the mathematical method used allowed only one solution of the main equation expressing the Laue condition for a second reflection (Eq. (7) of ¹), and no modifications were made for especial cases where no solution (for example reflections where h=k=0) could be obtained. This work aims to solve this deficiencies, and to improve solutions. For the sake of completeness, the figures and the background of the main equation are repeated here.

2. The method

Let k0 be the wave vector of an X-ray photon, which has already been diffracted by planes (h0k0l0). If θ0 is the Bragg angle of the first diffraction, and k=2π/λ with λ the radiation wave length, this is in the sample reference system

To determine possible secondary diffraction processes this wave can produce in the polycrystalline sample, the orientation curve of crystallites contributing to the intensity registered at a general point of a Debye-Scherrer ring produced by diffraction from plane (hkl) is to be calculated. Figure 1 shows the primary diffraction layout, and Fig. 2 shows the sequence of both diffraction processes.

FIGURE 1 Reference frame for the sample at the orientation x = 0 and φ = 0. 

FIGURE 2 Sequence of primary and secondary reflections by crystallites 1 and 2 respectively. D indicates the detector direction, and D - S the direction of the point of afrom which Debye-Scherrer ring which this particular event affects. 

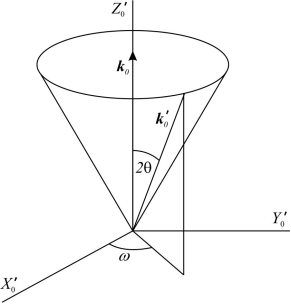
It is convenient to rotate the reference system an angle α around X0, to make k0 to coincide with the Z axis. Let ω be an angle characterizing a point of a Debye-Scherrer ring, as shown in Fig. 3. The wave vector of the second diffracted wave is then in the new reference system

FIGURE 3 Debye-Scherrer ring for a general secondary reflection. 

And expressed in the sample reference system, it is

If Q0≡k'0-k0, then

with

where the superindex 0 indicates that the sample is in its initial orientation (0,0).

Q₀ in terms of the pole figure angles (χ,φ)

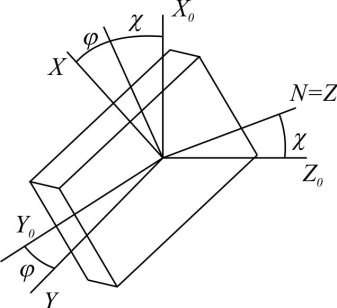
As in a measure of a pole figure, let the sample be rotated an angle χ around the Y axis (TD), followed by a rotation of an angle φ around the normal direction of the sample. Figure 4 shows the sample in this general orientation for the measurement of a point (χ,φ) of the pole figure. Q is the vector Q0 as seen by the sample in its new orientation after both rotations:

FIGURE 4 Sample in a general orientation (χ,φ). Axis X ₀ , Y₀ and Z₀ are those of the sample in its initial symetric orientation. X, Y y Z are the axis after rotation. N is the sample normal. 

Crystallites for which Q satisfies Laue conditions

Let hkl be a particular reflection, and C a crystallite such that the vector Q satisfies Laue conditions for a certain reciprocal vector Ghkl. The vector Q as seen by C is

where φ1, ϕ, φ2 are the Eulerian angles of the crystallite; i.e. those which bring the sample system to coincidence with C, and Mφ1ϕφ2 is the rotation matrix ⁶ (Eq. (2.50)):

Then

where Ghkl=hA+kB+lC is the reciprocal lattice vector corresponding to the reflection hkl. For a cubic system with lattice constant a:

where í, j and k^ are unit vectors of C.

After applying matrix Mφ1ϕφ2 to (6), with k2π/λ, and from (8) and (9), it follows

where

Total of solutions

Except for the especial case h=k=0, following solutions gi,j are obtained:

These are two curves if h2+k2>0, or a straight line along φ2 if h=k=0.

3. The computer program

A program in Dev C ++ has been written, as follows:

Primary data are given: Pole figure affected, i.e. (h0k0l0), secondary reflection (hkl), point ω of the Debye-Scherrer ring of secondary reflection, lattice constant of sample a, sample orientation (χ,φ), and wavelength λ. From these data, Bragg angles θ0 and θ for primary and secondary reflections respectively are calculated. Initial φ1 and an increment Δφ1 are also given.

The especial cases h=k=0 is solved from Eqs. (18).

4. Results

Figures 5 and 6 show some results for a silver sample using copper radiation. Curves are usually closed but they appear fragmentary when some angle reaches the 2π value. Particularly not every value of φ1 has a solution. Two curves result for each sample orientation, and for first and second reflections since every orientation of the sample is represented by two sets of Eulerian angles (φ,φ,φ) (⁶, Eq. (2.4)).

FIGURE 5 Curves obtained for a general orientation of the sample: X = 35^° and φ = 45° planes 111 for both reflections, primary and secondary. Copper radiation was used, and the sample is silver. 

FIGURE 6 Curves obtained for the symmetrical orientation of the sample: X = 0° and φ=0°, and planes 111 as the plane causing the first reflection, and 202 as the plane causing the second reflection. Copper radiation was used, and the sample is silver. 

5. Conclusions

A method has been developed to determine the orientation curves of crystallites able to produce a second diffraction of an incident photon. Calculations require only modest computer capabilities, and they could be applied in future work to determine the integrated intensity of the whole Debye-Scherrer rings, as a measure to evaluate the intensity loss due to a possible effect of secondary extinction.

The aim of this work is thus fulfilled; however, to know if these curves pass through high pole density zones, further calculations will be necessary since on the one hand, due to symmetric properties of the samples, only a part of this cube of side length 2𝜋 will be sufficient, and on the other hand, every curve should be first subdivided in segments of equal length. Both aspects require careful operations, and for the moment they are beyond the scope of this work.