1. Introduction
Fractional calculus (FC) is the natural generalization of the ordinary calculus involving derivatives and integrals of noninteger order. During the thirties or so, FC has attracted much attention due to its powerful and widely used tool for better modelling and control of processes in many areas of science and engineering 1-3. Nowadays, there are several definitions of fractional derivatives and integrals 4. These definitions include Riemann-Liouville, Grunwald-Letnikov, Caputo, Weyl 5-6 and, more recently, Caputo-Fabrizio 7 and Atangana-Baleanu 8. The most used definitions are the Riemann-Liouville and the Caputo fractional derivatives. There are classical applications where FC has shown its great capabilities, such as: the tautochrone problem 9, models based on memory mechanism 10, fractional diffusion equation 11, new linear capacitor theory 12, the non-local description of quantum dynamics like Brownian motion and anomalous diffusion 13, to name a few.
All definitions of fractional derivatives satisfy the property of linearity. However, properties, such as the product rule, quotient rule, chain rule, Rolle’s theorem, mean value theorem and composition rule and so on, they are lacking in almost all fractional derivatives. To avoid these difficulties, in 14 it was proposed an interesting idea that extends the ordinary limit definitions of the derivatives of a function, called conformable fractional derivative. This definition allows for many extensions of some classical theorems in calculus, for which the applications are essential in the fractional differential models that existing definitions do not permit. It has attracted the interest of researchers, as it seems to satisfy all the requirements of the standard derivative. Also, the computing using this new derivative is much easier than using other definitions of fractional derivative. Therefore, there is a large number of works carried out using this new definition and its generalization, 15-24.
Motivated by this new conformable derivative, we apply it to obtain new class of smooth solutions for the Newton’s law of cooling. In addition, the convection coefficient of fractional order k(γ) is found.
2. Basic results on fractional conformable derivative
In the paper 14, a new definition of fractional derivative is given, it is called conformable fractional derivative, defined as: Let f: [0,∞) → ℜ a given function, then, the conformable fractional derivative of the order γ is defined by
for all t > 0 and 0< γ ≤1. This expression is a possible generalization of the standard definition of derivative. When γ = 1 from (1), we obtain
Although the fractional conformable derivative is easily computed, it is not conformable at γ = 0, namely lim γ→0 Tγ ≠ f. If f is γ−differentiable in some (0,a), a > 0, and limt→0┼ fγ (t) exists, then, fγ (0) = limt→0┼ f γ (t) holds. The most important properties of this conformable fractional derivative are given as theorem in 14.
Theorem: Let γ ∈ (0, 1] and f and g be γ-differentiable at a point t > 0, then
3. Newton law of cooling
Temperature difference in any situation results from energy flow into a system or energy flow from a system to surroundings. The former leads to heating, whereas the latter leads to cooling.
Newton’s law of cooling states that the rate of change of temperature of the body is proportional to the difference between the temperature of the body and that of the surrounding medium 25,
where T 0 is the initial temperature of the body at t = 0, Tm is the temperature of the medium, which is considered to be constant, and k is the cooling coefficient (or convective) defined as
where α is the heat transfer coefficient for convection, A is the heat transfer surface area, m is the mass of the body, C is the specific heat. The coefficient k is measured in inverse unity of time, s −1. Equation (2) predicts that the difference between the initial temperature T0 and surrounding medium temperature Tm drops exponentially
Many experiments seem to support the applicability of this simplified theory for temperature difference 25. From this equation we have that if t→0, then T →T0, and if t→∞, then T → Tm, the body and the surrounding are in the thermal equilibrium.
Now, suppose that after some given time τ, the temperature changes from T to T1, with these conditions we can find the value of k from (4),
This result is well known and can be found in any physical textbook.
The question here is; what happens in the case of fractional conformable calculus. Which of the two models gives the best result for the convective coefficient k and therefore, for the behaviour of the Newton’s law of cooling? The answer is the main result of this short communication which is given in the next section.
4. Newton fractional conformable cooling law
Usually, authors replace integer derivative operators with fractional ones on a purely mathematical basis. However, from the physical and engineering point of view, this is not completely correct, and some dimensional corrections in the new equation are required. Having this in mind, in 26 we proposed a systematic way to construct fractional differential equations using the fractional Caputo derivative, which consists of the following:
where γ is an arbitrary parameter, which represents the order of the derivative, 0 < γ ≤ 1, σt is a parameter representing the fractional time components in the system, its dimensionality is of time s26. It is interesting to note, that depending on the system the σt may be done in terms of the physical parameters of the system, for example, in our case it is convenient to take σt = 1/k, because [k] = [s−1]. Then, in our particular case, to obtain a fractional derivative we must replace the ordinary derivative by the fractional one as follows:
So, substituting this expression in the ordinary differential equation (2), we have the corresponding fractional differential equation of order γ,
This equation has been solved in the case of the fractional derivative of Caputo, having as a solution the Mittag-Leffler function 27-28. However, using the Caputo procedure it is not easy to calculate the convective coefficient k. Due to this, in this work we apply the recently introduced fractional conformable derivative 14.
For this, we take into account the expression (7) and the formula 6 of the above theorem, when n = 0, we have
Recalling that [k] = s−1 and [t] = s, then k1−γt 1−γ is dimensionless. Substituting this time fractional conformable transform in (8), we obtain an ordinary differential equation
This equation has the particular solution
Observe that in the case γ = 1, the Eq. (11) transforms in (4). Suppose now, that after a time τ we have a temperature T1, then, from (11) we have
From here we can calculate the fractional convective coefficient easily,
Figure 1 shows how k(γ) depends on the values of γ.

FIGURE 1 Shows the behaviour of cooling coefficient k(γ) for different values of γ, where T0 = 100°C, T1 = 60°C, Tm = 5°C and τ = 600s.
Figure 2 shows that as γ decreases the steady state solution is reached at longer times. Besides, in the case of fractional conformable differential equations (10) we have a stretched exponential functions as solutions, unlike the Mittag-Leffler solutions obtained using the Caputo derivatives. On the other hand, the Caputo fractional derivatives are non-local, whereas the fractional conformable derivatives are local. However, the behaviour of the system, in general, is similar 27-28.
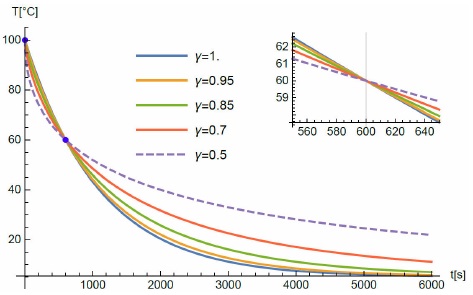
FIGURE 2 Shows the behaviour of the fractional Newton’s law of cooling (11) for different values of γ.
5. Conclusions
In this short communication we started with the ordinary differential equation for the Newton’s law of cooling, then, we used the method given in 26 to obtain the corresponding fractional differential equation. After that, we applied the conformable fractional derivative to obtain a first-ordinary homogeneous differential equation with non-integer power variable coefficients. When solving this equation we obtain a new class of smooth solutions for the Newton’s law of cooling given by stretched exponential functions. In addition, the convection coefficient of fractional order k(γ) is found easily. This conformable fractional derivative definition is a convenient definition in the exact solution procedure of fractional differential equations. Conformable fractional derivatives are easier to use when compared to the other fractional derivatives, as its derivative definition does not include any integral term.
Newton’s law of cooling is invoked in a wide range of contexts in applied science, for example, in materials science, high temperature superconductivity and atmospheric physics 29-30. We hope that the way of analysing the fractional differential equations using the conformable fractional transform (9) will be of great help in solving fractional equations that represent more complex systems. Of course, it will be interesting to compare the theoretical results with some experimental data.











 nova página do texto(beta)
nova página do texto(beta)


