1. Introduction
The idea of the spatial confinement of quantum systems has gained growing interest in recent years due to its potential ability to model a great number of applications in different areas of Physics and Chemistry, as it is shown in several reviews and books1-8.
A spatially confined quantum system is defined as that in which its state functions satisfy certain boundary conditions for a finite value of the spatial coordinates1. The one-dimensional (1-D) harmonic oscillator limited by impenetrable walls is called 1-D confined harmonic oscillator (CHO).
The 1-D confined harmonic oscillator has been used as a model to study some more complicated systems, as for example: the proton-deuteron transformation to generate energy in dense stars9-10; in the theory of white dwarfs11, in the escape velocity of a star from a galaxy or a globular cumulus12; in the calculation of the specific heat of a crystal subjected to high external pressures14; in magnetic properties of metals15; in the study of color centers. More recently, the dynamics of a CHO subjected to a static electric field and a strong laser field has been studied39, it has called attention because it could be used to understand some aspects of the dynamics of ions caught in a Paul trap and in the study of the time of the revival of a particle in a CHO. Also few studies have been made on the transition probabilities and Einstein coefficients of the 1-D confined harmonic oscillator20,34,35 as a function of the box size, showing that new allowed transitions appear as a result of the confinement, this fact may be of technological interest.
Perhaps the first ones who studied the problem of the 1-D confined harmonic oscillator were Kothari andAuluck9-11. In the decade of the 40’s. They found that the eigen-functions of the system could be written in terms of Kummer’s functions. To obtain the values of the energy they needed to find the zeroes of the confluent hypergeometric function. They decided to carry out expansions and approaches to the hypergeometric function to obtain an analytical expression for the energy as a function of box size. They found the correct qualitative behavior; the energy of the levels of the CHO increases fast as the size of the box diminishes. Few years ago Baijal and Singh decided to get the zeroes of the hypergeometric function in a numerical way but their results were not accurate. Vawter improved the numerical results found by Baijal and Singh . At the beginning of 1980, Aguilera-Navarro et al. used the linear variational method to find, in a numerical way, the eigen-energies and eigen-functions of the CHO problem. They diagonalized the Hamiltonian matrix in the basis set of the free particle in a box of impenetrable walls. They obtained numerical values more accurate than those reported previously. However, the accuracy of their results is lower than the number of decimals that they reported.
The purpose of this work is to show the way in which the results of Aguilera-Navarro et al.30 can be improved.
The content of this work is as follows: In Sec. 2 we present the exact solution of the CHO problem. In Sec. 3 we use the linear variational method to obtain the energy eigenvalues. Finally, in section 4 we discuss our results and we give our conclusions.
2. The exact solution
The Schrödinger equation for the free 1-D harmonic oscillator (in natural units,
where the unit of the distance is
In order to find the solutions of Eq. (1) we make the following substitution
where
where
Now we make a change of variable, by defining
then the Eq. (1) is transformed to
This equation is identified as the Kummer differential equation. Its general solution, in terms of x, is given by:
where
The potential energy of Eq. (1) is a symmetric function of x, therefore the eigenstates of the Schrödinger equation have definite parity; odd or even.
In order that the wave functions do not diverge as
On the other hand, the exact solutions for the 1-D confined harmonic
oscillator are well known 91020212530 they
are obtained as follows. When the harmonic oscillator is symmetrically confined in a
box, of length
The eigen-energies are found as the successive roots of the following equations:
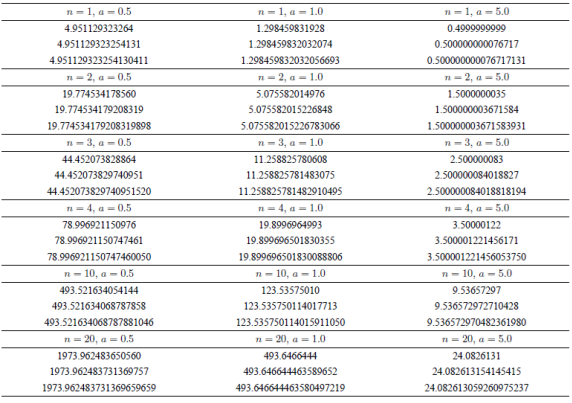
To determine the energy eigenvalues from these equations, it is necessary to solve numerically for one of the boundary conditions (4) according to the symmetry of the problem. The allowed energies can be determined with high accuracy by using some computer algebra system, Montgomery et al.16-17 used Maple but we can use Mathematica or a Fortran compiler with subroutines of extended precision. The numerical results obtained by solving the Eq. (5) with Mathematica 9 are reported in Table 1.
Table 1 Ground state energy for 1-D confined harmonic oscillator for few states n and different box sizes a. In the first row are the calculations of Aguilera-Navarro et al. 30. In the second row are the calculations of the present work, all calculations were carried out with N = 35 functions of the basis set. Finally, in the third row are the exact results obtained with the method described in the Sec. 2 and in the references16,17.
3. Linear variational approach
The Schrödinger equation independent of time is given by
We use the linear variational method to solve the eigenvalue Schrödinger equation. The wavefunction
where
The solution of eigen-value problem (Eq. 11) is equivalent to find the solutions of
where
are the elements of the Hamiltonian matrix.
It is convenient to write the CHO Hamiltonian in the following way:
Where
in which the potential is given by
The energy eigenvalues
And
The Hamiltonian matrix elements are:
in which
where
The Hamiltonian H (Eq. 15) is symmetric, therefore its eigen-functions have definite parity, even or odd. For even (odd) states, the expansion in (Eq. 12) includes only even (odd) states as given by Eq. (19).
The matrix elements
For even states they are:
Whereas for odd states we have
Where
4. Results and discussion
The diagonalization of the CHO Hamiltonian was already employed by Aguilera-Navarro, Ley-Koo and Zimerman30 in 1980, and subsequently used again by Taseli and Safer36 at the end of the 90’s. In Table I we show the calculations obtained in the present work with those obtained by Aguilera-Navarro et al.30 and with the exact ones16. Our calculations were made by using Mathematica 9 with real variables with 25 decimal places. For comparison we used the same number of basis set,
We compare our results with the exact ones obtained by Montgomery et al.16,17, and we find that the present calculations have the precision shown in Table 1.
In Table I we can see that the accuracy in the calculations of reference30 is lower than the results of the present study. The reason for this difference is due to the fact that in the early 80’s the diagonalization subroutines were not as efficient and accurate as they are today, and it could also be due to the fact that the calculations with double precision real variables could only handle 16 decimal places.
As we can see there is an improvement in the accuracy of the energy eigenvalues for boxes with











 text new page (beta)
text new page (beta)


