Introduction
The stress is generally developed in media due to natural phenomena. Since the earth is an elastic solid medium under high initial stresses. These stresses play a significant role to propagate an elastic wave. Chattopadhyay et al. (1978) discussed the propagation of Love type waves in an initially stressed crustal layer having an irregular interface. Chakraborty et al. (1981) shows that Love waves propagate in dissipative media under gravity. Chakraborty et al. (1983) discussed the effect of initial stress and irregularity on the propagation of SH-waves. Dey et al. (1996) discussed the propagation of Love waves in heterogeneous crust over a heterogeneous mantle. Abd-Alla et al. (1999) have studied the propagation of Love waves in a non-homogeneous orthotropic elastic layer under initial stress overlying semi-infinite medium. Dey et al. (2004) also studied the propagation of Love waves in an elastic layer with void pores. Sharma (2004) established a mathematical expression about wave propagation in a general anisotropic poroelastic medium with anisotropic permeability phase velocity and attenuation. Kalyani et al. (2008) have made finite-difference modeling of seismic wave propagation in monoclinic media.
Many researchers in the field of elastic wave propagation in layered medium bounded by different forms of irregular boundaries have been studied in several research papers. Such as Anjana and Samal (2010) shows that Love waves propagate in a fluid-saturated porous layer under a rigid boundary and lying over elastic half-space under gravity. Chattaraj and Samal (2013) investigated the propagation of Love waves in the fiber-reinforced layer over a gravitating porous half-space. Gupta et al. (2013a) discussed the propagation of Love waves in a non-homogeneous substratum over an initially stressed heterogeneous half-space. Gupta et al. (2013b) were introduced the possibility of Love wave propagation in a porous layer under the effect of linearly varying directional rigidities. SH-type waves dispersion in an isotropic medium sandwiched between an initially stressed orthotropic and heterogeneous semi-infinite media were studied by Kundu et al. (2013). Manna et al. (2013) show the Love wave propagation in a piezoelectric layer overlying in an inhomogeneous elastic half-space. Bacigalupo and Gambarotta (2014) discussed second-gradient homogenized model for wave propagation in heterogeneous periodic media. Propagation of Love wave in fiber-reinforced medium lying over an initially stressed orthotropic half-space was formulated by Kundu et al. (2014).
In this paper, we have studied the problem of propagation of Love waves in a porous layer over a heterogeneous elastic half-space under gravitating half-space for a rigid boundary as well as traction free boundary in the upper layer. Both the layer is considered under the effect of initial stress. The dispersion relations have been derived for rigid boundary as well as traction free boundary. The initial stresses play a vital role on the propagation of Love waves in the assumed medium. Gravity and heterogeneity in the half-space play a notable effect on the propagation of Love waves in the medium and half-space. The influences of porosity, initial stresses, and gravitational parameters have discussed graphically.
Formulation of the Problem
We consider a model consisting of the water-saturated anisotropic poroelastic layer under initial stress of finite thickness laying (Figure 1 under traction free boundary and Figure 2 under rigid boundary) over a gravitating heterogeneous elastic half-space under initial stress. Considering the origin of the coordinate system at the interface of the crust and mantle, z-axis downloads positively. The following variation has been taken.
For the half-space μ=μ2(1+a1z),ρ=ρ2
where, μandρ is the rigidity and density of the half space,a be the constant having inverse of length.
Solution of the Porous Layer
For the fluid-saturated anisotropic porous layer under initial stress P1 in the absence of body forces, the equation of motion can be written as (Biot, 1965).
where, s'ij are the components of stress tensor in the solid, S(=-fp) is the reduced pressure of the fluid, p is the pressure in the fluid, and f is the porosity of the porous layer, (u'x,v'y,w'z) are the components of the displacement vector of the solid and (Ux,Vy,Wz) are those of fluid. L and N are represent the shear moduli of the anisotropic layer in the x- and z- direction respectively, whereas A and F are elastic constants for the medium. The positive quantity Q is the measure of coupling between the changes in the volume of solid and liquid.
Since the Love waves propagating along the x-direction, having the displacement of particles along the y-direction, we have
This displacement will produce eyz and exy strain components and others are vanishing.
The dynamic components ρ11,,ρ12,ρ22 take into account the inertia effects of the moving fluid and are related to the densities of the solid ρs , fluid ρf and the layer ρ' by the equations
So that the mass density of the aggregate is
Also
The Love wave equation takes the form
Hence, we have from (2)
The shear wave velocity in the porous layer along the x-direction can be expressed as
whereㅤd=γ11-(γ212γ22),β1=√Nρ', is the velocity of shear wave in the corresponding initial stress free non-porous, anisotropic, elastic medium along x-direction.
ξ1=P12N , is the non-dimensional parameter due to the initial stress P1 and
γ11=ρ11ρ',γ12=ρ12ρ'andγ22=ρ22ρ', are the non-dimensional parameter for the material of the porous layer as obtained by Biot (1965)
We consider
where, k is the wave number and ω is the angular frequency.
Now, we get
The solution of equation (5)
where A andB are constants
Hence, finally we get
The Dynamic Equation of the Motion in the Half-Space
The dynamic equation of motion in the half space may be written as Biot (1965)
where, v2(x,z,t) is the displacement along y direction, ρ is the density, Sij are incremental stress and ωij are rotational components in the half space and g is the acceleration due to gravity. P2, is the initial stress.
The components of body forces are X=0,Y=0,Z=g.
The stress-strain relations are
In this problem ∂∂y=0 and μ=μ2(1+a1z),ρ=ρ2 , the stress strain relation becomes
where, μ is the modulus of rigidity, α1 is a variation parameter of a rigidity having dimension inverse of length.
Equation (8) using the above relations takes the form
The solution of equation (10) may be taken as
Equation (10) is now
where c22=μ2ρ2,ω=kc and G=ρ2gμ2k , is the Biot’s gravity parameter, ϕ″=∂2ϕ∂z2,ϕ'=∂ϕ∂z.
Now we substitute, φ(z)=ϕ(z){1+k(a1k-G2)z}12 in equation (12), we have
Using dimensionless quantities, η=-2[1+k(a1k-G2)z](a1k-G2)
Equation (13) becomes
which is standard Whittaker’s equation and solution is
where, DㅤandㅤE are arbitrary constants and WR,0(η) ,and W-R,0(-η) are the Whittaker functions.
As the solution should vanish at z→∞, i.e. for η→-∞, we may take the solution as
Hence
Boundary Conditions and Dispersion Relation
We consider two cases
Case A: If the surface of the upper layer is rigid boundary, then
Case B: If the surface of the upper layer is traction free, then
For case A using the boundary condition (i), we have
For case B using the boundary condition (i), we have
For case A and case B using the boundary condition (ii), we have
For case A and case B using the boundary condition (iii), we have
Case I -In rigid boundary, eliminating A, B and E from equation (17), (19) and (20), we have
Expanding the determinant, we get
Equation (22) gives the dispersion equation of Love wave in the anisotropic porous medium of finite thickness H under a rigid boundary and overlying elastic gravitating heterogeneous half-space under initial stress.
Particular Case
-
a. When, a1→0,ξ1=0andξ2=0, i.e. half-space becomes homogeneous under gravity, then equation (22) reduces to
b.
Which, is the result obtained by Anjana et al. (2010) for fluid-saturated porous layer under a rigid boundary and lying over an elastic half-space under gravity.
c. In case the medium is homogeneous i.e. f=0 then d=1,the equation (22) becomes
This is the dispersion equation of Love waves in medium under gravity.
d. When α=1i.eL=μ1, d→1,a1=0 andg→0, upper layer is non-porous and lower half-space is homogeneous without gravitational force the equation (22) becomes
e.
This is the dispersion equation of Love waves of finite thickness homogeneous elastic layer over semi-infinite in a homogeneous isotropic half-space bounded by a rigid boundary.
Case II -In traction free boundary, eliminating A, B and E from equation (18), (19) and (20), we have
Expanding the determinant, we get
Equation (28) gives the dispersion equation of Love wave in the anisotropic porous medium of finite thickness H under traction free boundary and overlying elastic gravitating heterogeneous half-space under initial stress.
Particular Case
When α=1 i.e. L→μ1,d→1,a1→0andG→0, upper layer is homogeneous and lower half-space is homogeneous without gravitational force the equation (27) becomes
This is the dispersion equation of Love waves in a homogeneous isotropic half-space in the absence of rigid layer.
Ange of Love Wave Speed
From the equation (22), it follows that Love waves can propagate in the porous layer under initial stress overlying heterogeneous elastic half-space under gravity if
Relation indicates the roll of initial stress and porosity of the media for the existence and non-existence of Love waves.
Numerical Results and Discussion
The dispersion curves of Love wave is drawn by taking d=0.6,cc2=0.87,Lμ2=2.5 and other data from Table 1 to Table 5.
Table 1 Parameters of Figure 1
| Rigid Boundary Curve No. | Traction free Boundary Curve No | ξ1 | G |
| 1 | 5 | 0.3 | 0.3 |
| 2 | 6 | 0.0 | 0.3 |
| 3 | 7 | 0.3 | 0.0 |
| 4 | 8 | 0.0 | 0.0 |
Table 2 Parameters of Figure 2
| Rigid Boundary Curve No. | Traction free Boundary Curve No. | ξ1 | G |
| 1 | 5 | 0.0 | 0.3 |
| 2 | 6 | -0.3 | 0.3 |
| 3 | 7 | 0.0 | 0.0 |
| 4 | 8 | -0.3 | 0.0 |
Table 3 Parameters of Figure 3
| Rigid Boundary Curve No. | Traction free Boundary Curve No. | G | a1k |
| 1 | 5 | 0.3 | 0.01 |
| 2 | 6 | 0.3 | 0.03 |
| 3 | 7 | 0.0 | 0.01 |
| 4 | 8 | 0.0 | 0.03 |
Table 4 Parameters of Figure 4
| Rigid Boundary Curve No. | Traction free Boundary Curve No. | ξ2 | G |
| 1 | 5 | 0.0 | 0.3 |
| 2 | 6 | -0.3 | 0.3 |
| 3 | 7 | 0.0 | 0.0 |
| 4 | 8 | -0.3 | 0.0 |
Table 5 Parameters of Figure 5
| Rigid Boundary Curve No. | Traction free Boundary Curve No. | ξ2 | G |
| 1 | 5 | 0.0 | 0.3 |
| 2 | 6 | 0.3 | 0.3 |
| 3 | 7 | 0.0 | 0.0 |
| 4 | 8 | 0.3 | 0.0 |
In Figure 1 and Figure 2, the phase velocity of the Love wave in absence of gravity is more than in the presence of gravity in the presence of compressive and tensile initial stress in the upper layer in case of rigid boundary. In case of traction free boundary, the phase velocity of the Love wave is same under the initial stress of the upper layer in the presence or absence of gravity.
The phase velocity of the Love waves in absence of gravity are more than in the presence of gravity without initial stress of the upper layer in rigid boundary, but in case of traction free boundary, the phase velocity of Love wave is same in the presence or absence of gravity without initial stress.
Also we observed that the phase velocity of Love waves in rigid boundary is more than traction free boundary, either upper layer is presence or absence of gravity or initial stress.
In Figure 3, the phase velocity of Love wave in absence of gravity is more than in presence of gravity in presence of heterogeneity parameter in case of rigid boundary where as in traction free boundary, the phase velocity of Love wave is same.
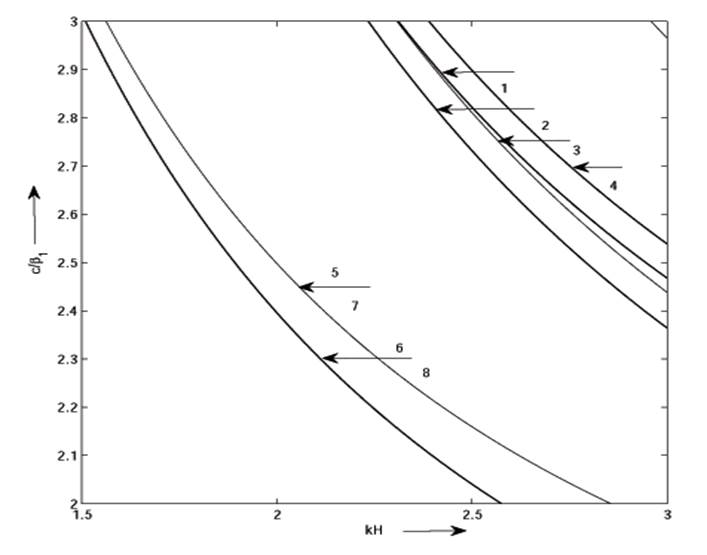
Figure 3 Variation of dimensionless phase velocity cβ1 against the wave number kH for the different values of initial stress ξ1.
If the heterogeneity parameter increases, the phase velocity of Love wave increases in presence or absence of gravity under rigid where as in traction free boundary the phase velocity decreases.
In Figure 4 and Figure 5, the phase velocity of Love wave in absence of gravity parameter is more than the presence of gravity parameter under compressive and tensile stress in half-space, under rigid boundary, whereas the phase velocity of Love wave is same in case of traction free boundary.
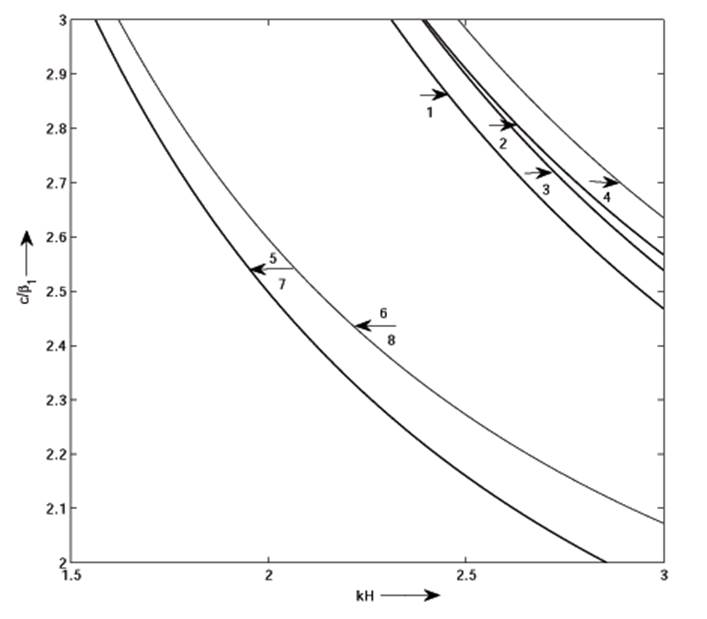
Figure 4 Variation of dimensionless phase velocity cβ1 against the wave number kH for the different values of heterogeneity parameter.
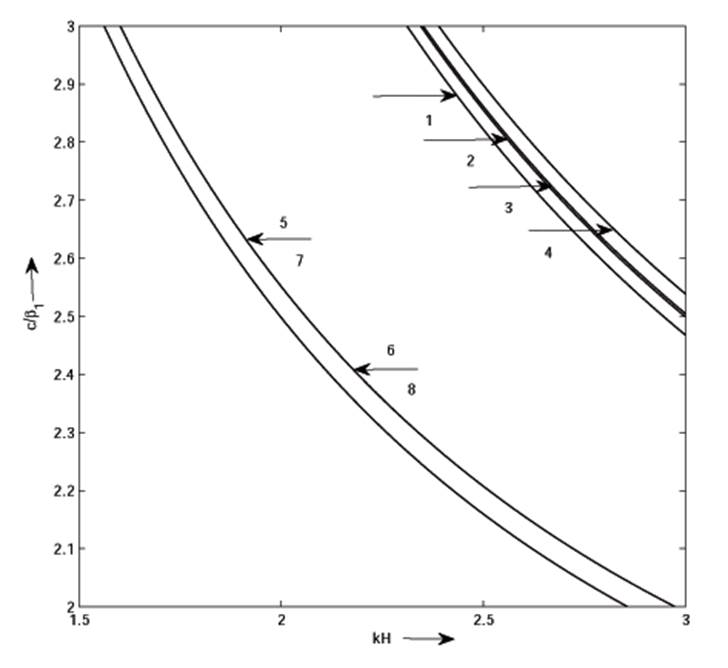
Figure 5: Variation of dimensionless phase velocity cβ1 against the wave number kH for the different values of heterogeneity parameter and G
The phase velocity of Love wave in absence of compressive and tensile stress in the half-space is more than the presence of gravity parameter under rigid boundary where as in traction free boundary, the phase velocity of Love wave is same. The phase velocity of Love wave in rigid boundary is always more than the traction free boundary in presence or absence of compressive and tensile stress in the half space.
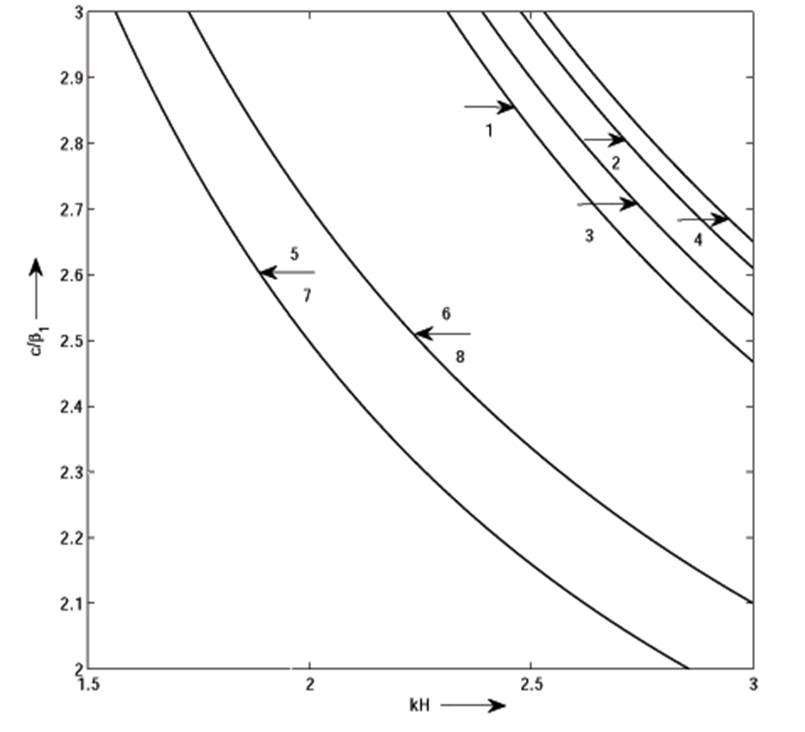
Figure 6 Variation of dimensionless phase velocity cβ1 against the wave number kH for the different values of compressive initial stress ξ2 and G.
Conclusions
Propagation of Love waves in porous layer under initially stress over heterogeneous half-space under gravity and initially stress has been investigated analytically in rigid boundary as well as traction free boundary. The dispersion equation is obtained in both the cases. From the figures we may conclude that
In absence or presence of initial stresses in the half-space or layer, the phase velocity of Love waves in rigid boundary is more than the traction free boundary in presence or absence of gravity.
In presence of initial stress in the half-space and absence of initial stress in the layer, the phase velocities of Love wave in traction free boundary is more than the rigid boundary.
Initial stresses play a vital role on the propagation of Love waves in the assumed medium.
Gravity and heterogeneity in the half-space play a notable effect on the propagation of Love waves in the medium and half-space.











 nueva página del texto (beta)
nueva página del texto (beta)





