PACS: 98.80Jk; 02.30.Gp
1.Introduccion
In recent years, increasing attention has been focused on the applications of fractional differential and integral operators to different branches of applied sciences and it has contributed a lot to mathematical physics and theoretical physics 1,2,3. In fact, the use of fractional calculus in many fundamental physical problems has attracted theorists to pay more attention to accessible fractional calculus tools that can be used in solving numerous problems of theoretical physics and quantum field theories 4-15. Recently, it was observed that fractional calculus plays an interesting role as well in cosmology and the physics of the early universe where some hidden properties are captured and analyzed in details (16,17,18 and references therein). In a more recent work, we have we have discussed the Friedman-Robertson-Walker (FRW) cosmology characterized by a scale factor obeying different independent types of fractional differential equations with solutions given in terms of and generalized Kilbas-Saigo-Mittag-Leffler functions 19. It was observed that this new fractional cosmological scenario exhibits some interesting results like the occurrence of an accelerated expansion of the universe dominated by the dark energy (DE) and the occurrence of a repulsive gravity in the early stage of the universe and time-decaying cosmological constant without the presence of scalar fields. In this paper, we would like to generalize this approach by introducing a new generalized fractional form of the scale factor in terms of the Hubble parameter and discuss its implications in the absence and in the presence of scalar fields. Our basic motivation to deal with a generalized fractional scale factor (GFSF) is based on the fact that the Hubble parameter which describes the expansion of the universe is considered one of the most important parameter in cosmology as it is used to estimate the age and the size of our universe. Considerable progress has been made in determining the Hubble constant and deducing its time-dependence since theories and observations predict that the Hubble parameter varies with time 20,21. Although there exist in literature a large number of phenomenological theories to describe the accelerated expansion of the universe 22-30, we consider that using fractional differential operators to describe cosmological scenarios is of considerable interest since fractional-order derivatives and integrals are nonlocal operators and hence one expects them to play important roles in cosmology 31,32. Therefore, one expects more hidden properties are present in cosmology with a GFSF that deserve to be captured and analyzed.
The paper is organized as follows: in Sec. 2, we introduce the main definitions and setups for the case of a FRW flat cosmology with a GFSF and a time-dependent Hubble parameter and we discuss some features in the absence of the scalar field whereas the presence of the scalar field will be discussed in Sec. 3; we consider the case of a cosmology with a Gauss-Bonnet gravity for reasons that will be mentioned in the same section; finally conclusions and perspectives are given in Sec. 4.
2. FRW cosmology with a generalized fractional scale factor
By using the following FRW metric for a flat universe
where
is the Mittag-Leffler function 38.
2.1
We choose at the beginning
we can write the solution (1) as:
We can set for simplicity
One particular choice of parameter is obtained if for instance we set
and therefore the scale factor is increasing with time and a universe dominated by such a form of a scale factor is accelerated with time and is non-singular. In conclusion, Eq. (5) is the solution of the fractional differential equation
In our model the Einstein’s field equations that govern our model of consideration are
and
51, the cosmological constant varies as:
Accordingly the Friedmann equation gives:
The differential equation
with
For
For
2.2
We can choose
then from Eq. (1) the scale factor evolves as:
For λ = 1 -α, Eq. (12) is reduced to:
we can write:
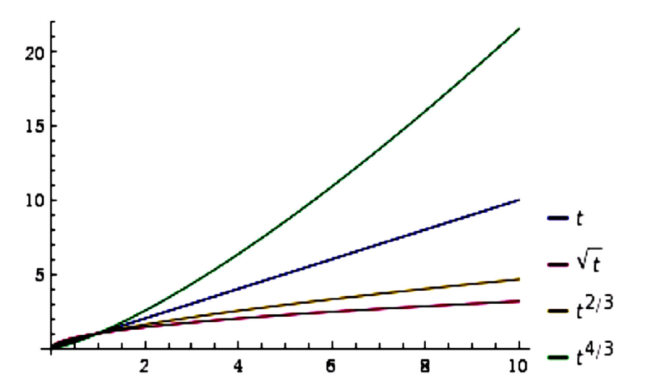
The scale factor in that case increases faster than in the previous case and the universe is non-singular. To illustrate graphically, we plot in Fig. 5 the variations of the scale factor for both cases

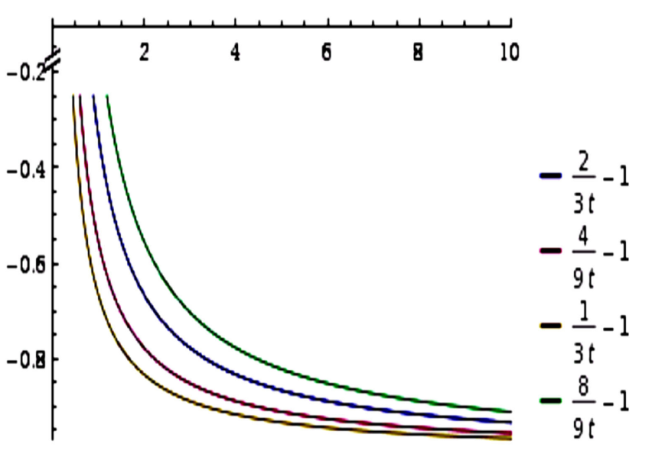
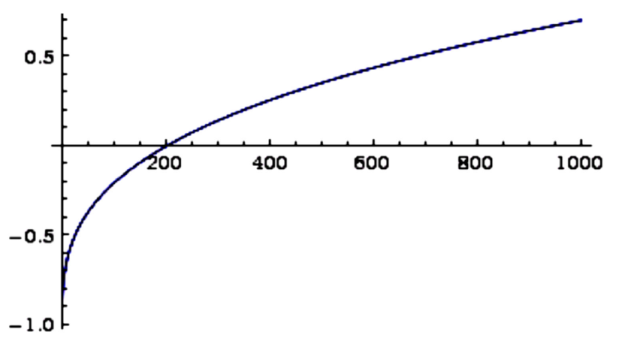
Figure 5 Variations of α(t) for
For
whereas for
we find
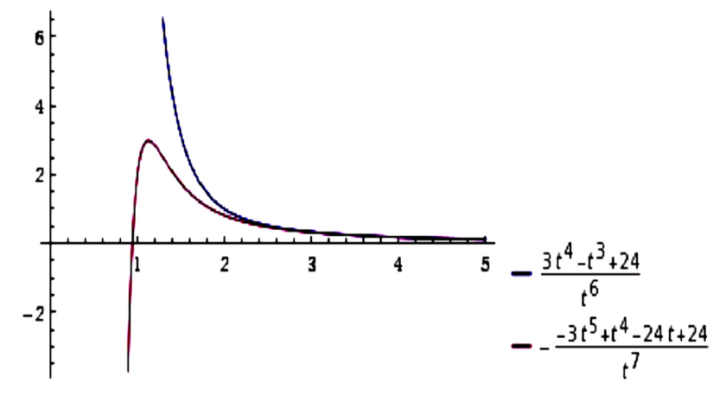
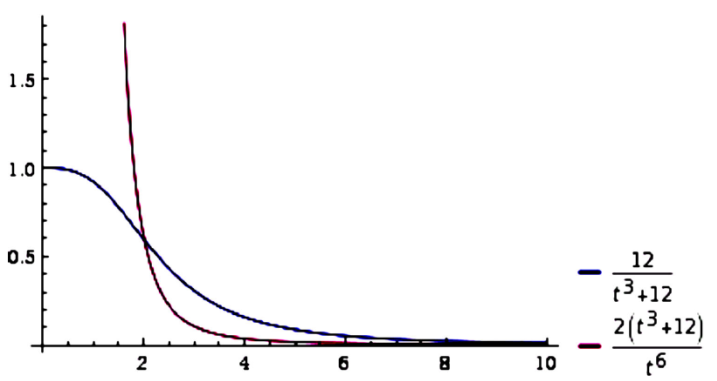
Figure 6 Variations of Λ(t) for
It is noteworthy that for α = 1 and for σ = 0, i.e. absence of F(t) , the fractional differential equation
3. FRW cosmology with a generalized fractional scale factor and with a scalar field
Despite that the OULFDE offers the standard FRW model many desirable features in the absence of scalar fields it will be of interest to know the effects of OULFDE on the FRW model with single scalar field. It is believed that scalar fields can account for both an accelerated exponentially expansion of the early universe as well for the late-time accelerated expansion of the universe 54,55. However, there are recent approaches which suggest that a non-minimally coupled field to the scalar curvature can generate dark energy and even dark matter 56. It is notable that the coupling of the scalar field to curvature appears in alternative theories of gravities which could be responsible after compactification of higher dimensions for the current accelerated expansion. Some nice alternatives theories include the string-inspired dilaton gravities, Kaluza-Klein theory, M/string theory and higher derivative theories with correction terms of higher-orders in the curvature 57,58. These correction terms may play a significant role in the inflationary epoch. The leading quadratic correction terms correspond to the Gauss-Bonnet (GB) curvature invariant which appears in the tree-level effective action of the heterotic string 59,60. In four-dimension, the GB term is a topological invariant, ghost-free in Minkowski background, its appearance alone in the action can be neglected as a total divergence. However, if coupled, it affects the cosmological dynamics in presence of dynamically evolving dilaton and modulus fields at the one-loop level of string effective action and may have desirable features in many cosmological scenarios, e.g. avoiding the initial singularity of the universe 61. Many of inflationary potentials are disfavored when constrained to Planck CMB data due mainly to the large tensor-to-scalar ratio yet it was argued in 62 that non-minimal coupling to the GB term may set these potentials in good agreement with the Planck data. There are many research papers discussing the scalar field cosmology with GB curvature corrections as possible solutions to DE with a field-dependent scalar potential 63-66. In this section, we investigate a cosmological FRW model in which a single dynamical scalar field is minimally coupled to gravity in the presence of the GB invariant and a scale factor governed by the OULFDE. However, in this section we assume that G is constant and we set
where
By varying the action over the scalar field, we obtain:
In addition, the continuity equation for the present model
The dynamics depends accordingly on the value of the fractional parameter α. We discuss the following two independent cases:
3.1
For α = 1/2, the following 2nd-order linear differential equation for the scalar field
We plot in Fig. 7 the variations of the energy density with time for ɛ = 1 and ɛ = 2 assuming
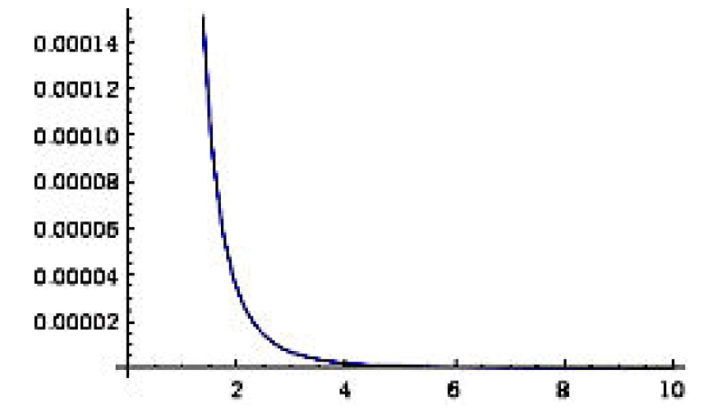
Figure 7 shows that for ɛ = 2 the energy density was negative at the early stage of the evolution. A negative energy density in the early universe was discussed recently in70 and has many physical impacts on inflationary epoch. For ɛ = 1 the continuity equation
For ɛ = 2, we find
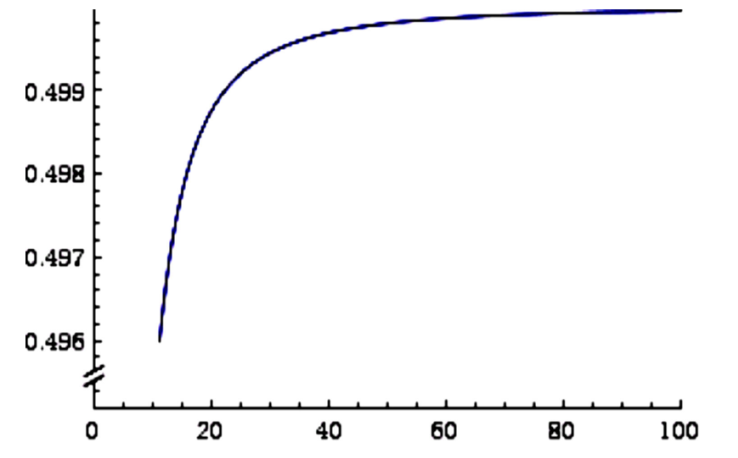
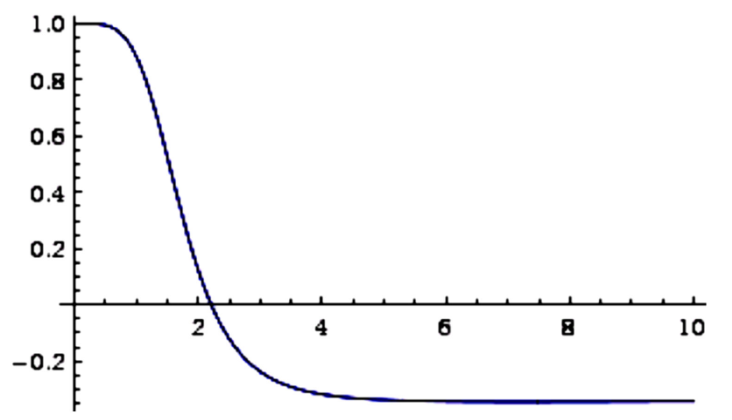
Their variations with time are plotted in Figs. 9 and 10.
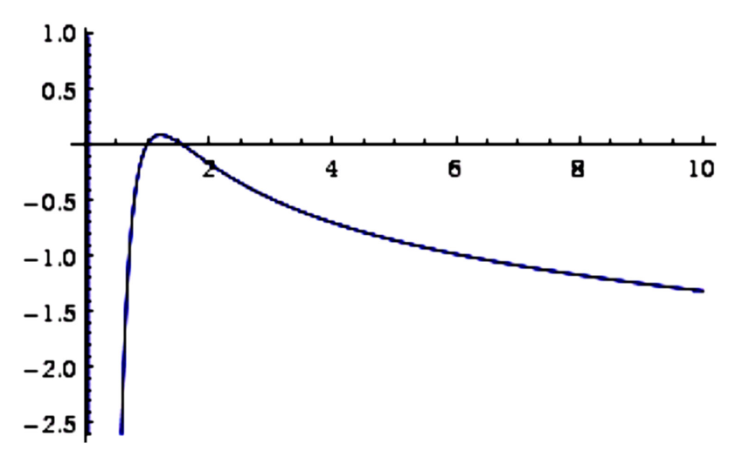
For ɛ = 1 the EoS parameter decreases with time and tends toward a stable value close to -0.335 which is within the range of observational limits 71,72 whereas for ɛ = 2, the EoS parameter decreases from a larger positive value toward a negative stable value close as well to -0.335. This case shows that the early universe is not necessarily dominated by a negative pressure matter with a positive energy density. The behavior of the EoS parameter shows that for ɛ = 1 and ɛ = 2 the phantom-divide line is not crossed in our scenario.
3.2
For α = 1/4 and ɛ = 1, the following 2nd-order linear differential equation for the scalar field
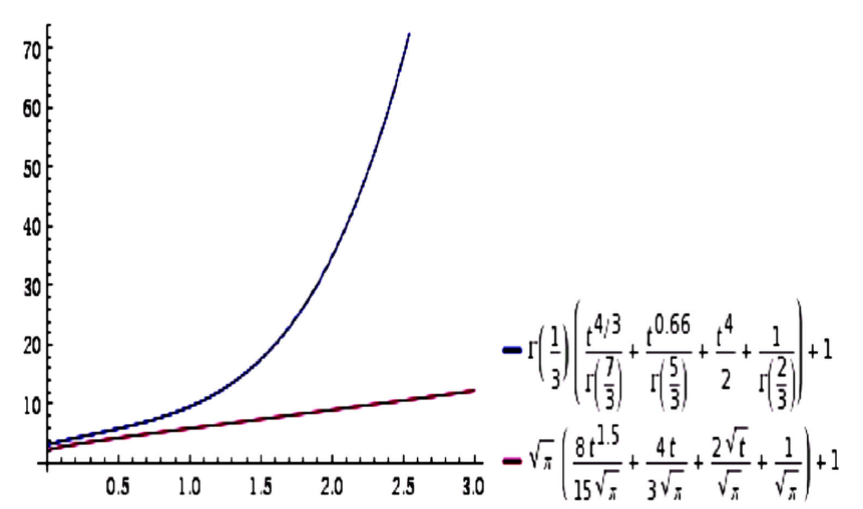
and the solution is given by:
where
Accordingly, the energy density varies as:
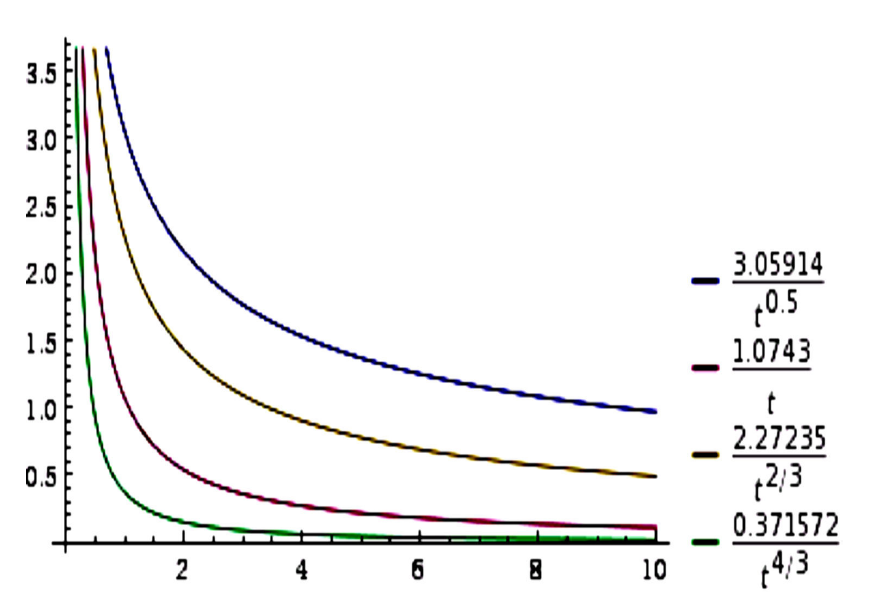
Accordingly, the EoS parameter varies asymptotically as
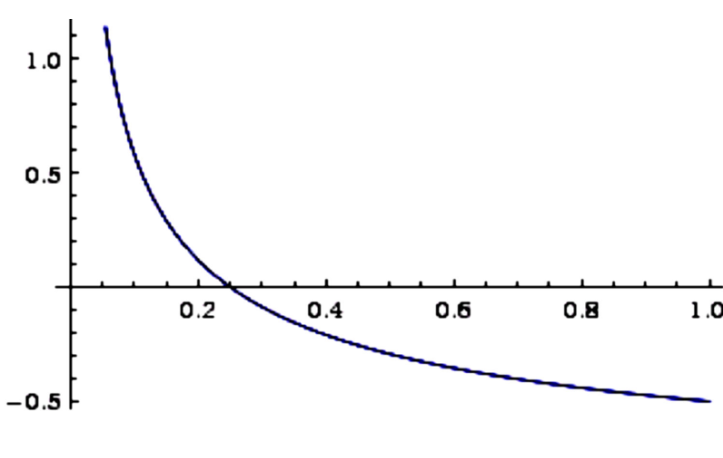
We plot in Figs. 11-13 respectively the variations of the scalar field, the energy density and the EoS parameter with time:
In that case the EoS parameter tends asymptotically toward ω = -1 at the present epoch. The universe in non-singular, expanding acceleratedly with time and is dominated by a cosmological constant at very late time. The energy density was negative in the past, increases toward a positive value then decreases toward zero with time. The expansion of the early universe is due to the negative energy density with the negative pressure and the late-time accelerated expansion comes from the positive energy and the negative pressure which behave like a dark energy. It is amazing that the non-singular universe is expanding with time whereas a transition from a negative energy density to a positive energy density occurs during its dynamical evolution. Similar scenario occurs in 73 and negative energies density in accelerated universe was discussed recently in 74.
Let us at the end mention that for the case of a time-dependent gravitational constant and cosmological constant with
and accordingly, we find after simple algebra assuming
Assuming
Accordingly, the EoS parameter decays as
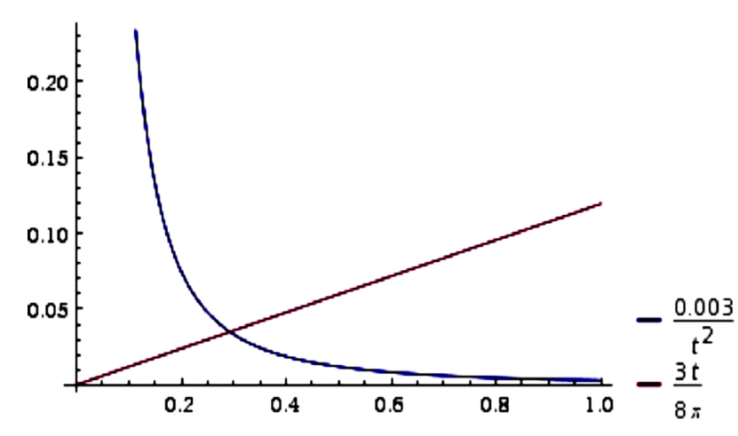
The present time relative variation of the linearly increasing gravitational constant is
For α = 2/3 and ɛ = 1, we find
and the solution is given by:
where c5 and c6 are constants of integration;
Asymptotically, at
Assuming
We left other values of for interested readers.
It is notable that for α = 1 and σ = 0, the scale factor
The dynamics therefore differs from previous solutions obtained for
which differs completely from previous solutions obtained. c7,…,c9 are constants of integration The solution depends on initial conditions and is constrained by
4. Conclusions and Perspectives
In this paper, we have tried to show that fractional calculus plays an important role in modern cosmology and a number of properties were hidden and deserve to be captured. Through this paper, we have considered a GFSF which obeys the OULFDE with a particular Hubble parameter of the form
In the absence of the scalar field and for the case of a flat FRW model with time-dependent gravitational constant and a time-dependent cosmological constant varying as
In the presence of the scalar field and higher-order GB curvature term, the dynamical equations offer some new features. For the case of a constant and Λ, the dynamics depend on the form of the GB coupling function
These features prove that fractional calculus deserves to be considered seriously in cosmology and physics of the early universe. Both the GFSF and the OULFDE offers new insights in modern cosmology and it will be of interest to explore in details in the future their impacts on the dark energy problem and the quantum physics of the universe. It will be interesting to explore in the upcoming work whether the present fractional model may be tested by future observational data fittings.











 nueva página del texto (beta)
nueva página del texto (beta)