1. Introduction
Fabrication of artificial multiferroic thin film structures, in which ferromagnetic (FM) and ferroelectric (FE) compounds are artificially assembled is valuable, because these structures are promising candidates in exploration of robust cross-coupling effects at room temperature by exploiting the elastic coupling between the two constituents, leading to extrinsic magnetoelectric (ME) effects or other phenomena such as the sheet resistance modulation (Mott transition) in a ferroelectric field-effect transistor (FE-FET) structure, which is comparatively easy to produce as it does not require large defect-free ferroelectric layers1. Furthermore, some manganites used like electrodes are known to drastically decrease fatigue on capacitive structures based on Pb(Zr,Ti)O 3 -PZT, making them natural candidates for robust FE-FETs1. Among the most attractive materials for practical implementation of multiferroic heterostructures and fundamental studies of magnetoelectric coupling are the lanthanum doped manganites, La 1−𝑥 A 𝑥 MnO 3 , (A=Ca, Sr, or Ba) for FM materials2, while that for FE material are BiFeO 3 , BaTiO 3 and PZT3-8. At ferromagnetic/insulator interfaces, the ME effect may originate from purely electronic mechanisms. It was predicted that displacements of atoms at the FM/FE interface caused by ferroelectric instability alter the overlap between atomic orbitals at the interface, which affects the interface magnetization9. This produces a ME effect that manifests itself in the abrupt change in the interface magnetization caused by ferroelectric switching under influence of applied electric field10. Additionally, the stacking of layers of different ferroelectrics in a multilayer composite configuration has proven successful for the improvement of the dielectric and ferroelectric properties of single phase films. Some examples are the solid solution (1−𝑥) (Bi 0.5 Na 0.5 ) TiO (3−𝑥) BaTiO 3 (BNBT) has been presented as a very promising candidate11,12. It shows large piezoelectric coefficients for compositions in the vicinity of a MPB. Recently, it has been reported relaxor-ferroelectric character in the operties of this solid solution for compositions close to the MPB13. Additionally, (Bi 0.5 Na 0.5 ) 1−𝑥 Ba 𝑥 TiO 3 (BNBT) solid solution from solution precursors with and without Na + and Bi 3+ excesses prepared from solution precursors with different concentrations (𝑥=0.035, 0.055, 0.080, 0.100 and 0.150) can be used as a ferroelectric material in future research because of its saturation polarization values and low coercive fields14. In vertically aligned nanostructures, there is no such constraint and the ME effect is expected to be stronger. Such a behavior was demonstrated for ferrimagnetic CoFe 2 O 4 nanopillars embedded in an FE BaTiO 3 matrix. Temperature-dependent magnetic measurements demonstrated the coupling between the two-order parameters, which was manifested as a change in magnetization at the FE Curie temperature15.
Lanthanum doped manganites are intimately related to the coexistence of competing nearly degenerate states which couple simultaneously active degrees of freedom-charge, lattice, orbital, and spin states 16,17. La 0.67 Sr 0.33 MnO 3 (LSMO) is considered as a compound with a higher metal-insulator transition temperature and is ferromagnetic at a Curie temperature of 370 K18. The electrical and magnetic behavior is largely determined by the electrons in the 3d shell of the manganese atoms19. A lot of studies have been focused on BaTiO 3 (BTO) thin films as FE layers because of their remarkable ferroelectric, electrical, optical, nonlinear optical and dielectric properties. Also in their paraelectric and ferroelectric phases offer great promise as capacitor dielectrics for high-density random access memory (RAM) applications. Theoretically, the critical thickness for BTO films has been determined to range from 12 nm to less than 1 nm20,21. On BaTiO 3 hybridization of d orbitals with occupied p orbitals of the octahedrally coordinated oxygen ions can induce long range polarization22. Through its low-temperature structural changes, ferroelectric BTO offers an opportunity to study strain modifications of magnetic and magnetotransport properties3,4,6,23. In this work, we investigated the effect of the presence of BaTiO 3 layer with different thicknesses (t BTO ) on structural, electrical and magnetic properties of La 2/3 Sr 1/3 MnO 3 /BaTiO 3 bilayers grown on (001) SrTiO 3 substrates by sputtering at high pure oxygen pressures. X-ray diffraction allows to study structural properties, showing a strained LSMO layer and a relaxed behavior on BTO layer. Electrical measurements show a metal behavior at room temperature and magnetic properties like saturation magnetization and coercive field exhibit dependence with BTO thickness.
2. Experimental details
LSMO/BTO bilayers were deposited on (001) single-crystal STO by RF and DC sputtering, respectively, at pure O 2 pressure around of 3.5 mBar and a substrate temperature of 830 ∘ C. A power density of 7 W/cm 2 and 12 W/cm 2 were used for LSMO and BTO targets, respectively. In our studies, LSMO thickness was chosen around 40 nm and BTO thickness varied in the range from 20 to 100 nm. The thicknesses of the individual layers were obtained by X-ray reflectivity measurements in the bilayers, not shown. X-ray diffraction
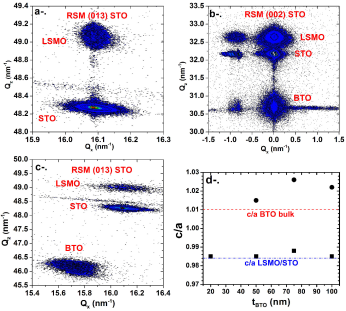
Figure 1 a-. Asymmetric (013) RSM for LSMO(40 nm)/STO thin film. b-. Symmetric (002) RSM for LSMO(40 nm)/BTO(20 nm) bilayer. c-. Asymmetric (013) RSM for LSMO(40 nm)/BTO(20 nm) bilayer. d-. c/a ratio dependence with tBTO for all bilayers
and Reciprocal Space Maps (RMS) measurements show highly textured layers with preferential growth in the c-axis direction. Resistivity as a function of temperature was measured using indium contacts on the surface and aluminum wires (0.127 mm of diameter) with in a current-inplane (CIP) geometry. The magnetic loops, and thermal demagnetization measurements were carried out in a MPMS Quantum Design ® system operated by a SQUID detector in the 10-400 K temperature range.
3. Results and analysis
3.1 Structural properties
In order to determine the degree of relaxation and strain in the bilayers, reciprocal space mapping (RSM) of the bilayers was performed. In Fig. 1a-. (013) asymmetric RSM of LSMO(40 nm)/STO a LSMO film strained is observed, with a lattice parameter c=3.843 Å. For LSMO(40 nm)/BTO(20 nm) bilayer a (002) symmetric RSM show the three materials on Fig. 1b-. Additionally, on (013) asymmetric RSM of LSMO(40 nm)/BTO(100 nm), shown in Fig. 1c, we found a LSMO layer strained due to that on all RSM, the (013) peak of film is aligned with STO peak. Instead of BTO layers is relaxed as is characteristic on all bilayers (in RSM BTO peak is shifted to the left of STO) with a c/a 𝐿𝑆𝑀𝑂 =0.985 and c/a BTO =1.022. An increase of c/a ratio with t 𝐵𝑇𝑂 due to BTO relaxation is observed on Fig. 1d-.
3.2 CIP transport properties
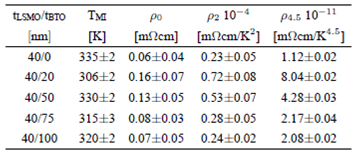
In Fig. 2 the dependence of resistivity with temperature measured in CIP geometry is shown, for LSMO/BTO with t 𝐿𝑆𝑀𝑂 =40 nm and varying the thickness of the BTO layer, t 𝐵𝑇𝑂 , from 0 to 100 nm. All bilayers show a metal insulator transition characteristic of the LSMO system above 350 K (not shown). In this system was not observed a weak discontinuity on resistivity, attributed to structural transitions of BTO5, which was found on LCMO/BTO bilayers due to strain on the interfaces on previous work5. The metal insulator transition temperature (T 𝑀𝐼) is slightly dependent of BTO thickness. A decrease on residual resistivity is observed with increase of t BTO , with values near to LSMO/STO thin film 60 𝜇𝛺cm. The values obtained for the fits are consigned on Table 1. At low temperature, the resistivity in zero field was fitted using 𝜌(𝑇)= 𝜌 0 + 𝜌 2 𝑇 2 + 𝜌 4.5 𝑇 4.5 , where 𝜌 0 is the residual resistivity, 𝜌 2 is related to electron-electron scattering24,25 and 𝜌 4.5 corresponds to the magnon-magnon scattering26,27.
3.3 Magnetic properties
Figure 3 shows ZFC thermal demagnetization, from 10 to 350 K, for LSMO(40 nm)/BTO (t BTO ) bilayers, with BTO thickness: 0 nm (diamond), 20 nm (pentagon), 50 nm (triangle), 75 nm (circle) and 100 nm (square). A magnetic field
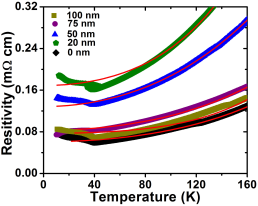
Figure 2 Temperature dependence of the resistivity for LCMO/BTO bilayers varying tBTO: 0 nm (diamond), 20 nm (pentagon), 50 nm (triangle), 75 nm (circle) and 100 nm (square).
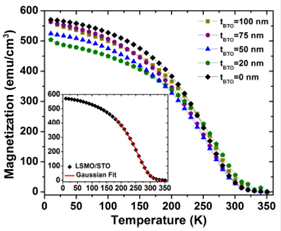
Figure 3 Temperature dependence of magnetization at ZFC (Happ = 5 kOe for all bilayers), for LSMO(40 nm)/BTO (tBTO)/STO bilayers, with tBTO = 0 nm (diamond), 20 nm (open square), 50 nm (triangle), 75 nm (circle) and 100 nm (square). Inset: Magnetization for LSMO/STO (diamond) with Gaussian fit28.
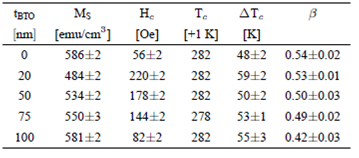
(H app ) of 5 kOe was applied parallel to the plane of the film to measure magnetization. By fitting experimental data to a Gaussian distribution of long-range conducting ferromagnetic regions (inset Fig. 3), which has been used in LSMO thin films28, we extracted Curie temperature, T C , and width of the ferromagnetic/paramagnetic transition temperature 𝛥T C (Table 2). Saturation magnetization, coercive field, Curie temperature and 𝛥T C values obtained for an LSMO/STO film and the bilayers grown, are given for comparison. Thermal demagnetization curves display a typical behavior of LSMO thin film. However, LSMO(40 nm) /BTO(50 nm) bilayer (triangle), display the lower Ms∼500 emu/cm 3 at 10 K, which is a reduction of 17% on Ms in comparison with LSMO/STO thin film. For other bilayers the reduction on Ms is lower than 10% due to BTO layer influence. In these samples no magnetic moment is blocked or frozen like on previous studies on BTO/LCMO bilayers6. The presence of the BTO layer affects the magnetic moment of the ferromagnetic layer at the interface and this interaction depends on the thickness of the BTO layer. Probably, a magnetic contribution exists of the BTO induced by interfacial interactions at the interface, but also induced by crystalline strains originated during the growth process. Critical exponent 𝛽 obtained from fit, identifies a magnetic behavior associated to theoretical model of mean field approximation where 𝛽=0.529 and was observed on LSMO30. In our bilayers, the BTO presence affects M 𝑠 and H 𝑐 of the LSMO layer. T 𝐶 and critical exponents are approximately constant for all bilayers, this may be explained by BTO relaxation observed on RMS. The strain at the interface is not enough for changing the LSMO magnetic mechanism. Additionally, 𝛥T C show that the homogeneity is the same for all bilayers.
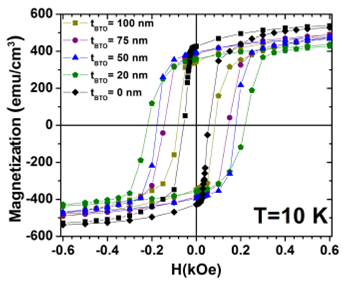
Figure 4 shows isothermal magnetic hysteresis loops at 10 K, from -0.6 to +0.6 kOe, for the LSMO(40 nm)/ BTO(t BTO ) bilayers, with BTO thickness. The bilayer exhibit saturation magnetization at magnetic field of 1 kOe. Saturation magnetization in bilayers is lower than that for an LSMO/STO film (590 emu/cm 3 ) grown under identical parameters. Interesting enough, when t BTO reaches 100 nm thick, Ms and Hc (56 Oe) have approximately the same value for a 40 nm thick LSMO film. Figure 5 shows saturation magnetization a-. and coercive field b.- as function of BTO

Figure 5 BTO layer thickness (tBTO) a-. Dependence of saturation magnetization (Circle) and b-. Coercive field (squares). Lines are guides for the eye.
layer thickness, t BTO (nm), where Ms and Hc exhibits a linear behavior. For t BTO smaller than 100 nm Ms is reduced whereas coercive field is bigger than those from the expected value for a film. This reduction of the saturation magnetization and increase of the coercive field in the bilayers could
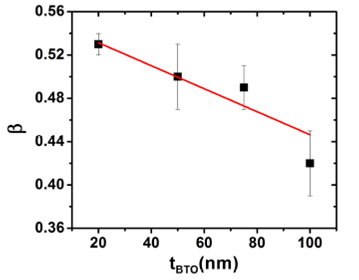
could be attributed to both spin canting and phase separation at the interface, and strain-induced magnetic disorder in the film31,32, as we have observed in previous studies on LCMO/BTO and BTO/LCMO [3,4-6]. We observed a dependence of coercive field with t BTO . Figure 6 displays de critical exponent dependence with t BTO , for bilayers. An apparent change on magnetic mechanism is observed from mean field to near regions of Heisenberg 3d33-35. This probably is due to dead layer for low thickness36,37, and strain on LCMO distorted the MnO octahedral. This dependences confirms the influence of BTO in the magnetic properties of the manganite (for example mismatch LSMO/BTO). In our bilayers magnetic properties of the system are affected by the presence of the ferroelectric layer. Oxygen vacancy can strongly change the correlation effects especially in transition metal oxides. The oxygen vacancies can change the electronic structure; doping with electron/hole; variation in valence of cation; induce local strain; change local electron density; changing the magnetic, dielectric and transport properties of complex metal oxides, also. Additionally, the polarization direction and dislocations on BTO for regions near to interface, can alter the crystalline field and modify the direction of magnetization (from in-plane to out-plane) or cause effects like spin glass, dead layer that alter the magnetism mechanism.
4. Conclusions
We studied structural, magnetic and transport properties in ferroelectric/ferromagnetic bilayers of BTO and LSMO oxides grown via DC and RF sputtering in pure oxygen atmosphere, systematically varying the BTO layer thickness and maintaining constant LSMO layer thickness. Bilayers show that LSMO layer is strained on all samples and BTO is always relaxed, c/a ratio (0.986) for LSMO remain approximately constant, while for BTO c/a >1, indicating ferroelectric behavior in this kind of bilayer. Electrical measurements shows that all samples satisfy the electron-electron scattering and magnon-magnon scattering model, so the BTO layer does not affect the electrical transport mechanism in LSMO. The saturation magnetization and coercive field show dependence on BTO thickness, which may be due to relaxation effects of the BTO film for thicker layers.











 nueva página del texto (beta)
nueva página del texto (beta)